A condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor when the potential difference across the condenser reduces to V2 is
(C(V1−V2)2L)12
C(V21−V22)L
C(V21+V22)L
(C(V21−V22)L)12
The correct Answer is:D
To solve the problem step by step, we can follow these steps:
Step 1: Understand the Initial Conditions
A capacitor with capacitance C is charged to a potential difference V1. The initial charge Q0 on the capacitor can be calculated using the formula:
Q0=C⋅V1
Step 2: Connect the Capacitor to the Inductor
When the capacitor is connected to an ideal inductor of inductance L, it begins to discharge, creating an oscillatory current in the circuit.
Step 3: Define the Charge at Any Time
The charge Q on the capacitor at any time t during the discharge can be expressed as:
Q=Q0cos(ωt)
where ω is the angular frequency of the oscillation, given by:
ω=1√LC
Step 4: Relate the Charge to the Potential Difference
Initially, the potential difference across the capacitor is V1, and it reduces to V2. The relationship between charge and potential difference is given by:
Q=C⋅V
Thus, when the potential difference is V2, we have:
Q=C⋅V2
Step 5: Set Up the Equation
From the expression for Q:
C⋅V2=Q0cos(ωt)
Substituting Q0=C⋅V1:
C⋅V2=C⋅V1cos(ωt)
Dividing both sides by C (assuming C≠0):
V2=V1cos(ωt)
Thus, we can express cos(ωt) as:
cos(ωt)=V2V1
Step 6: Find the Current Through the Inductor
The current I through the inductor is given by:
I=−dQdt
Calculating dQdt:
I=−ddt(Q0cos(ωt))=−Q0(−ωsin(ωt))=Q0ωsin(ωt)
Step 7: Substitute Values
Substituting Q0=C⋅V1 and ω=1√LC:
I=C⋅V1⋅1√LCsin(ωt)
Step 8: Express sin(ωt)
Using the identity sin2(ωt)+cos2(ωt)=1:
sin2(ωt)=1−cos2(ωt)=1−(V2V1)2
Thus, sin(ωt)=√1−(V2V1)2.
Step 9: Final Expression for Current
Substituting sin(ωt) back into the equation for current:
I=C⋅V1⋅1√LC√1−(V2V1)2
This simplifies to:
I=C⋅V1√LC
⎷1−V22V21
Finally, this can be expressed as:
I=C(V21−V22)L
Final Answer
The current through the inductor when the potential difference across the capacitor reduces to V2 is:
I=C(V21−V22)L
A capacitor with capacitance C is charged to a potential difference V1. The initial charge Q0 on the capacitor can be calculated using the formula:
Q0=C⋅V1
When the capacitor is connected to an ideal inductor of inductance L, it begins to discharge, creating an oscillatory current in the circuit.
The charge Q on the capacitor at any time t during the discharge can be expressed as:
Q=Q0cos(ωt)
where ω is the angular frequency of the oscillation, given by:
ω=1√LC
Initially, the potential difference across the capacitor is V1, and it reduces to V2. The relationship between charge and potential difference is given by:
Q=C⋅V
Thus, when the potential difference is V2, we have:
Q=C⋅V2
From the expression for Q:
C⋅V2=Q0cos(ωt)
Substituting Q0=C⋅V1:
C⋅V2=C⋅V1cos(ωt)
Dividing both sides by C (assuming C≠0):
V2=V1cos(ωt)
Thus, we can express cos(ωt) as:
cos(ωt)=V2V1
The current I through the inductor is given by:
I=−dQdt
Calculating dQdt:
I=−ddt(Q0cos(ωt))=−Q0(−ωsin(ωt))=Q0ωsin(ωt)
Substituting Q0=C⋅V1 and ω=1√LC:
I=C⋅V1⋅1√LCsin(ωt)
Using the identity sin2(ωt)+cos2(ωt)=1:
sin2(ωt)=1−cos2(ωt)=1−(V2V1)2
Thus, sin(ωt)=√1−(V2V1)2.
Substituting sin(ωt) back into the equation for current:
I=C⋅V1⋅1√LC√1−(V2V1)2
This simplifies to:
I=C⋅V1√LC
⎷1−V22V21
Finally, this can be expressed as:
I=C(V21−V22)L
The current through the inductor when the potential difference across the capacitor reduces to V2 is:
I=C(V21−V22)L
Topper's Solved these Questions
Similar Questions
A condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor wehnn the potential difference across the condenser reduces to V2 is
View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionA capacitor of capacitnace C is charged to a potential difference V and then disconnected from the battery. Now it is connected to an inductor of inductance L at t=0. Then
 View Solution
View SolutionA capacitor of capacity 2μF is changed to a potential different of 12V . It is then connected across an inductor of inductance 0.6mH What is the current in the circuit at a time when the potential difference across the capacitor is 6.0V ?
View SolutionA capacitor of capacity 2μF is changed to a potential different of 12V . It is then connected across an inductor of inductance 0.6mH What is the current in the circuit at a time when the potential difference across the capacitor is 6.0V ?
View SolutionA capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 6μH. At an instant when potential difference across the capacitor is 6V, what is the current(in A)?
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View SolutionIn the circuit shown, rms circuit is 11 A . The potential difference across the inductor is
 View Solution
View SolutionTwo capacitors of capacitance C and 3C are charged to potential difference V0 and 2V0, respectively, and connected to an inductor of inductance L as shows in Fig. Initially, the current in the inductor is zero. Now, switch S is closed.
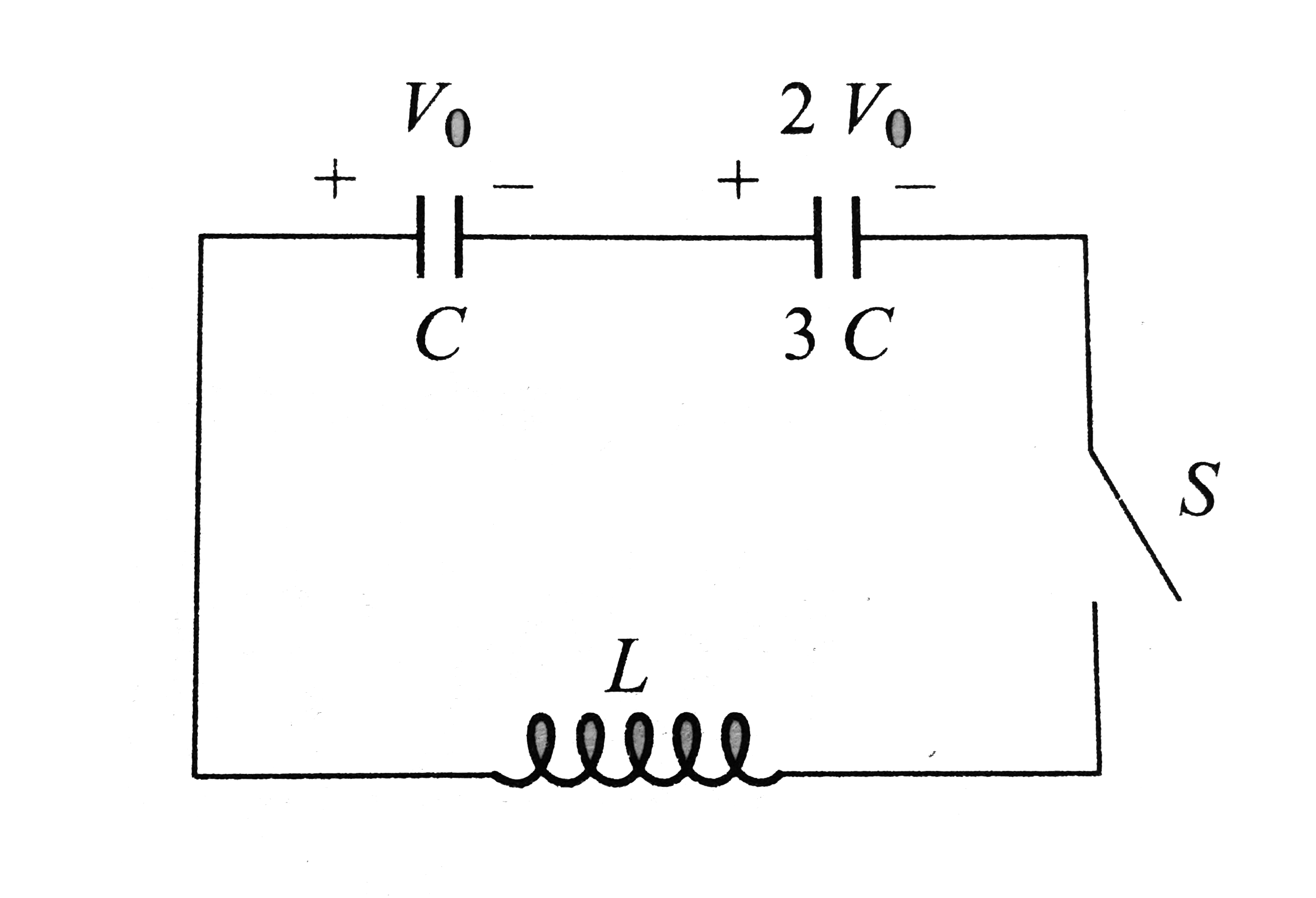
Potential difference across capacitor of capacitance C when the current in the circuit is maximum isView SolutionTwo capacitors of capacitance C and 3C are charged to potential difference V0 and 2V0, respectively, and connected to an inductor of inductance L as shows in Fig. Initially, the current in the inductor is zero. Now, switch S is closed.
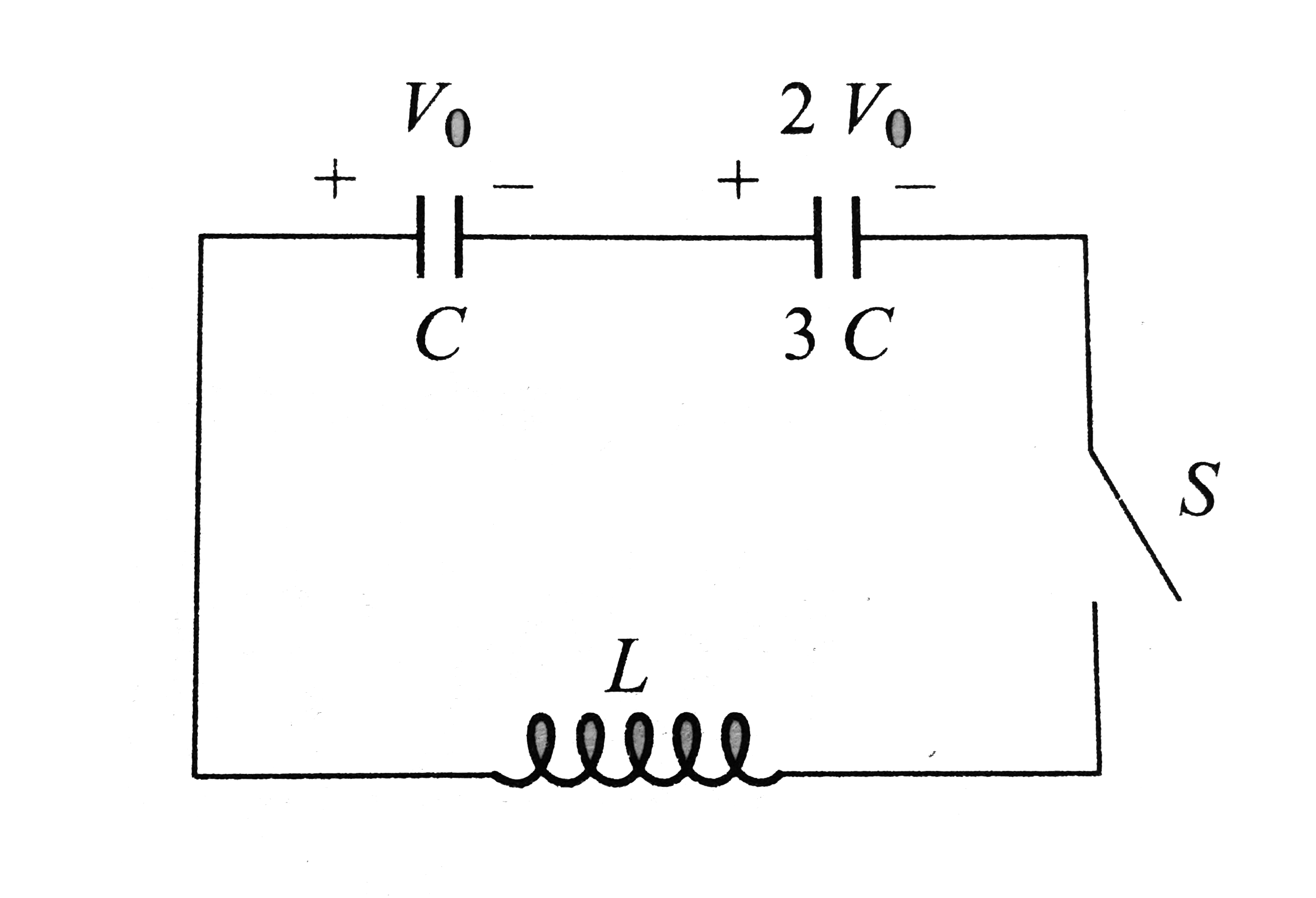
Potential difference across capacitor of capacitance 3C when the current in the circuit is maximum isView Solution

