A capacitor of capacity 2μF is changed to a potential different of 12V . It is then connected across an inductor of inductance 0.6mH What is the current in the circuit at a time when the potential difference across the capacitor is 6.0V ?
3.6A
2.4A
1.2A
0.6A
The correct Answer is:D
To solve the problem, we will use the principle of conservation of energy in an LC circuit. The energy stored in the capacitor will be transferred to the inductor as the circuit oscillates.
1. Calculate the initial energy stored in the capacitor:
The energy UC stored in a capacitor is given by the formula:
UC=12CV2
where:
- C=2μF=2×10−6F
- V=12V
Substituting the values:
UC=12×2×10−6×(12)2
UC=12×2×10−6×144=1.44×10−4J
2. Calculate the energy in the capacitor when the voltage is 6V:
When the potential difference across the capacitor is 6V, the energy UC2 stored in the capacitor is:
UC2=12CV2
where V=6V:
UC2=12×2×10−6×(6)2
UC2=12×2×10−6×36=3.6×10−5J
3. Use conservation of energy to find the energy in the inductor:
The initial energy in the capacitor will be equal to the sum of the energy in the capacitor and the energy in the inductor:
UC=UC2+UL
Rearranging gives:
UL=UC−UC2
Substituting the values:
UL=1.44×10−4−3.6×10−5=1.08×10−4J
4. Relate the energy in the inductor to the current:
The energy UL stored in the inductor is given by:
UL=12LI2
where L=0.6mH=0.6×10−3H. Setting the two expressions for UL equal gives:
1.08×10−4=12×0.6×10−3×I2
Rearranging for I2:
I2=2×1.08×10−40.6×10−3=2.16×10−40.6×10−3=0.36
5. Calculate the current I:
Taking the square root:
I=√0.36=0.6A
Final Answer:
The current in the circuit when the potential difference across the capacitor is 6.0V is 0.6A.
---
The energy UC stored in a capacitor is given by the formula:
UC=12CV2
where:
- C=2μF=2×10−6F
- V=12V
UC=12×2×10−6×(12)2
UC=12×2×10−6×144=1.44×10−4J
When the potential difference across the capacitor is 6V, the energy UC2 stored in the capacitor is:
UC2=12CV2
where V=6V:
UC2=12×2×10−6×(6)2
UC2=12×2×10−6×36=3.6×10−5J
The initial energy in the capacitor will be equal to the sum of the energy in the capacitor and the energy in the inductor:
UC=UC2+UL
Rearranging gives:
UL=UC−UC2
Substituting the values:
UL=1.44×10−4−3.6×10−5=1.08×10−4J
The energy UL stored in the inductor is given by:
UL=12LI2
where L=0.6mH=0.6×10−3H. Setting the two expressions for UL equal gives:
1.08×10−4=12×0.6×10−3×I2
Rearranging for I2:
I2=2×1.08×10−40.6×10−3=2.16×10−40.6×10−3=0.36
Taking the square root:
I=√0.36=0.6A
The current in the circuit when the potential difference across the capacitor is 6.0V is 0.6A.
Topper's Solved these Questions
Similar Questions
A capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 6μH. At an instant when potential difference across the capacitor is 6V, what is the current(in A)?
View SolutionIn the given circuit, with steady current, the potential difference across the capacitor is
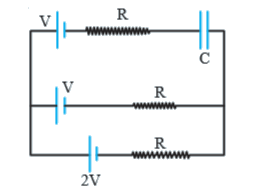
View Solution
Knowledge Check
A condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor when the potential difference across the condenser reduces to V2 is
A(C(V1−V2)2L)12BC(V21−V22)LCC(V21+V22)LD(C(V21−V22)L)12
given that potential differene across 1μF capacitor is 10V. Then,
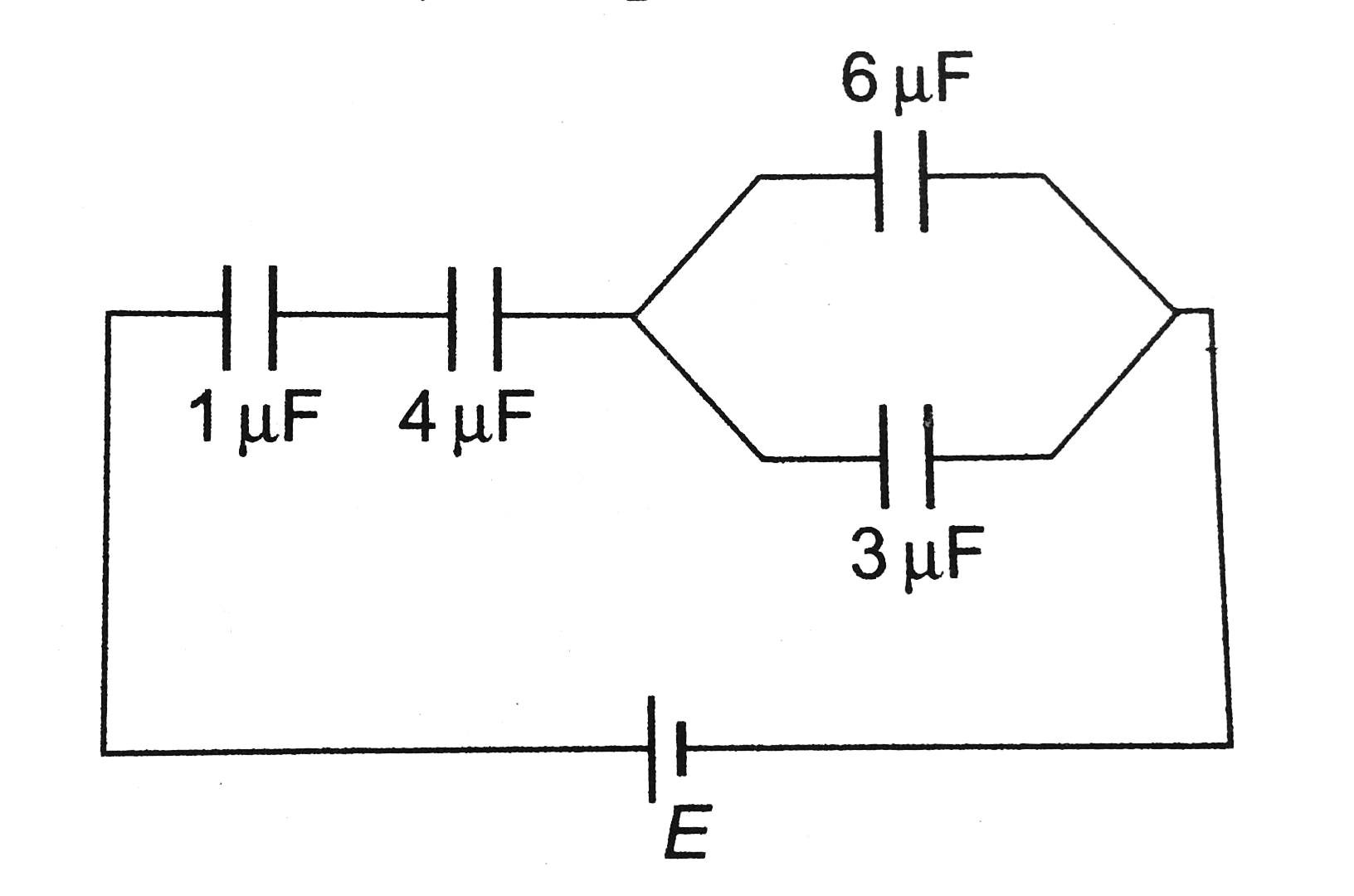
View SolutionIn the circuit shown in the figure, the potential difference across the 4.5μF capacitor is
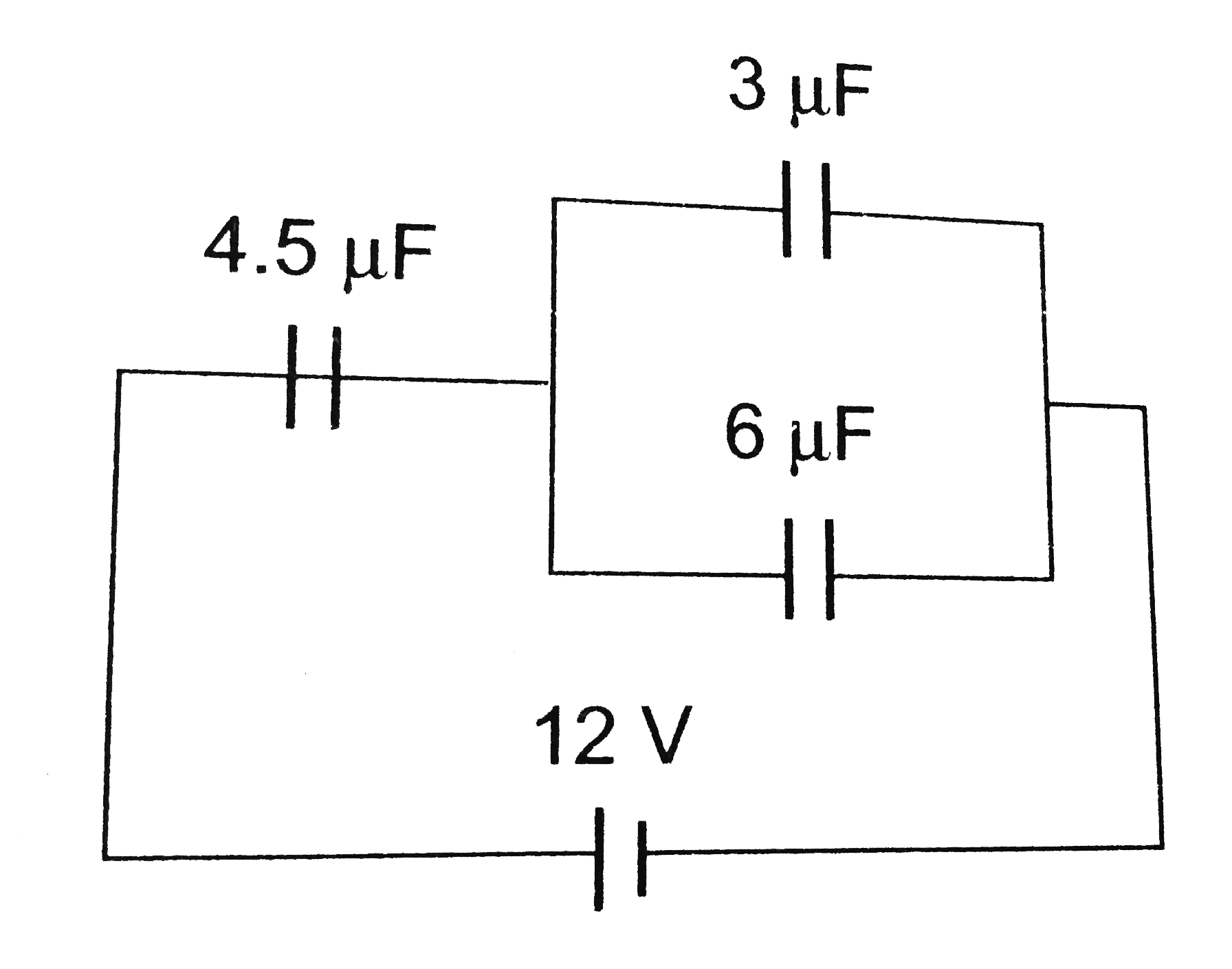
View SolutionIn the adjoning figure, the potential difference across the 4.5μF capacitor is

View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionIn the given circuit, find the potential differece across the 6μf capacitor in steady state.
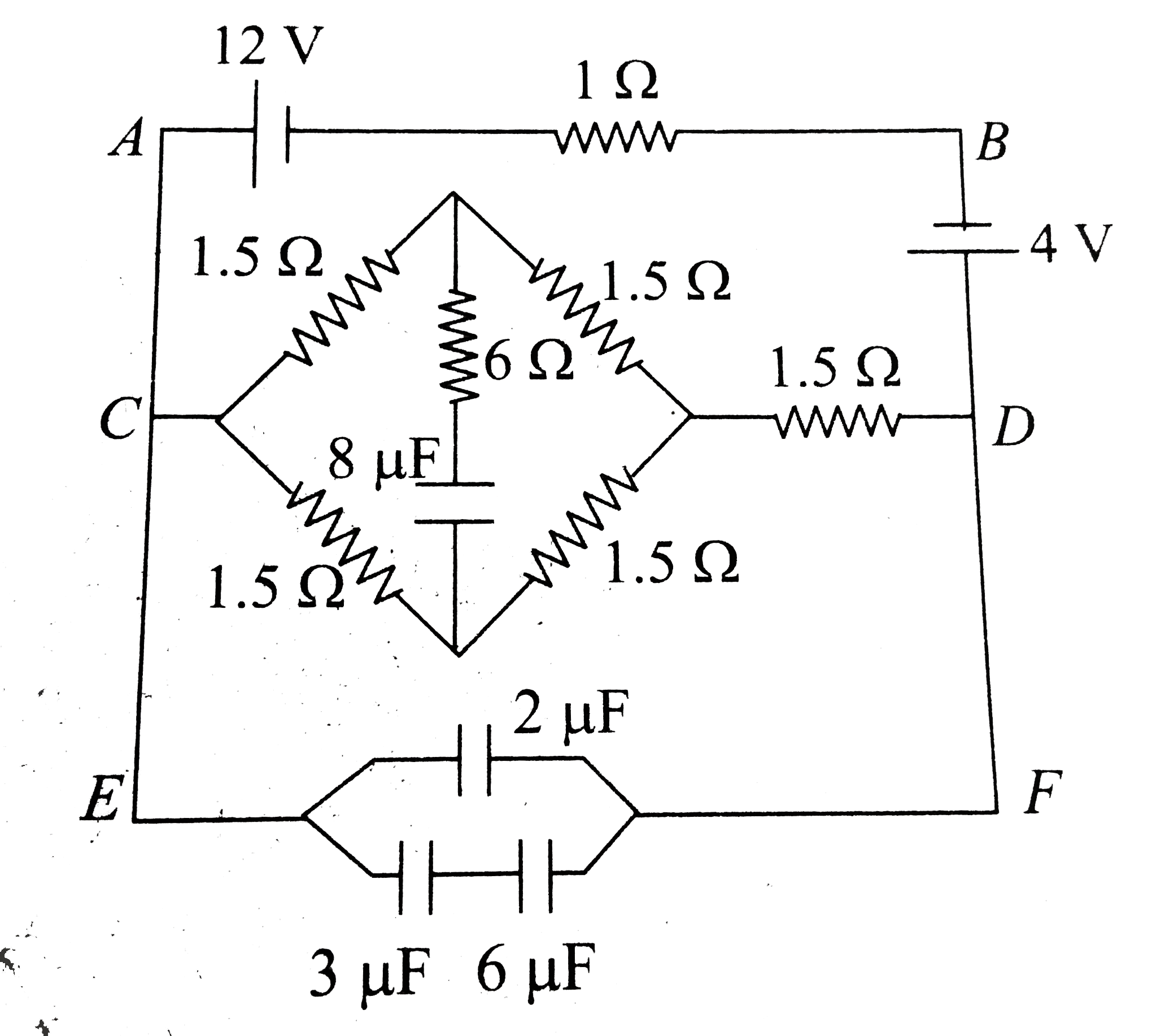
View SolutionIn the given circuit, the potential difference across the 6μF capacitor in steady state is
View SolutionA condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor wehnn the potential difference across the condenser reduces to V2 is
View Solution
