Let ρ(r)=QrπR4 be the charge density distribution for a soild sphere of radius R and total charge Q. For a point P inside the sphere at a distance r1 from the centre of the sphere, the magnitude of electric field is
Q4πε0r21
Qr214πε0R4
Qr213πε0R4
zero
The correct Answer is:B
To find the magnitude of the electric field at a point P inside a solid sphere with a given charge density distribution, we will follow these steps:
Step 1: Understand the Charge Density
The charge density is given by:
ρ(r)=QrπR4
This indicates that the charge density varies linearly with the distance r from the center of the sphere.
Step 2: Consider a Differential Element
To find the electric field at point P, which is at a distance r1 from the center, we consider a thin spherical shell of radius r and thickness dr. The surface area of this shell is:
dA=4πr2
Step 3: Calculate the Charge in the Differential Shell
The charge dq in the differential shell can be expressed as:
dq=ρ(r)⋅dV=ρ(r)⋅(dA⋅dr)=QrπR4⋅(4πr2⋅dr)=4Qr3R4dr
Step 4: Apply Gauss's Law
According to Gauss's Law, the electric field dE due to the charge dq at point P is given by:
dE=14πϵ0⋅dqr21
Substituting dq:
dE=14πϵ0⋅4Qr3R4r21dr
Step 5: Integrate to Find Total Electric Field
To find the total electric field E at point P, we integrate dE from r=0 to r=r1:
E=∫r10dE=∫r1014πϵ0⋅4Qr3R4r21dr
This simplifies to:
E=Qϵ0R4r21∫r10r3dr
Calculating the integral:
∫r10r3dr=(r44)r10=r414
Thus, substituting back:
E=Qϵ0R4r21⋅r414=Qr214ϵ0R4
Final Result
The magnitude of the electric field at point P inside the sphere is:
E=Qr214πϵ0R4
The charge density is given by:
ρ(r)=QrπR4
This indicates that the charge density varies linearly with the distance r from the center of the sphere.
To find the electric field at point P, which is at a distance r1 from the center, we consider a thin spherical shell of radius r and thickness dr. The surface area of this shell is:
dA=4πr2
The charge dq in the differential shell can be expressed as:
dq=ρ(r)⋅dV=ρ(r)⋅(dA⋅dr)=QrπR4⋅(4πr2⋅dr)=4Qr3R4dr
According to Gauss's Law, the electric field dE due to the charge dq at point P is given by:
dE=14πϵ0⋅dqr21
Substituting dq:
dE=14πϵ0⋅4Qr3R4r21dr
To find the total electric field E at point P, we integrate dE from r=0 to r=r1:
E=∫r10dE=∫r1014πϵ0⋅4Qr3R4r21dr
This simplifies to:
E=Qϵ0R4r21∫r10r3dr
Calculating the integral:
∫r10r3dr=(r44)r10=r414
Thus, substituting back:
E=Qϵ0R4r21⋅r414=Qr214ϵ0R4
The magnitude of the electric field at point P inside the sphere is:
E=Qr214πϵ0R4
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Higher Order Thinking Skills
7 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - NCERT Exemplar
5 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Application Of Gauss Law
9 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT Exemplar
6 Videos
Similar Questions
Let P(r)=QπR4r be the charge density distribution for a solid sphere of radius R and total charge Q. For a point 'p' inside the sphere at distance r1 from the centre of the sphere, the magnitude of electric field is:
View SolutionA hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionA non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
View SolutionA solid insulating sphere of radius R is given a charge Q. If at a point inside the sphere the potential is 1.5 times the potential at the surface, this point will be
View SolutionA conducting sphere of radius R is given a charge Q. The electric potential and the electric field at the centre of the sphere respectively are
View SolutionA conducting sphere of radius R is given a charge Q. The electric potential and the electric field at the centre of the sphere respectively are
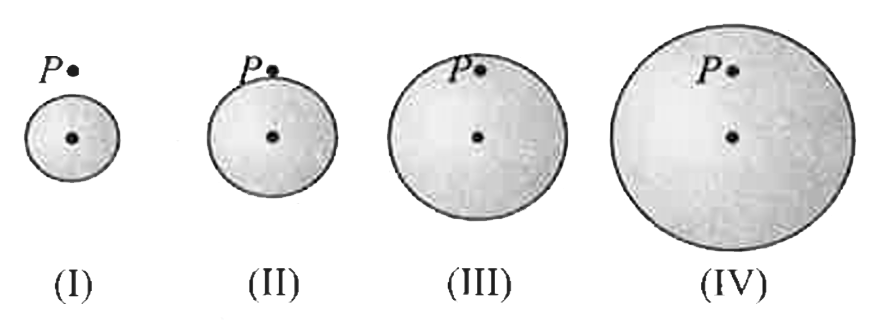
View SolutionFigure showns four solid spheres, each with charge Q uniformly distributed through its volume. The figure also shows a point P for each sphere. All at the same distance from the centre of the sphere. Rank the spheres according to the magnitude of the electric field they produce at point P.
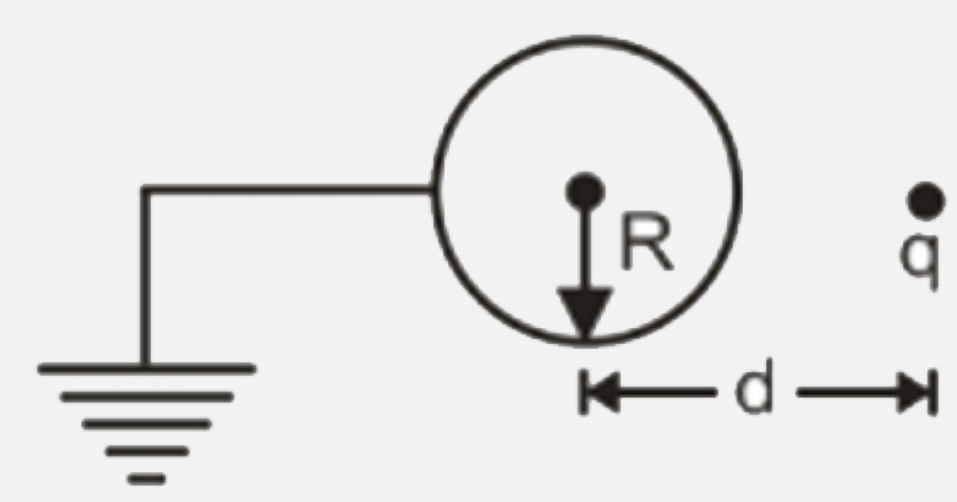
View SolutionIn the shown figure, the charge appearing on the conducting shell (grounded) of Radius R, when a point charge q is kept at a distance of d from the centre of the sphere is:
View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View Solution
