A steel wire of area of cross-section A and length 2L is clamped firmly between two points seperated by a distance .2L.. A body is hung from the middle point of the wire such that the middle point sags by a distance x. Calculate the mass of the body and the angle made by string with the horizontal Solution in Telugu
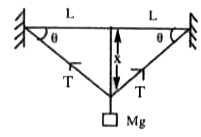
Since .θ. is small
sinθ=tanθ=xL
y=FA.Le
F=YAeL=YAL[(L2+x2)1/2−L]
F=YAL[L(1+x22L2)−L]=YAL[L+x22L−L]
F=YAx22L2,2Tsinθ=Mg
2(T)θ=Mg (∵ for small angles sinθ=θ)
2Fθ=Mg 2.YAx22L2θ=Mg
2YAx22L2.xL=Mg, M=YAX3L3g
xL=(MgYA)1/3,Tanθ=xL
Tanθ=(MgYA)1/3⇒θ=Tan−1(MgYA)1/3
Similar Questions
A steel wire of length 2L and cross-section area A is stretched, wetl within elastic limit horizontally between two pillars, a mass m is suspended from the mid-point of wire such that depression at the middle is X. Strain in the wire is proportional to
View SolutionA mild steel wire of length 2L and cross-sectional area A is stretched, well within elastic limit, horizontally between two pillars, A mass m is suspended from the midpoint of the wire. Strain in the wire is
View SolutionA steel wire of diameter 0.8 mm and length 1m is clamped firmly at two points P and Q which are one metre apart and in the same horizontal plane. A body is hung from the middle point of the wire, such that the middle point sags one cm lower from the original position as shown in figure. Calculate the mass of the body. Y = 2xx 10^(11) N//m^(2) .
View SolutionA steel wire of length 2L and cross-section area A is stretched, wetl within elastic limit horizontally between two pillars, a mass m is suspended from the mid-point of wire such that depression at the middle is x. Strain in the wire is proportional to
View SolutionA wire of cross section A is stretched horizontally between two clamps located 2lm apart. A weight Wkg is suspended from the mid-point of the wire. If the mid-point sags vertically through a distance x<l, the strain produced is
View SolutionA steel wire of diameter 0.8mm and length 1m is clamped firmly at two points A and B which are 1m apart and in the same plane. A body is hung from the middle point of the wire such that the middle point sags 1cm lower from the original position. Calculate the mass of the body. Given that Young's modulus of the material of wire is 2xx10^(11)N//m^(2) .
View SolutionA mild steel wire of length 2L and cross-sectional area A is stretched, well within elastic limit, horizontally between two pilars show in the figure A mass m is suspended from the mid point of the wire. Strain in the wire is .
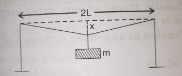
View SolutionA steel wire of negligible mass, length 2I , cross sectional area 'A' and Young's modulus 'Y' is held between two rigid walls. A small body of mass 'm' is suspended from its middle point 'O' as shown. The vertical descent of the middle point 'O' of the wire is
View Solution
