Obtain the expression for the deflecting torque acting on the current carrying rectangular coil of a galvanometer in a uniform magnetic field. Why is a radial magnetic field employed in the moving coil galvanometer?

Text Solution

Verified by Experts

Consider a rectangular coil PQRS, of length $l$ and breadth b, of a galvanometer carrying a current I, placed in a uniform magnetic field B such that a vector normal to the plane of the coil subtends an angle $θ$ from the direction of B.

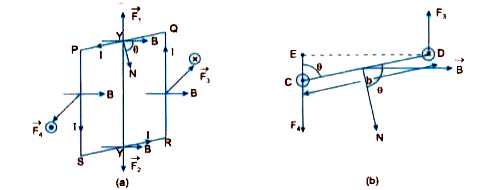
In this situation forces $F_{1} and F_{2}$ having magnitude I b B $sin θ$ are acting on arms PQ and RS. The forces are equal, opposite and collinear, hence they cancel out.
Again forces $F_{3} and F_{4}$ having magnitude $I l B$ are acting on each of the two arms QR and SP. These forces too are equal and opposite but these are non-collinear and form a couple whose torque is given as:
$τ = (I l B) \times b sin θ = I (l b) B sin θ = I A B sin θ$
where $A = l b =$ area of the loop. If instead of a single loop, we have a rectangular coil having N turns, then
torque $τ = N I A B sin θ$
In vector notation $\to τ = N I (\to A \times \to B) = (\to m \times \to B)$ , where $\to m = N I \to A =$ magnetic moment of current carrying coil.
A radial magnetic field, in which $θ = 90^{\circ}$ , is employed in the moving coil galvanometer because now torque acting on the coil does not change with orientation of the coil.

Updated on:21/07/2023

To make the field radial in a moving coil galvanometer
AThe number of turns in the coil is increased
BMagnet is taken in the form of horse-shoe
CPoles are cylindrically cut
DCoil is wounded on aluminium frame
To make the field radial in moving coil galvanometer
Athe number of turns in the coil is increased
Bpoles are cylindrically cut
Cmagnet is taken in the form of horse shoe
Dcoil is wound an aluminium frame