Question

What is a wavefront? How does it propagate? Using Huygens' principle, explain reflection of a plane wavefront from a surface and verify the laws of reflection.

Explanation

A wavefront is defined as a surface of constant phase. Propagation of a wavefront is based on following two points:
(i) Every point on a given wavefront acts as fresh source of secondary wavelets, which travel in all directions with the speed of wave (i.e., of light) in the medium.
(ii) The common envelope (tangent) of these secondary wavelets in the forward direction gives the new wavefront at that instant.
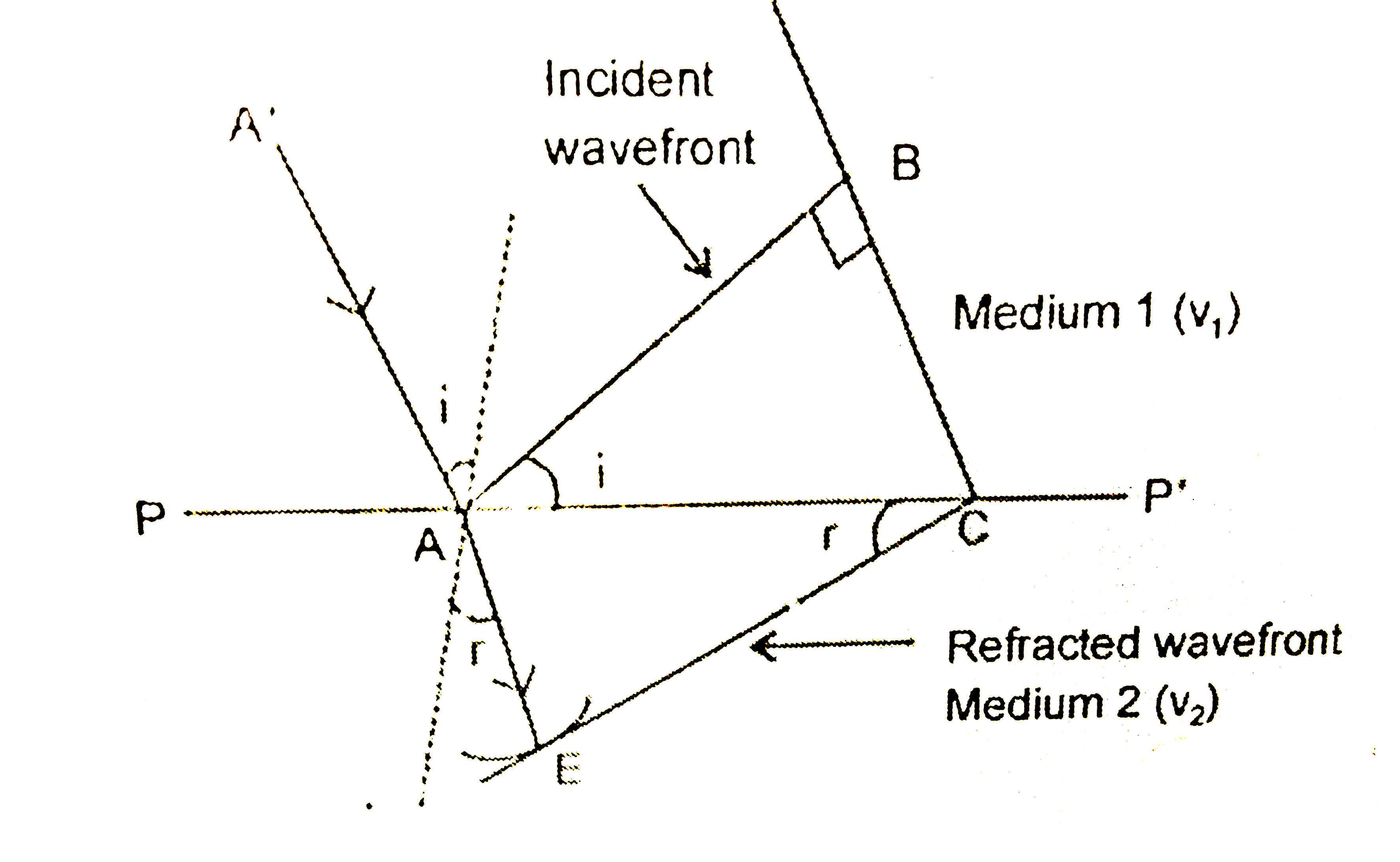
Let us consider a plane wavefront AB incident obliquely on a plane reflecting surface MM.. Let one end A of wavefront strikes the mirror at an angle i but the other end B has still to cover distance BC. Time required for this will be `t=(BC)/(c )`, where c is the speed of light.
According to Huygen.s principle point A starts emitting secondary wavelets and in time t, these will cover a distance `ct=c.(BC)/(c )=BC` and spread. Hence, with point A as centre and BC as radius, draw a circular arc. Draw tangent CD on this arc from the point C. Obviously, CD is the reflected wavefront inclined at an angle r. Obviously the incident and reflected both are in the plane of paper.

Again in `DeltaABC and DeltaADC`, we have
`BC=AD` (by construction)
`angleABC=angleADC=90^(@)`
and AC is common.
So, the two triangles are congruent and,
hence, `angleBAC=angleDCA`
or `angle i=angler` i.e., the angle of reflection is equal to the angle of incidence.