For a reaction graph between logK vs 1T is as with slope = -10,000.
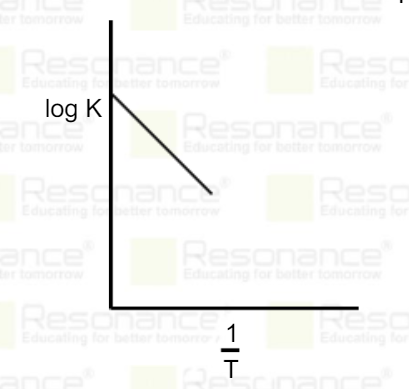
At temperature 500K rate constant k = 10−5 .
Find the temperature in K at which rate constant = 10−4
Step by step video & image solution for For a reaction graph between logK vs 1/T is as with slope = -10,000. At temperature 500K rate constant k = 10^(-5) . Find the temperature in K at which rate constant = 10^(-4) by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Graph of logK and 1/T was given for which valuue of slope is -10000 K if T is 500K and rate constant is 10−5 at which temperature value rate constant will be 10−4
View SolutionFor the reaction aA+bB→cC+dD, the plot of log k vs 1T is given below,
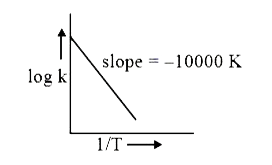
The temperature at which the rate constant of the reaction is 10−4s−1 is _____K.
(Rouded -off the nerest integer)
[Given : The rate constant of the reaction is 10−5s−1 at 500 K.]
View Solution
Knowledge Check
Graph between log k and 1/T [where K is rate constant in s−1 and T is the temperature (in K) is a straight line with Hence, Ea will be
 A2.303×2 calB22.303calC2 calDNone of these
A2.303×2 calB22.303calC2 calDNone of theseFor a reaction, consider the plot of In K versus 1/T given in the figure. If the rate constant of this reaction at 400 K is 10−5s−1, then the rate constant at 500K is :
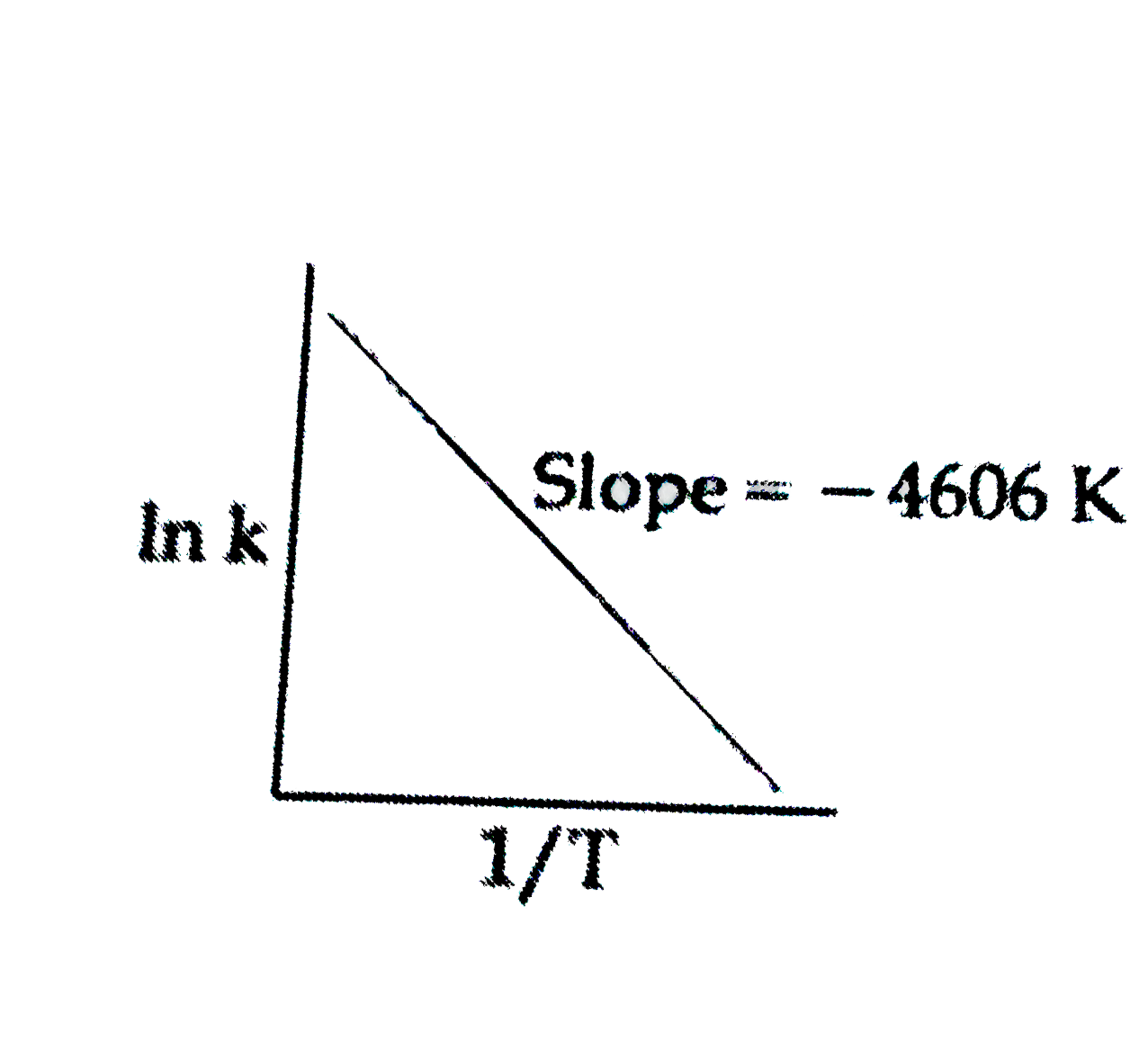 A10−6S−1B2×10−4s−1C10−4s−1D4×10−4s−1
A10−6S−1B2×10−4s−1C10−4s−1D4×10−4s−1The slope of straight line graph between ln k us 1T is equal to 2.4×104K. Calculate the activation energy of the reaction at 400 K. (K represents rate constant)
A24 kJB48 kcalC9600 kcalD48 kJ
The rate of a reaction increases to 2.3 times when the temperature is raised form 300K to 310K. If Q is the rate constant at 300K, then the rate constant at 310K will be equal to
View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be

View SolutionFor a reaction, activation energy (Ea) =0 and rate constant, k=1.5x104s−1 at 300 K. What is the value of rate constant at 320 K'?
View SolutionGraph between logk and 1/T [k rate constant (s−1) and T and the temperature (K)] is a straight line with OX=5,θ=tan−1(1/2.303). Hence −Ea will be

View SolutionGraph between log k and 1T (k is rate constant in s−1 and T is the temperature in K) is a straight line. As shown in figure if OX = 5 and slope of the line =−12.303then Ea is :

View Solution