In ΔABC, if BC=a,CA=bandAB=C, then what is the value of ¯¯¯¯¯¯AB.¯¯¯¯¯¯AC+¯¯¯¯¯¯BC.¯¯¯¯¯¯BA+¯¯¯¯¯¯CA.¯¯¯¯¯¯CB?Solution in Telugu
a2+b2+c2
12(a2+b2+c2)
2(a2+b2+c2)
abc
The correct Answer is:B
Step by step video, text & image solution for In DeltaABC, " if " BC = a, CA = b and AB = C, " then what is the value of " bar(AB).bar(AC)+bar(BC).bar(BA)+bar(CA).bar(CB)? by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DOT PRODUCT OF TWO VECTORSBOOK - AAKASH SERIESCHAPTER - DOT PRODUCT OF TWO VECTORSEXERCISE - EXERCISE - II
71 Videos
- DIRECTION COSINES AND RATIOSBOOK - AAKASH SERIESCHAPTER - DIRECTION COSINES AND RATIOSEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
8 Videos
- ERRORS AND APPROXIMATIONSBOOK - AAKASH SERIESCHAPTER - ERRORS AND APPROXIMATIONSEXERCISE - ADVANCED SUBJECTIVE TYPE QUESTION
15 Videos
Similar Questions
If ¯a,¯b,¯c are three non zero vectors, and ¯a.¯b=¯a.¯c⇒
View SolutionIf the line joining the points (2,k,5), (0,1,6) is parallel to the line joining the points (3,-1,2), (-1,2,4) then find k,
View SolutionFind the approximate value of log (2.01) give that log 2 = 0.6934
View SolutionIn DeltaABC, |bar(CB)| = a, |bar(CA)| = b and |bar(AB)| =c. CD is the median through the vertex C. Then bar(CA).bar(CD)=
View SolutionABC is an equilateral triangle of side ‘a'. Then bar(AB).bar(BC) +bar(BC).bar(CA)+ bar(CA).bar(AB) =
View SolutionProve the following boolean relations. bar(AB + BC + CA) = bar(A)bar(B) + bar(B)bar(C ) +bar(C)bar(A)
View SolutionIf the midpoint of the sides ¯¯¯¯¯¯BC,¯¯¯¯¯¯CA,¯¯¯¯¯¯AB of ΔABC are (3, -3), (3, -1), (1, 1) respectively then the vertices A, B, C are
View SolutionA,B,C,D are four points in space and ∣∣¯¯¯¯¯¯ABׯ¯¯¯¯¯CD+¯¯¯¯¯¯BCׯ¯¯¯¯¯AD+¯¯¯¯¯¯CAׯ¯¯¯¯¯BD∣∣=λ (area ΔABC) then value of λ is _____
View Solutionత్రిభుజం ABC లో, ¯¯¯¯¯¯BC=¯a, ¯¯¯¯¯¯CA=¯b,¯¯¯¯¯¯AB=¯c అయితే, ¯a∗¯b=¯b∗¯c=¯c∗¯aఅని చూపండి.
View SolutionIn the adjacent figure ΔABC is isosceles as ¯¯¯¯¯¯AB=¯¯¯¯¯¯AC,¯¯¯¯¯¯BAand¯¯¯¯¯¯CA are produced to Q and P such that ¯¯¯¯¯¯AQ=¯¯¯¯¯¯AP. . Show that ¯¯¯¯¯¯PB=¯¯¯¯¯¯QC.
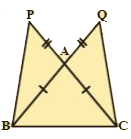
View SolutionIn the adjacent figure ΔABC is isosceles as ¯¯¯¯¯¯AB=¯¯¯¯¯¯AC,¯¯¯¯¯¯BAand¯¯¯¯¯¯CA are produced to Q and P such that ¯¯¯¯¯¯AQ=¯¯¯¯¯¯AP. . Show that ¯¯¯¯¯¯PB=¯¯¯¯¯¯QC.
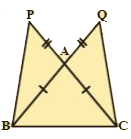
View SolutionIn the adjacent figure ΔABC is isosceles as ¯¯¯¯¯¯AB=¯¯¯¯¯¯AC,¯¯¯¯¯¯BAand¯¯¯¯¯¯CA are produced to Q and P such that ¯¯¯¯¯¯AQ=¯¯¯¯¯¯AP. . Show that ¯¯¯¯¯¯PB=¯¯¯¯¯¯QC.
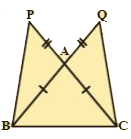
View SolutionIn a right angled triangle ABC, hypotenuse AB=P then ¯¯¯¯¯¯AB.¯¯¯¯¯¯AC+¯¯¯¯¯¯BC.¯¯¯¯¯¯BA+¯¯¯¯¯¯CA.¯¯¯¯¯¯CB = ………
View SolutionIf AD, BE and CF are the medians of ΔABC, then the value of ¯¯¯¯¯¯BC.¯¯¯¯¯¯AD+¯¯¯¯¯¯CA.¯¯¯¯¯¯BE+¯¯¯¯¯¯AB.¯¯¯¯¯¯CF=
View SolutionIf A,B,C,D are four point and ∣∣¯¯¯¯¯¯ABׯ¯¯¯¯¯CD+¯¯¯¯¯¯BCׯ¯¯¯¯¯AD+¯¯¯¯¯¯CAׯ¯¯¯¯¯BD∣∣=k(Area of ΔABC), then k =
View SolutionA point I is the centre of a circle inscribed in a triangle ABC then show that
∣∣¯¯¯¯¯¯BC∣∣¯¯¯¯¯IA+∣∣¯¯¯¯¯¯CA∣∣¯¯¯¯¯IB+∣∣¯¯¯¯¯¯AB∣∣¯¯¯¯¯IC=¯0
View Solution
