The moment of inertia of a flat angular ring having mass M, inner radius a and outer radius b about the diametric axis through the centre is
M2(b2+a2)
M2(b2−a2)
M4(b−a)
M4(b2−a2)
To find the moment of inertia of a flat angular ring with mass M, inner radius a, and outer radius b about the diametric axis through the center, we can follow these steps:
Step 1: Understand the Geometry
The flat angular ring is essentially a hollow cylinder with an inner radius a and an outer radius b. The mass M is distributed between these two radii.
Step 2: Use the Formula for Moment of Inertia
The moment of inertia I of a thin ring about its central axis is given by:
I=12M(R21+R22)
where R1 and R2 are the inner and outer radii respectively.
Step 3: Apply the Parallel Axis Theorem
Since we need the moment of inertia about the diametric axis (which is perpendicular to the axis through the center), we can use the parallel axis theorem. The moment of inertia about the diametric axis Id can be expressed as:
Id=Icm+Md2
where d is the distance from the center of mass to the axis of rotation. For a ring, this distance is zero when rotating about the center.
Step 4: Calculate the Moment of Inertia
For our ring:
1. The moment of inertia about the central axis (using the formula for a ring) is:
Icm=12M(a2+b2)
2. Since we are rotating about the diametric axis, we do not need to add any additional term (as d=0):
Id=Icm=12M(a2+b2)
Step 5: Final Expression
Thus, the moment of inertia of the flat angular ring about the diametric axis through the center is:
Id=12M(a2+b2)
Summary of Steps
1. Understand the geometry of the ring.
2. Use the formula for the moment of inertia of a ring.
3. Apply the parallel axis theorem if necessary.
4. Calculate the moment of inertia using the derived formula.
5. State the final expression.
The flat angular ring is essentially a hollow cylinder with an inner radius a and an outer radius b. The mass M is distributed between these two radii.
The moment of inertia I of a thin ring about its central axis is given by:
I=12M(R21+R22)
where R1 and R2 are the inner and outer radii respectively.
Since we need the moment of inertia about the diametric axis (which is perpendicular to the axis through the center), we can use the parallel axis theorem. The moment of inertia about the diametric axis Id can be expressed as:
Id=Icm+Md2
where d is the distance from the center of mass to the axis of rotation. For a ring, this distance is zero when rotating about the center.
For our ring:
1. The moment of inertia about the central axis (using the formula for a ring) is:
Icm=12M(a2+b2)
2. Since we are rotating about the diametric axis, we do not need to add any additional term (as d=0):
Id=Icm=12M(a2+b2)
Thus, the moment of inertia of the flat angular ring about the diametric axis through the center is:
Id=12M(a2+b2)
1. Understand the geometry of the ring.
2. Use the formula for the moment of inertia of a ring.
3. Apply the parallel axis theorem if necessary.
4. Calculate the moment of inertia using the derived formula.
5. State the final expression.
Topper's Solved these Questions
Similar Questions
The moment of inertia of a flat angular ring is having mass M, inner radius a and outer radius b about the perpendicular axis through the centre is
View SolutionCalculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
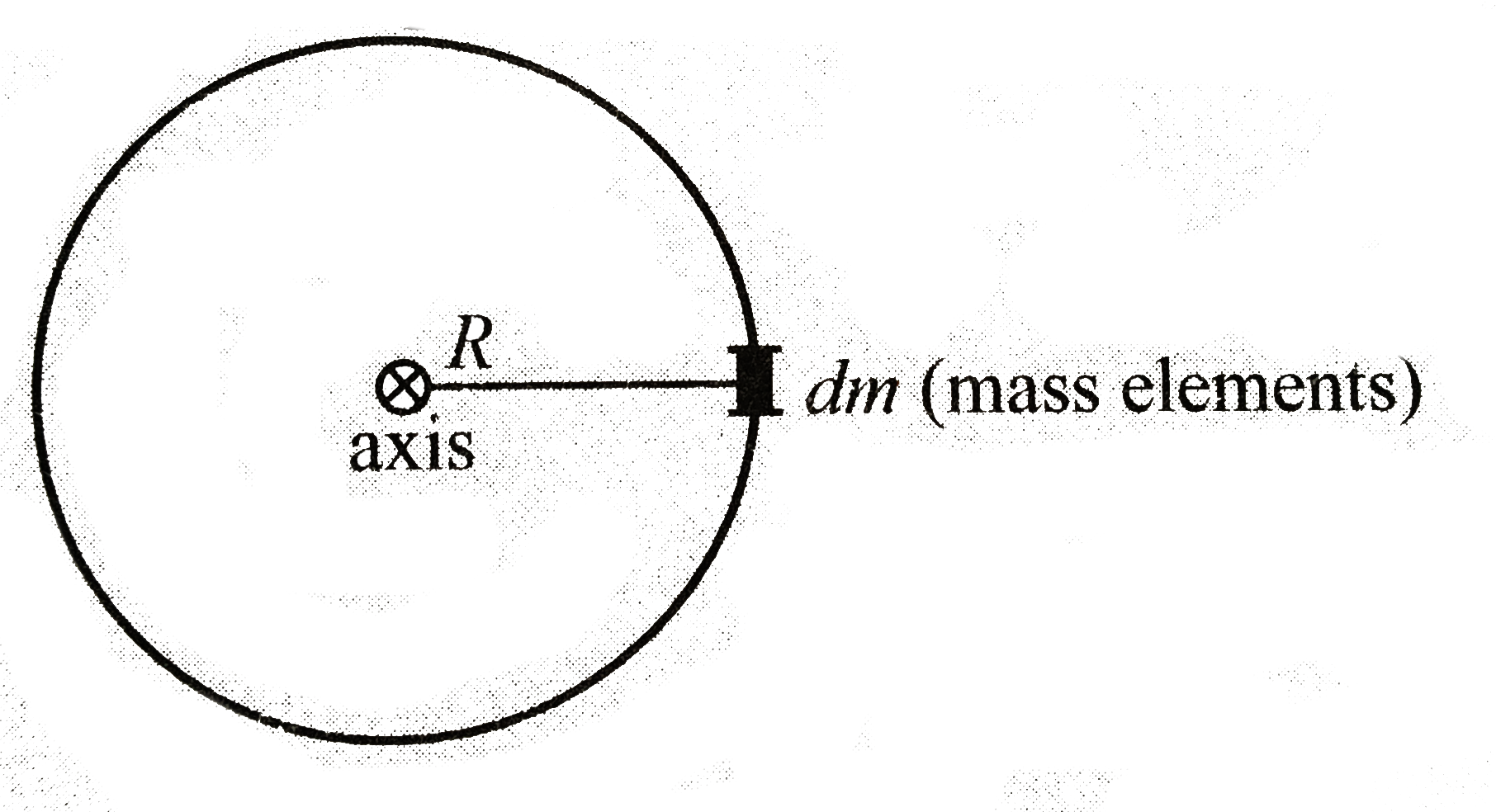
View Solution
Knowledge Check
Moment of inertia of a thin circular plate of mass M, radius R about an axis passing through its diameter is I. The moment of inertia of a circular ring of mass M, radius R about an axis perpendicular to its plane and passing through its centre is
A2IBI2C4IDI4The moment of inertia of a uniform semicircularwire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is :
A12Mr2BMt2C25Mr2D14Mr2The moment of inertia of hollow sphere (mass M) of inner radius R and outer radius 2R, having material of uniform density, about a diametric axis is
A31MR2/70B43MR2/90C19MR2/80DNone of these
The moment of inertia of a ring of mass M and radius R about PQ axis will be

View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be :-

View SolutionFind the moment of inertia of uniform ring of mass M and radius R about a diameter.
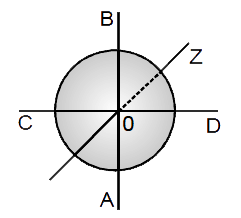
View SolutionFind the moment of inertia of a half uniform ring (mass m, radius r) about its center.
View SolutionThe moment of inertia of a uniform circular ring, having a mass M and a radius R, about an axis tangential to the ring and perpendicular to its plane, is
View Solution
