A object is sliding along the frictionless floor with initial velocity (a) Describe the motion of the object relation to car (b) when does the object reach its original position relative to the box car.
To solve the problem step by step, we will analyze the motion of the object relative to the boxcar and determine when it returns to its original position.
Step 1: Understand the System
We have a 2 kg object sliding on a frictionless floor with an initial velocity of 10m/s^i. The boxcar is considered a non-inertial frame of reference.
Step 2: Set Up the Motion Equation
In a non-inertial frame (the boxcar), we can describe the motion of the object using the equation:
x=x0+uxt+12axt2
where:
- x is the position of the object at time t,
- x0 is the initial position of the object,
- ux is the initial velocity (which is 10m/s),
- ax is the acceleration (which is 0 since the floor is frictionless).
Step 3: Substitute Values
Since the object is moving with constant velocity (no acceleration), we can simplify the equation:
x=x0+10t
Here, we ignore the acceleration term because ax=0.
Step 4: Determine the Relative Motion
The boxcar is also moving, but we need to establish its motion. If we assume the boxcar is moving with some constant velocity vb, the relative position of the object with respect to the boxcar can be expressed as:
xrelative=x−xboxcar
Assuming the boxcar starts from the same position x0 and moves with a constant velocity vb, we have:
xboxcar=x0+vbt
Step 5: Find When the Object Returns to the Original Position
To find when the object returns to its original position relative to the boxcar, we set:
xrelative=0
This gives us:
x0+10t−(x0+vbt)=0
Simplifying this, we get:
10t−vbt=0
Factoring out t:
t(10−vb)=0
This implies either t=0 (the initial time) or 10−vb=0, which means vb=10m/s.
Step 6: Calculate Time to Return to Original Position
If the boxcar is moving at 10m/s, the object will return to its original position relative to the boxcar after:
t=x010−vb
Since vb=10m/s, the object will never return to the original position relative to the boxcar unless it stops or the boxcar stops.
Conclusion
(a) The object moves with a constant velocity of 10m/s relative to the ground, and if the boxcar moves at the same speed, it will not appear to move relative to the boxcar.
(b) The object will reach its original position relative to the boxcar only if the boxcar stops moving or if the object stops moving.
We have a 2 kg object sliding on a frictionless floor with an initial velocity of 10m/s^i. The boxcar is considered a non-inertial frame of reference.
In a non-inertial frame (the boxcar), we can describe the motion of the object using the equation:
x=x0+uxt+12axt2
where:
- x is the position of the object at time t,
- x0 is the initial position of the object,
- ux is the initial velocity (which is 10m/s),
- ax is the acceleration (which is 0 since the floor is frictionless).
Since the object is moving with constant velocity (no acceleration), we can simplify the equation:
x=x0+10t
Here, we ignore the acceleration term because ax=0.
The boxcar is also moving, but we need to establish its motion. If we assume the boxcar is moving with some constant velocity vb, the relative position of the object with respect to the boxcar can be expressed as:
xrelative=x−xboxcar
Assuming the boxcar starts from the same position x0 and moves with a constant velocity vb, we have:
xboxcar=x0+vbt
To find when the object returns to its original position relative to the boxcar, we set:
xrelative=0
This gives us:
x0+10t−(x0+vbt)=0
Simplifying this, we get:
10t−vbt=0
Factoring out t:
t(10−vb)=0
This implies either t=0 (the initial time) or 10−vb=0, which means vb=10m/s.
If the boxcar is moving at 10m/s, the object will return to its original position relative to the boxcar after:
t=x010−vb
Since vb=10m/s, the object will never return to the original position relative to the boxcar unless it stops or the boxcar stops.
(a) The object moves with a constant velocity of 10m/s relative to the ground, and if the boxcar moves at the same speed, it will not appear to move relative to the boxcar.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
28 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A 2kg object is slide along the frictionless floor with intial transverse volocity (10m/s)ˆk (a) Describe the motion (a) in car's frame and (b) in ground frame.
View SolutionA 2kg object is slide along a rough floor (coefficient of sliding friction =0.3)with initial volocity (10m/s)ˆi. Describe the motion of the object relation to car assuming that the coefficient of static friction is greater than 0.5.
View Solution
Knowledge Check
m=M√1−v2c2
The equation above describes, according to Einstein's theory of relativity, how the mass of an object increase with velocity where m is the mass of moving object, M is the mass the mass the object when it is not moving, v is the velocity of the object relative to a stationary observer, and c is the speed of light. Which of the following expresses v in terms of m, M, and c?Ac√1−(Mm)2Bc√1+(Mm)2C√c2+(Mm)2D√c2+(Mm)2−1
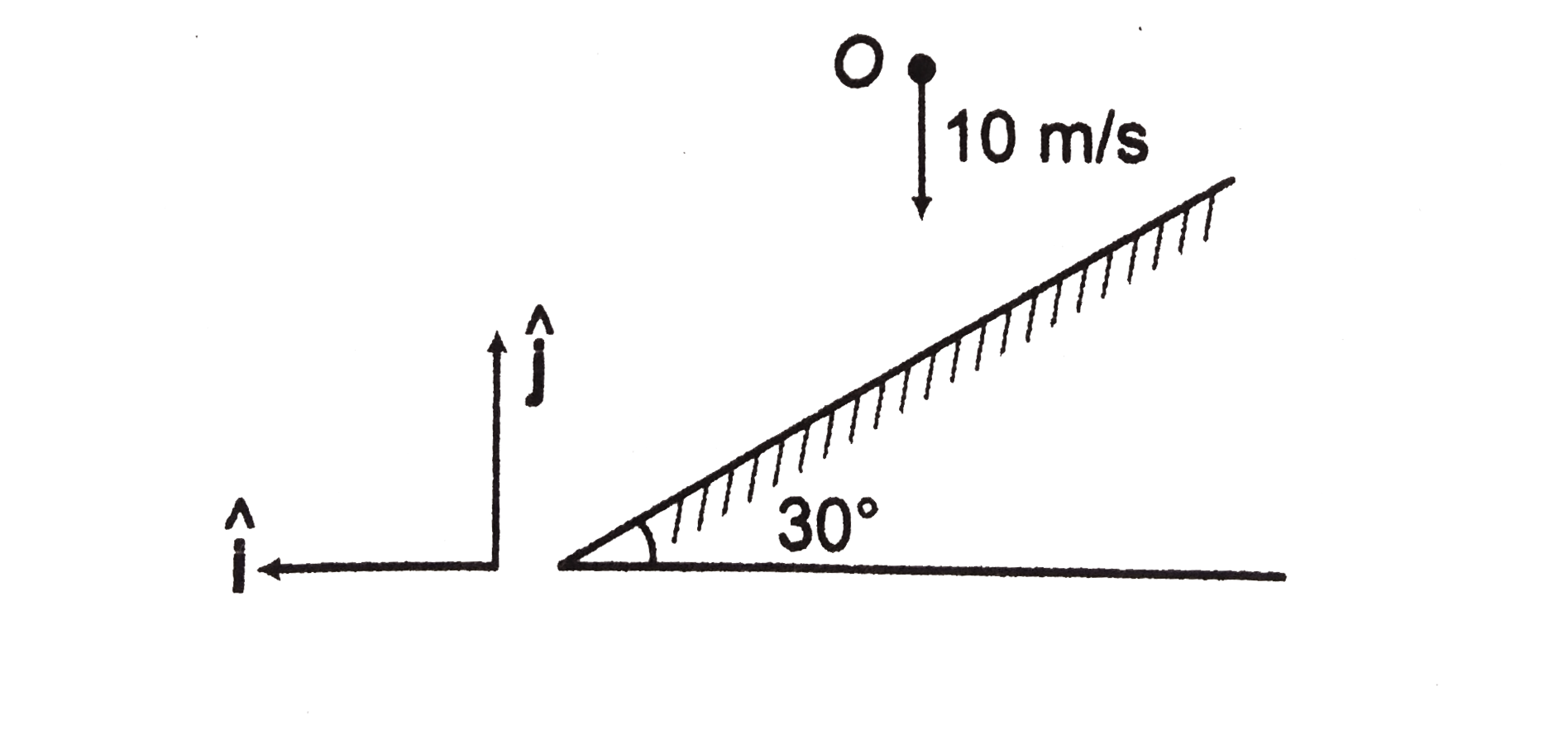
An object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
View SolutionAn object of mass 1 kg moving on a horizontal surface with initial velocity 8 m/s comes to rest after 10 s. If one wants to keep the object moving on the same surface with velocity 8 m/s the force required is
View SolutionA projectile is projected in air with initial velocity →v=(3ˆi+4ˆj) m/s from the origin. The equation of trajectory of the projectile is given as (g=−10ˆjms2) (neglect air resistance )
View SolutionAn object of mass 5 kg moves at a constant speed of 6 m/s in a circular path of radius 2m. Find the magnitude of the object's acceleration and the net force responsible for its motion.
View SolutionA 5 kg mass attached to a spring scale rests on a frictionless , horizontal surface as in figure . The spring scale, attached to the front end of a box car, reads 18 N when the car is in motion .
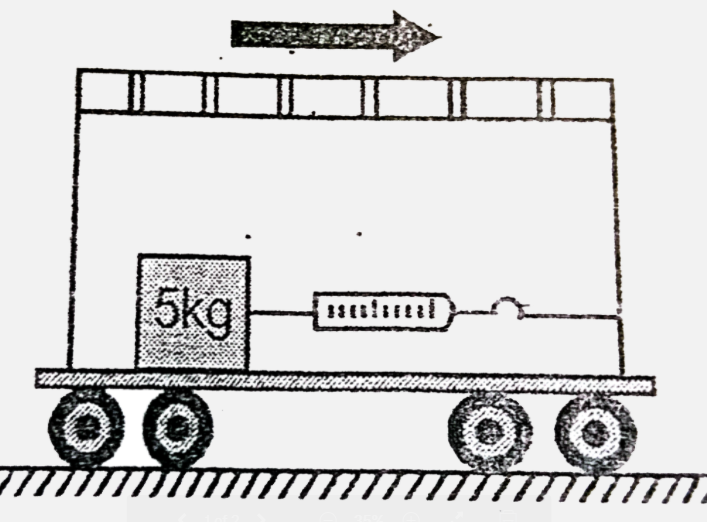
(a) If the spring reads zero when the car is at rest , determine acceleration of the car while it is in motion .
(b) What will the spring scale read if the car moves with constant velocity ?
(c ) Describe the forces on the mass as observed by someone in the car and by someone at rest outside the car.
View SolutionThe velocity of an object is changing with time and relation is given by the following equation.
v=2t+3t2
Calculate the position of the object from the origin at t = 2 s.
Assume particle to be at origin at t = 0
View SolutionA man of mass 100kg stands at the rim of a turtable of radius 2m and moment of inertia 4000kgm2 mounted on a vertical frictionless shaft at its centre. The whole system is initially at rest. The man now walks along the outer edge of the turntable with a velocity of 1m/s relative to the earth
a. With what angular velocity and in what direction does the turntable rotate?
b. Through what angle will it have rotated when the man reaches his initial position on the turntable?
c. Through what angle will it have rotated when the man reaches his initial position relative to the earth?
View Solution
