All the surface and pulleys are frictionless in the shown arrangement. Pulleys P and Q are massless .The force applied by clamp on pulley P is
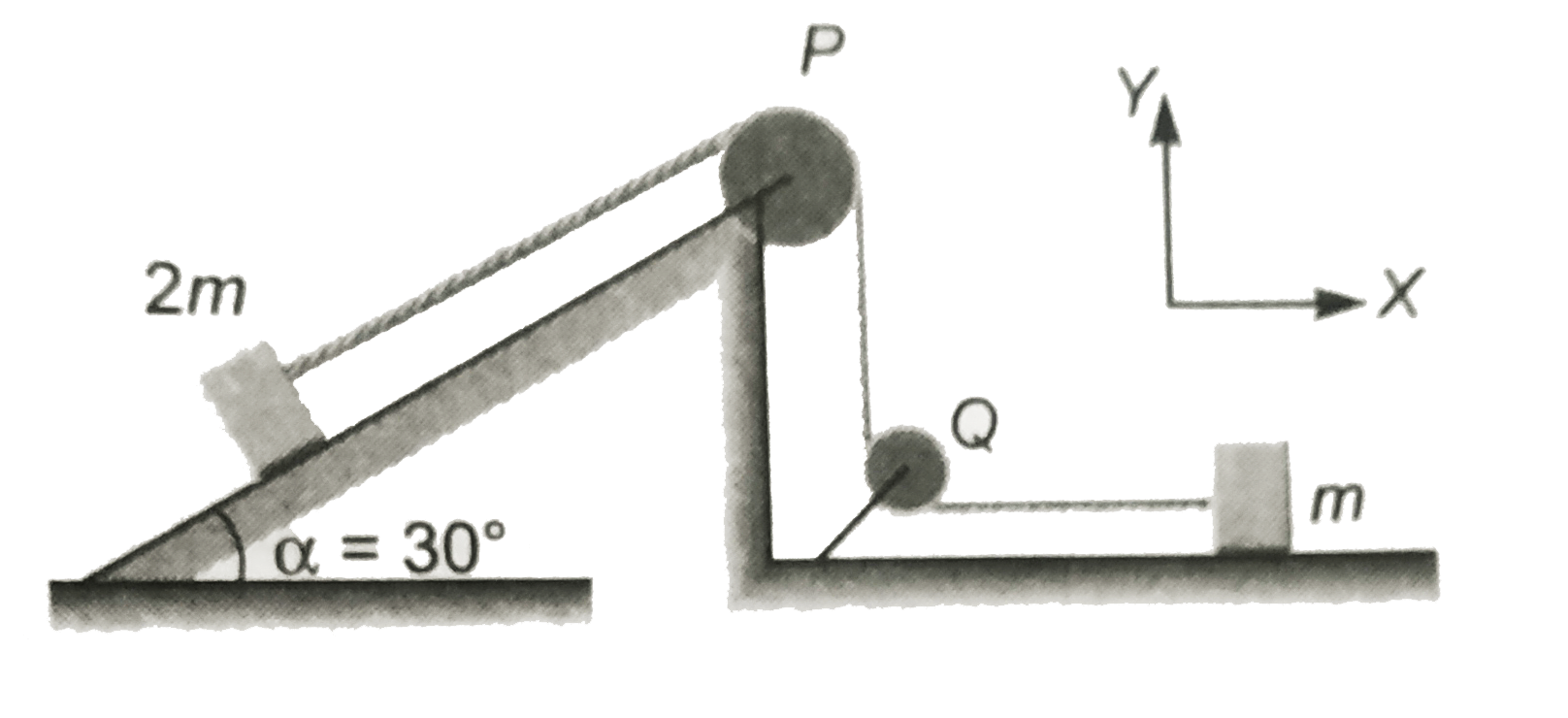
mg6(−√3ˆi−3ˆj)
mg6(√3ˆi+3ˆj)
mg6√2
None of these
The correct Answer is:B
a=Net pulling forceTotal mass
=2mgsin30∘2m+m=g3
FBDofm
T=ma=mg/3
Resultant of tensions
R=−(Tcos30∘)ˆi−(Tsin30∘+T)ˆj
Putting T=mg/3
R=−√36mgˆi−mg2ˆj
Since pulley P is in equilibrium. Therefore,
F+R=0
when , F= force applied by clamp on pulley
∴F=−R=mg6(√3ˆi+3ˆj)
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
28 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
In the arrangement shown, the pulleys and strings are massless. The acceleration of block B is :
View SolutionThree blocks of masses m1,m2 and M are arranged as shown in figure. All the surfaces are fricationless and string is inextensible. Pulleys are light. A constant force F is applied on block of mass m1. Pulleys and string are light. Part of the string connecting both pulleys is vertical and part of the strings connecting pulleys with masses m1 and m2 are horizontal.
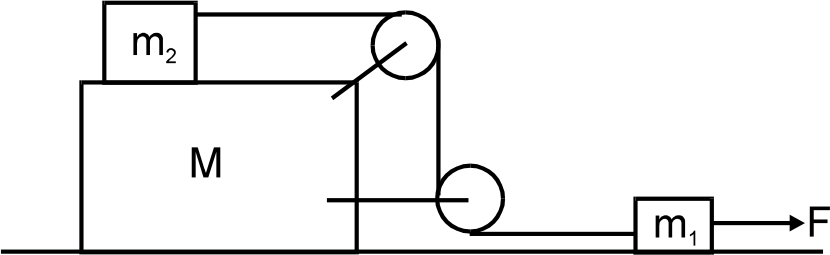
(P)Accleration of mass m1(1)Fm1(Q)Acceleration of massm2(2)Fm1+m2(R)Acceleration of mass M(3)zero(S)Tension in the string(4)m2Fm1+m2
View SolutionIn the situation shown in figure, both the pulleys and the strings are light and all the surfaces are frictionless. The acceleration of mass M, tension in the string PQ and force exerted by the clamp on the pulley, will respectively be -
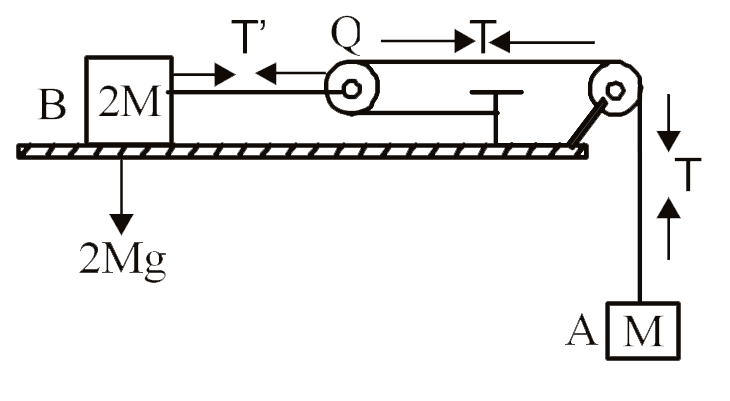
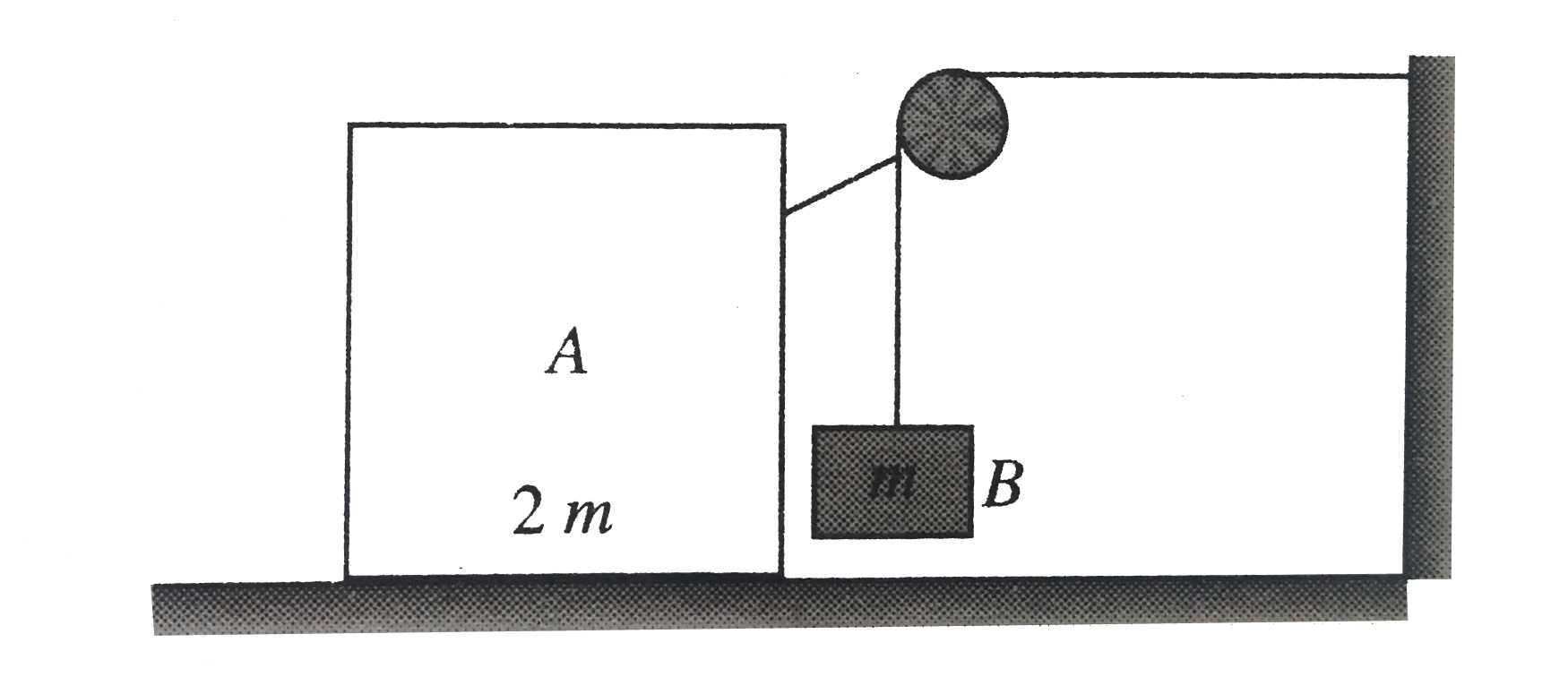
View SolutionIn the system shown all the surfaces are frictionless while pulley and string are massless. The mass of block A is 2m and that of block B is m. The acceleration of block B immediately after system is released from rest is
View SolutionThere blocks a,b and c of massses 10 kg ,10 kg and 20 kg respectivaly are arrenged as shown in figure all the surface are frictionless and string is inextensible A constant force F= 20N is applied on block a as shown . pulley and string are light . part of the string connecting both pulleys is vertical and part of the string connecting pulleys with masses a and b are horizontal .
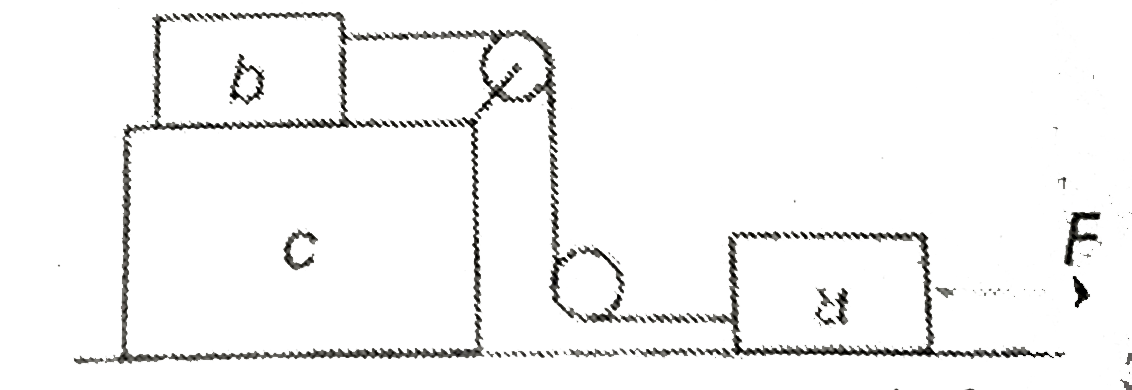
View SolutionFigure shows an arrangement of pulleys and two blocks. All surfaces are frictionless . All pulleys and strings are massless. All string are smooth and massless.
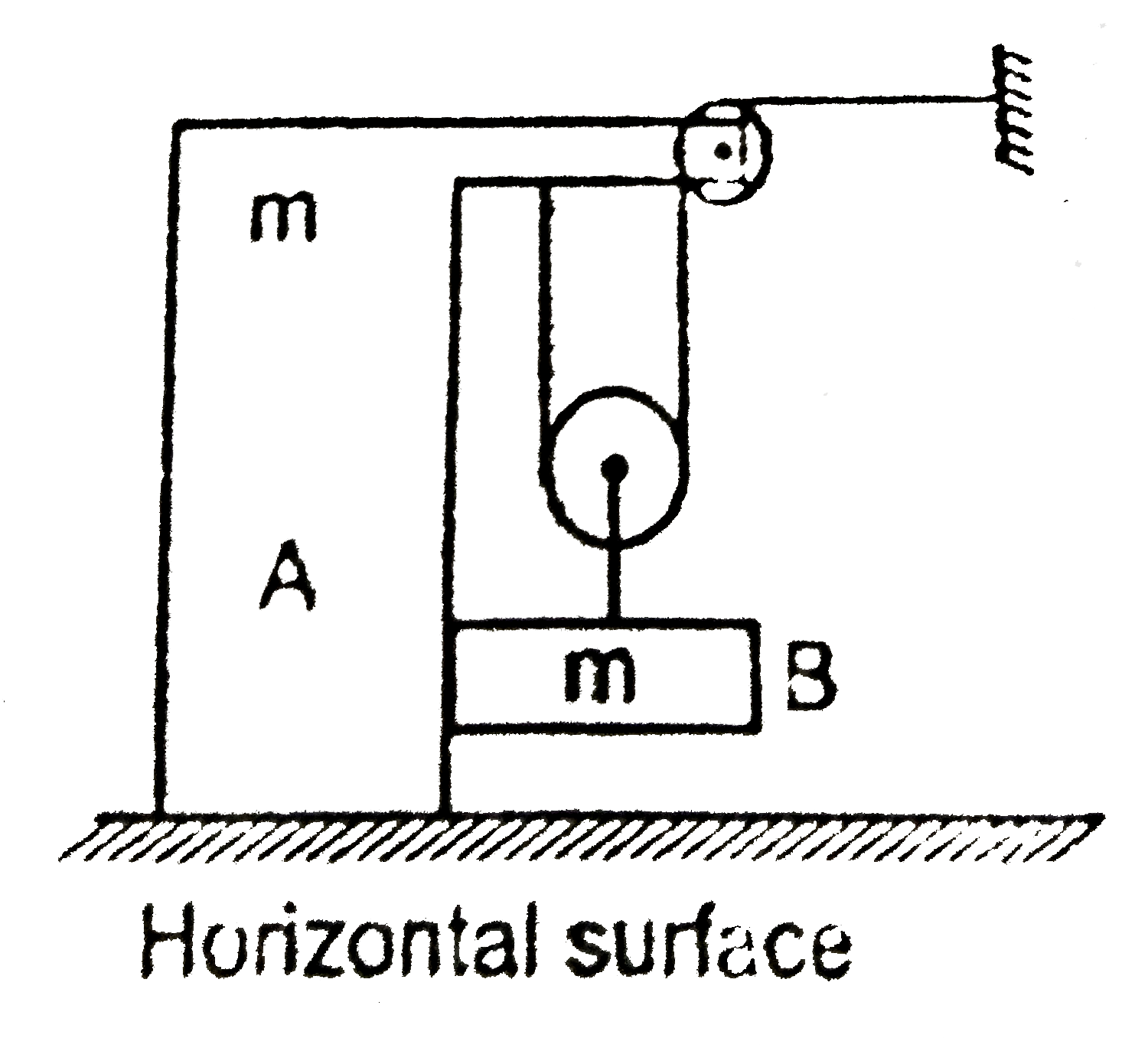
The acceleration of block A is :
View SolutionFigure shows an arrangement of pulleys and two blocks. All surfaces are frictionless . All pulleys and strings are massless. All string are smooth and massless.
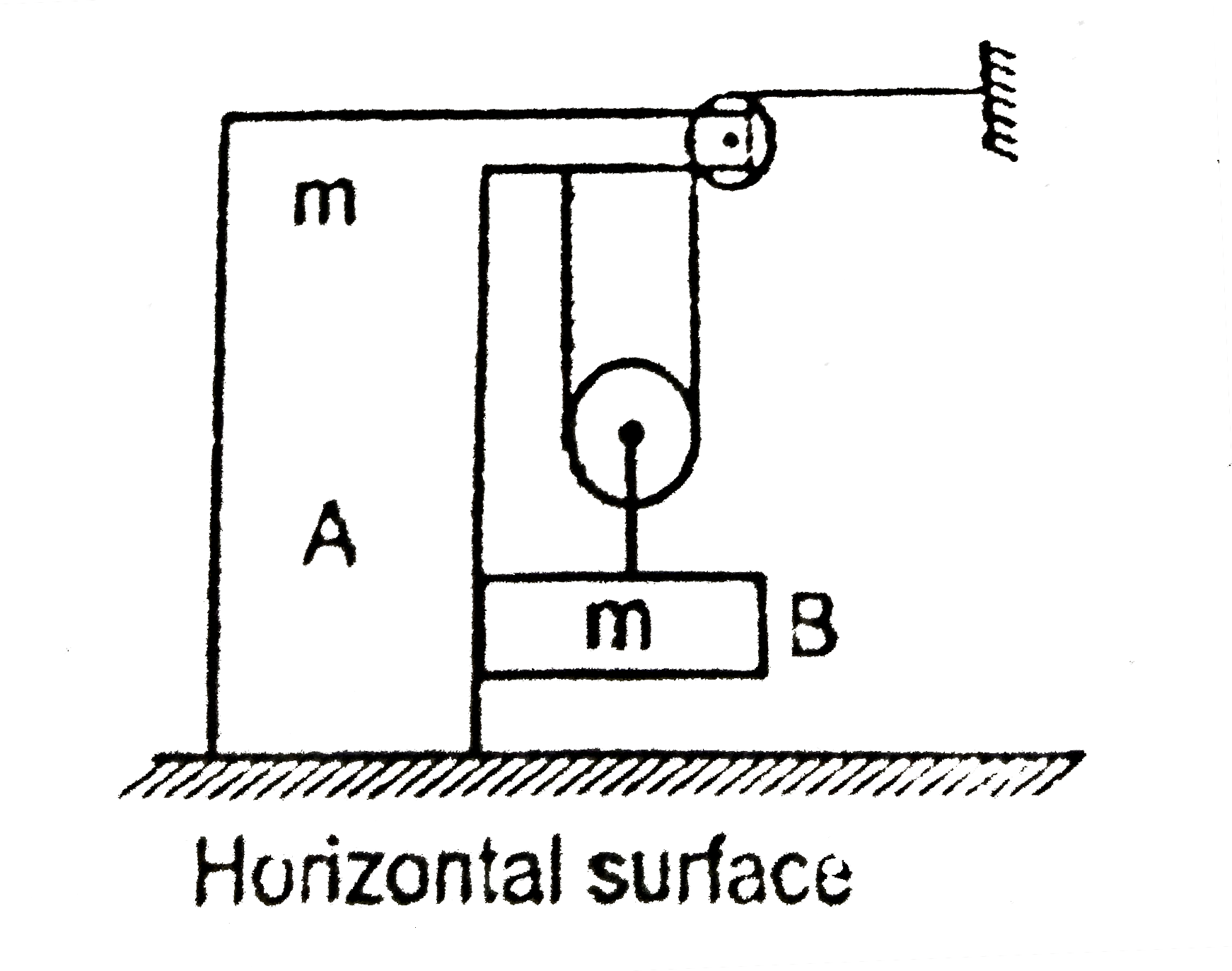
Normal reaction between A and ground is :
View SolutionConsider the situation shown in figure. Both the pulleys and the strings are light and all the surfaces are frictionless. Calculate
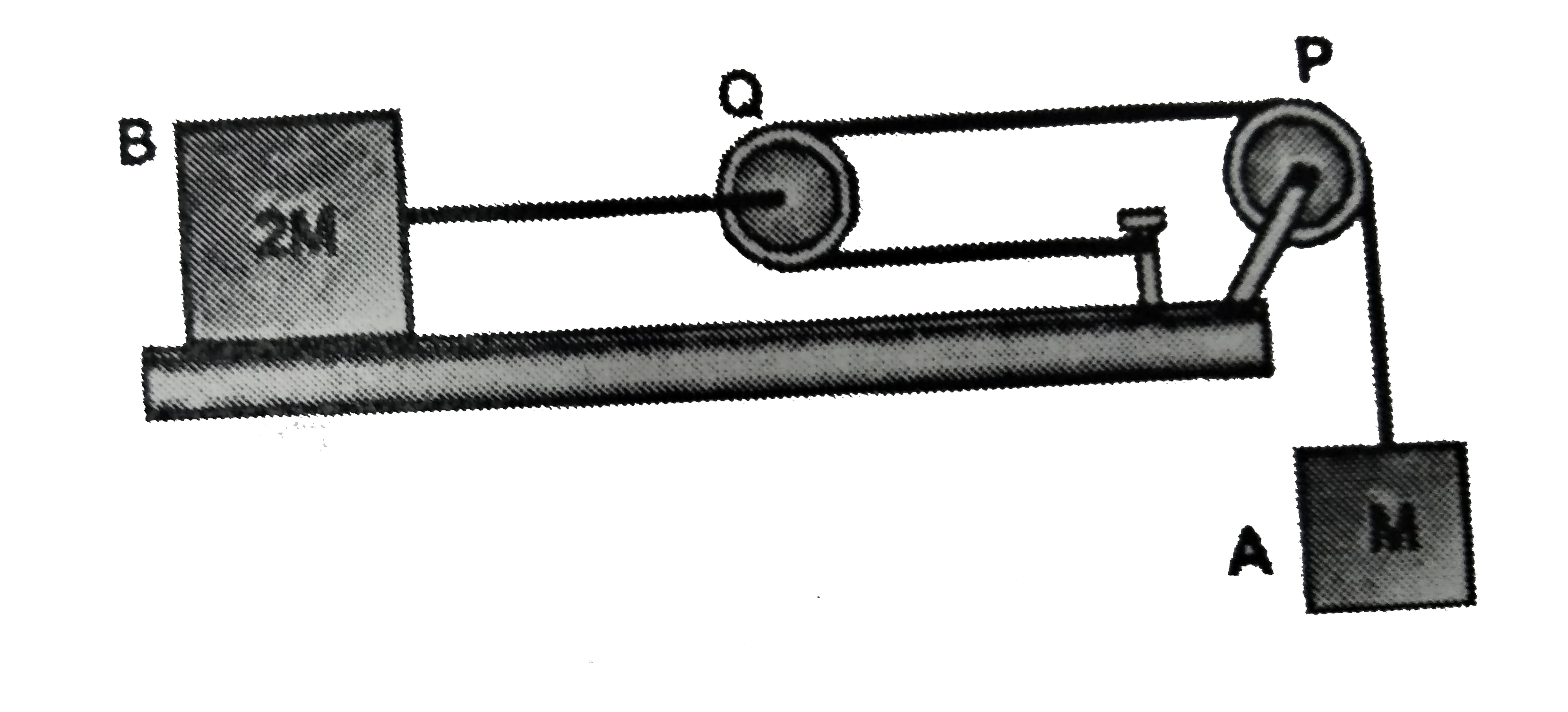
(a) the acceleration of mass M,
(b) tension in the string PQ and
(c ) force exerted by the pulley P.
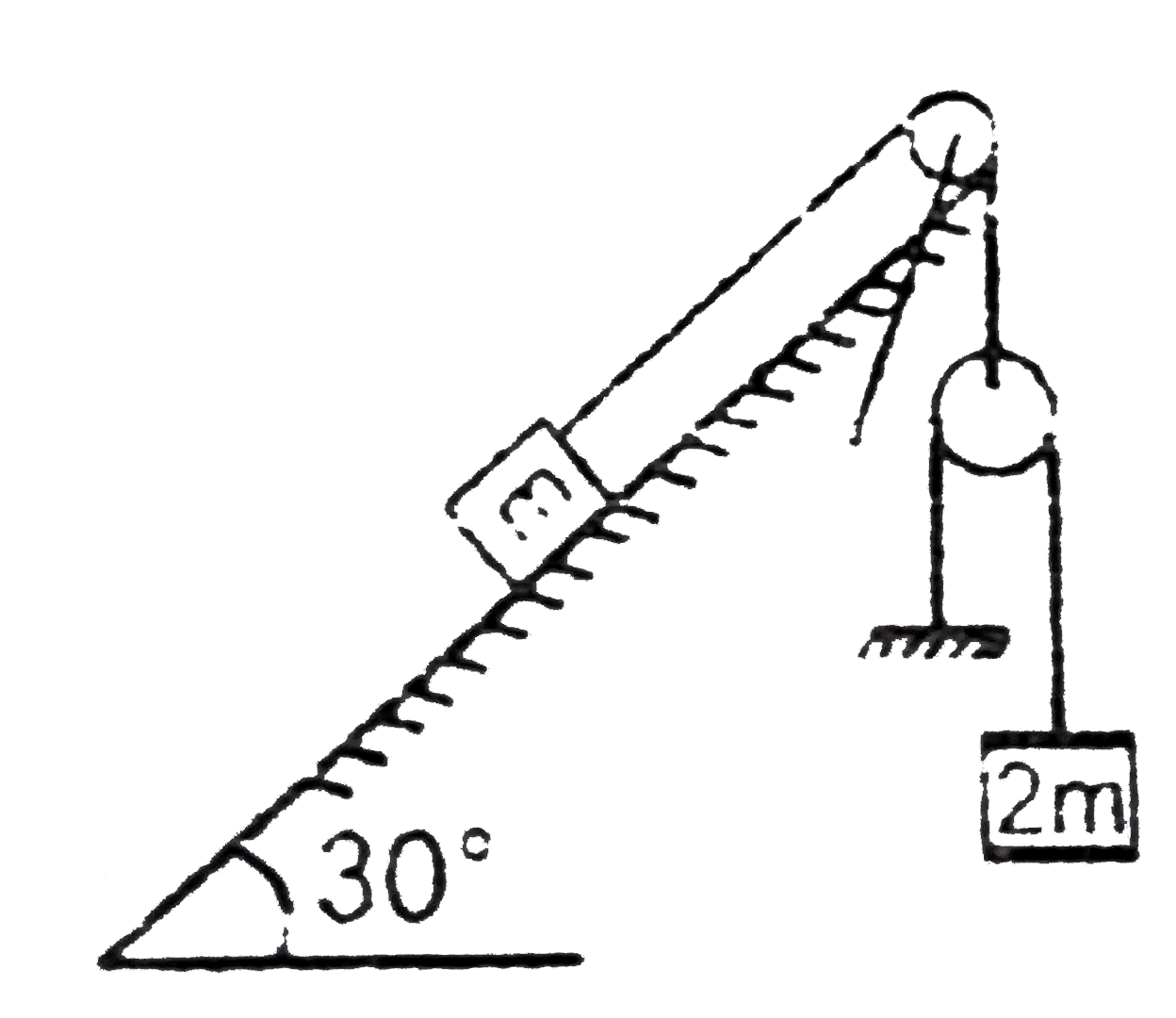
View SolutionIn the given figure inclined surface and pulleys are smooth. Strings and pulleys are massless. Acceleration of mass m is :
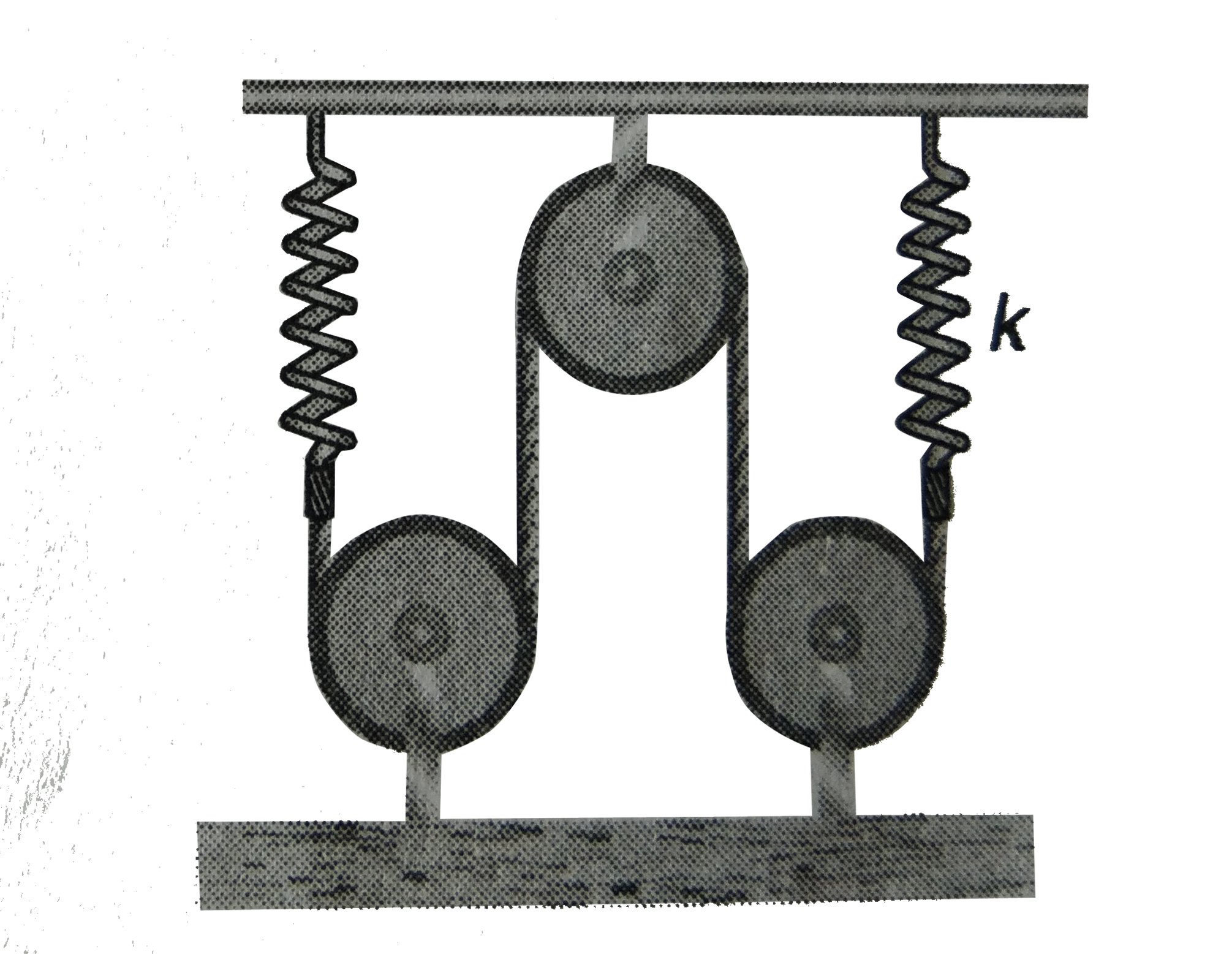
View SolutionFind the natural frequency of the system shown in figure. The pulleys are smooth and massless.
View Solution
