A disc of mass 3 m and a disc of mass m are connected by a massless spring of stiffness k. The heavier disc is placed on the ground with the spring vertical and lighter disc on top from its equilibrium position the upper disc is pushed down by a distance δ and released. Then.
if δ>3mgk the lower disc will bounce up.
if δ=2mgk maximum normal reaction on lower disc = 6mg .
if δ=2mgk maximum normal reaction from ground on lower disc = 4 mg.
if δ>4mgk, the lower disc will bounce up
The correct Answer is:B, D
To solve the problem step by step, we will analyze the system of two discs connected by a spring and determine the conditions under which the lower disc will bounce up, as well as the maximum and minimum normal reactions acting on the lower disc.
Step 1: Understand the System
We have two discs:
- A heavier disc of mass 3m placed on the ground.
- A lighter disc of mass m placed on top of the heavier disc, connected by a spring of stiffness k.
Step 2: Determine the Equilibrium Position
In the equilibrium position, the weight of the upper disc (mg) is balanced by the spring force. Let the compression of the spring at equilibrium be δ0.
Using Hooke's Law:
Fspring=kδ0=mg
From this, we can find the equilibrium compression:
δ0=mgk
Step 3: Analyze the Initial Displacement
The upper disc is pushed down by a distance δ from its equilibrium position and then released. The total compression of the spring when the upper disc is pushed down is:
x=δ0+δ=mgk+δ
Step 4: Condition for Lower Disc to Bounce Up
The lower disc will bounce up if the force exerted by the spring exceeds the weight of the lower disc. The force exerted by the spring when compressed by x is:
Fspring=kx=k(mgk+δ)=mg+kδ
For the lower disc to bounce up:
Fspring>3mg
This leads to the condition:
mg+kδ>3mg⟹kδ>2mg⟹δ>2mgk
Step 5: Maximum Normal Reaction on the Lower Disc
If δ=2mgk, we can calculate the maximum normal reaction (Nmax) on the lower disc. The total downward force on the lower disc is:
Fdown=3mg+Fspring=3mg+3mg=6mg
Thus, the maximum normal reaction is:
Nmax=6mg
Step 6: Minimum Normal Reaction on the Lower Disc
If δ=2mgk, we can also find the minimum normal reaction. The compression of the spring at this point is:
x=mgk+2mgk=3mgk
The force exerted by the spring is:
Fspring=kx=3mg
The minimum normal reaction occurs when the spring force just balances the weight of the upper disc:
Nmin=3mg−mg=2mg
Step 7: Condition for Lower Disc to Bounce Up Again
If δ>4mgk, we can analyze the forces again. The spring force must be equal to or greater than the weight of the lower disc for it to bounce up:
Fspring=3mg
Thus, the condition is:
kδ>3mg⟹δ>3mgk
Conclusion
- The lower disc will bounce up if δ>3mgk.
- The maximum normal reaction on the lower disc when δ=2mgk is 6mg.
- The minimum normal reaction on the lower disc when δ=2mgk is 2mg.
- The lower disc will bounce up again if δ>4mgk.
We have two discs:
- A heavier disc of mass 3m placed on the ground.
- A lighter disc of mass m placed on top of the heavier disc, connected by a spring of stiffness k.
In the equilibrium position, the weight of the upper disc (mg) is balanced by the spring force. Let the compression of the spring at equilibrium be δ0.
Fspring=kδ0=mg
From this, we can find the equilibrium compression:
δ0=mgk
The upper disc is pushed down by a distance δ from its equilibrium position and then released. The total compression of the spring when the upper disc is pushed down is:
x=δ0+δ=mgk+δ
The lower disc will bounce up if the force exerted by the spring exceeds the weight of the lower disc. The force exerted by the spring when compressed by x is:
Fspring=kx=k(mgk+δ)=mg+kδ
For the lower disc to bounce up:
Fspring>3mg
This leads to the condition:
mg+kδ>3mg⟹kδ>2mg⟹δ>2mgk
If δ=2mgk, we can calculate the maximum normal reaction (Nmax) on the lower disc. The total downward force on the lower disc is:
Fdown=3mg+Fspring=3mg+3mg=6mg
Thus, the maximum normal reaction is:
Nmax=6mg
If δ=2mgk, we can also find the minimum normal reaction. The compression of the spring at this point is:
x=mgk+2mgk=3mgk
The force exerted by the spring is:
Fspring=kx=3mg
The minimum normal reaction occurs when the spring force just balances the weight of the upper disc:
Nmin=3mg−mg=2mg
If δ>4mgk, we can analyze the forces again. The spring force must be equal to or greater than the weight of the lower disc for it to bounce up:
Fspring=3mg
Thus, the condition is:
kδ>3mg⟹δ>3mgk
- The lower disc will bounce up if δ>3mgk.
- The maximum normal reaction on the lower disc when δ=2mgk is 6mg.
- The minimum normal reaction on the lower disc when δ=2mgk is 2mg.
- The lower disc will bounce up again if δ>4mgk.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Subjective15videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Objective30videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question11videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
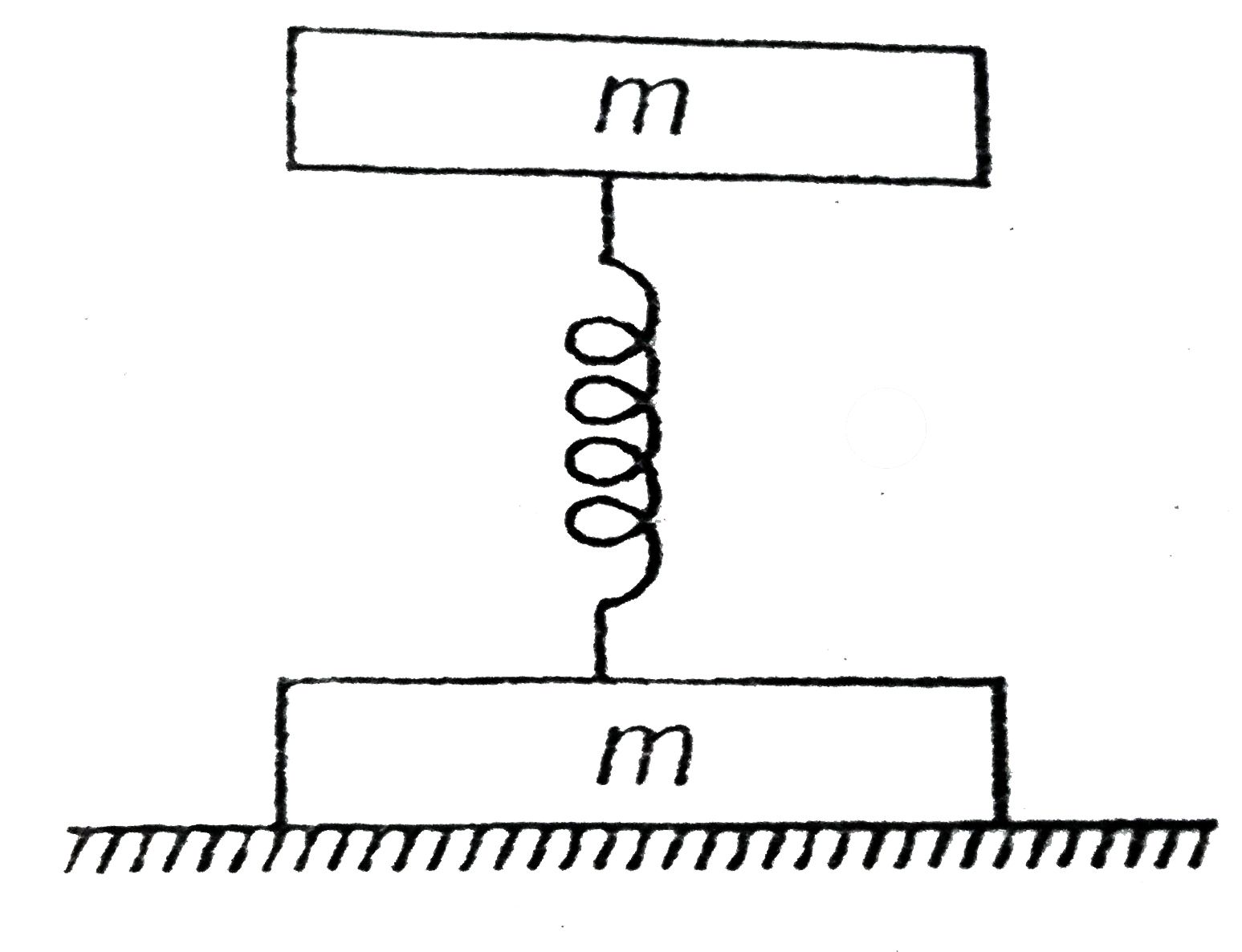
Figure shows two discs of same mass m. They are rigidy attached to a spring of stiffness k. The system is in equlibrium. From this equilibrium position, the upper disc is pressed down slowly by a distance x and released. Find the minimum value of x, if the lower disc is just lifted off the ground.
 View Solution
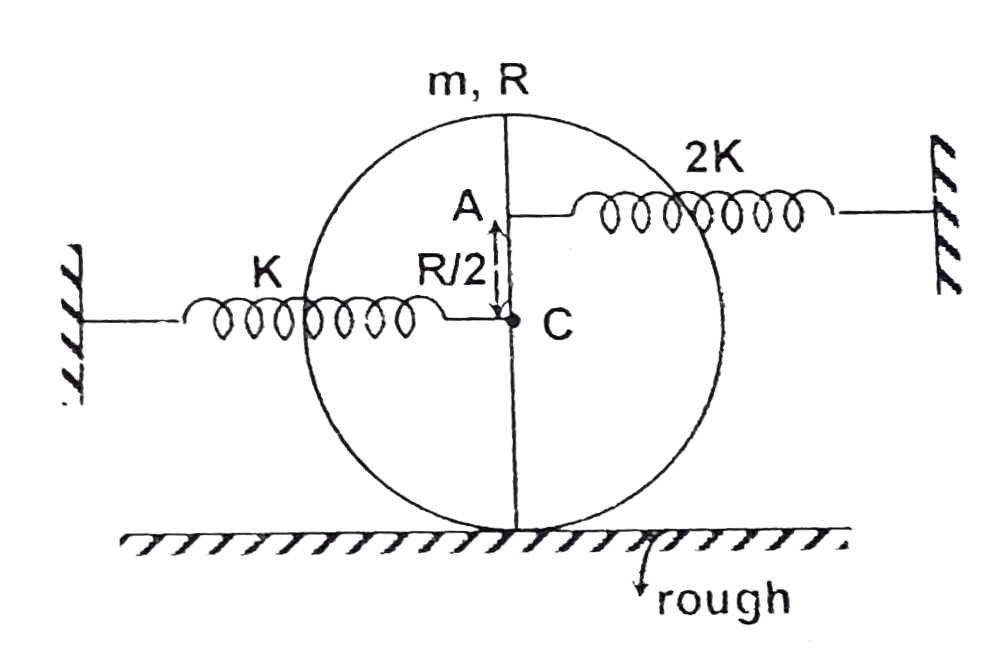
View SolutionA uniform circular disc of mass 'm' and radius 'R' is placed on a rough horizontal surface and connected to the two ideal non-deformed spring of stiffness k and 2k at the centre 'C' and point 'A' as shown . The centre of the disc is slighty displaced horizontally from equilibrium positon and then released, then the time period of small oscillation of the disc is (there is no slipping between the disc and the surface).
 View Solution
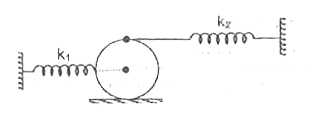
View SolutionA disk of mass m is connected to two springs of stiffness k1andk2 as shown in the figure. Find the angular frequency of the system for small oscillation. Disc can roll on the surface without slipping
 View Solution
View SolutionA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

Force of friction between the disc and the plank isView SolutionOne end of spring of spring constant k is attached to the centre of a disc of mass m and radius R and the other end of the spring connected to a rigid wall. A string is wrapped on the disc and the end A of the string (as shown in the figure) is pulled through a distance a and then released.
The disc is placed on a horizontal rough surface and there is no slipping at any contact point. What is the amplitude of the oscillation of the centre of the disc?
 View Solution
View SolutionA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

Acceleration of the plank isView SolutionA disc of mass 5 kg and radius 50 cm rolls on the ground at the rate of 10ms−1. Calculate the K.E. of the disc.
View Solution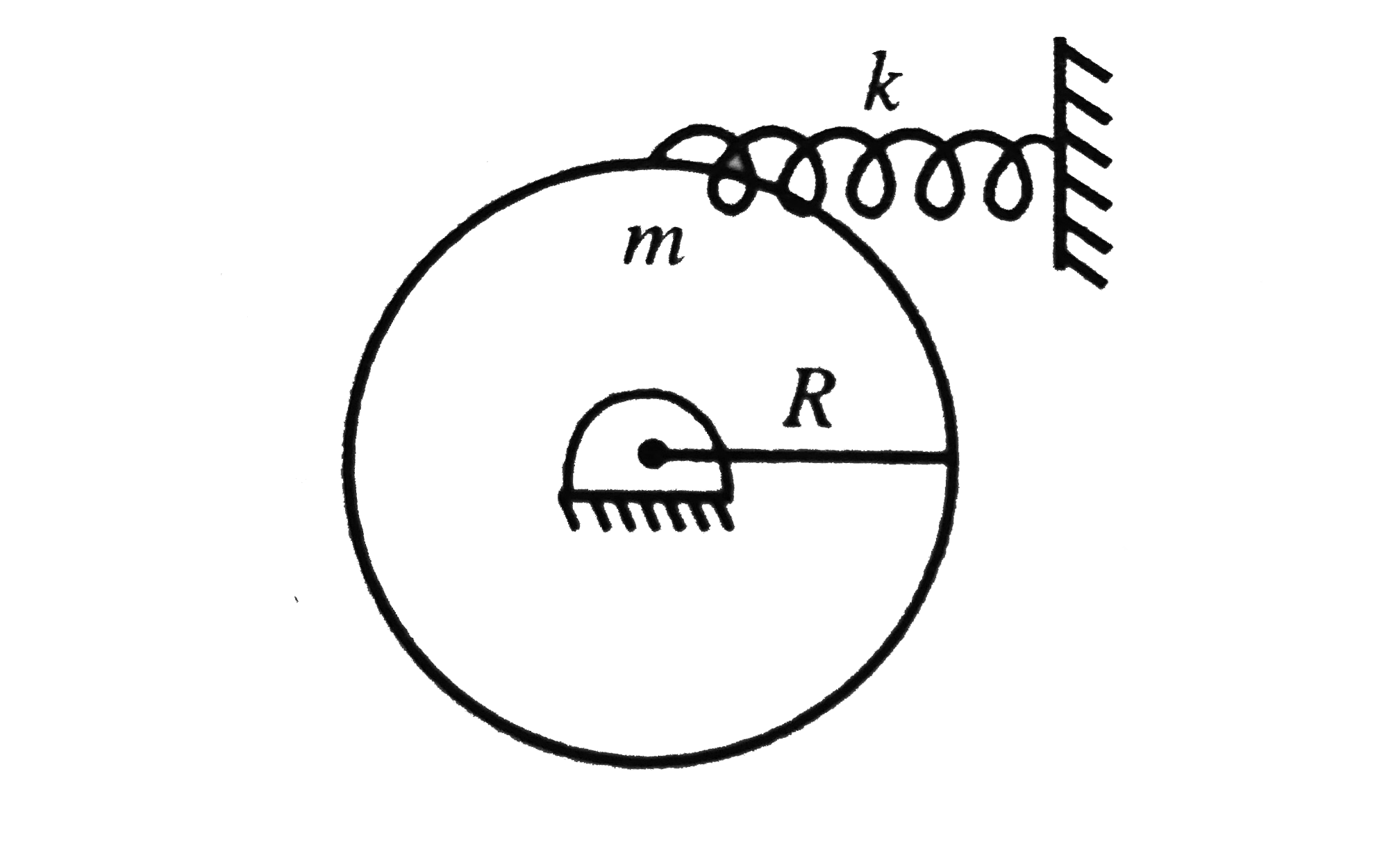
A uniform disc of mass m and radius R is pivoted smoothly at its centre of mass. A light spring of stiffness k is attached with the dics tangentially as shown in the Fig. Find the angular frequency in rads of torsional oscillation of the disc. (Take m=5kg and K=10Nm.)View Solution
A uniform disc of mass m and radius R is pivoted smoothly at its centre of mass. A light spring of stiffness k is attached with the dics tangentially as shown in the Fig. Find the angular frequency in rads of torsional oscillation of the disc. (Take m=5kg and K=10Nm.)View SolutionA uniform disc of mass M and radius R is mounted on a fixed horizontal axis. A block of mass m hangs from a massless string that is wrapped around the rim of the disc. The magnitude of the acceleration of the falling block (m) is
View Solution

