
A ball of mass m and radius r rolls along a circular path of radius R its speed at the bottom (θ=0∘) of the path is v0 find the force of the path on the ball as a function of θ.

h=(R−r)(1−cosθ)
K=75(12mv20)−mgh
∵ In case of pure rolling
KT=57K
∴12mv2=12mv20−57mgh
∴v2=v20−107gh ...(ii)
Equation of motion at angle θ is
N−mgcosθ=mv2(R−r)
∴N=mgcosθ=m(R−r)(v20−107gh)
Subsituting value of h from eq. (i)
N=mgcosθ+(mR−r)
{v20−107g(R−r)(1−cosθ)}
=mg7(17cosθ−10)+mv20R−r)
Force of friction,
f=mgsinθ1+mr2I (for pure rolling to take place)
=mgsinθ1+52 (I=25mr2)
=27mgsinθ
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Level 21videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions17videos
ROTATIONAL MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
A particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B
 AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
A satellite of mass m goes round the earth along a circular path of radius r. Let mE be the mass of the earth and RE its radius then the linear speed of the satellite depends on.
View SolutionA body of mass 100 g is rotating in a circular path of radius r with constant speed. The work done in one complete revolution is
View SolutionA ball of mass m and radius r rolls inside a hemispherical shell of radius R. It is released from rest from point A as shown in figure. The angular velocity of centre of the ball in position B about the centre of the shell is.
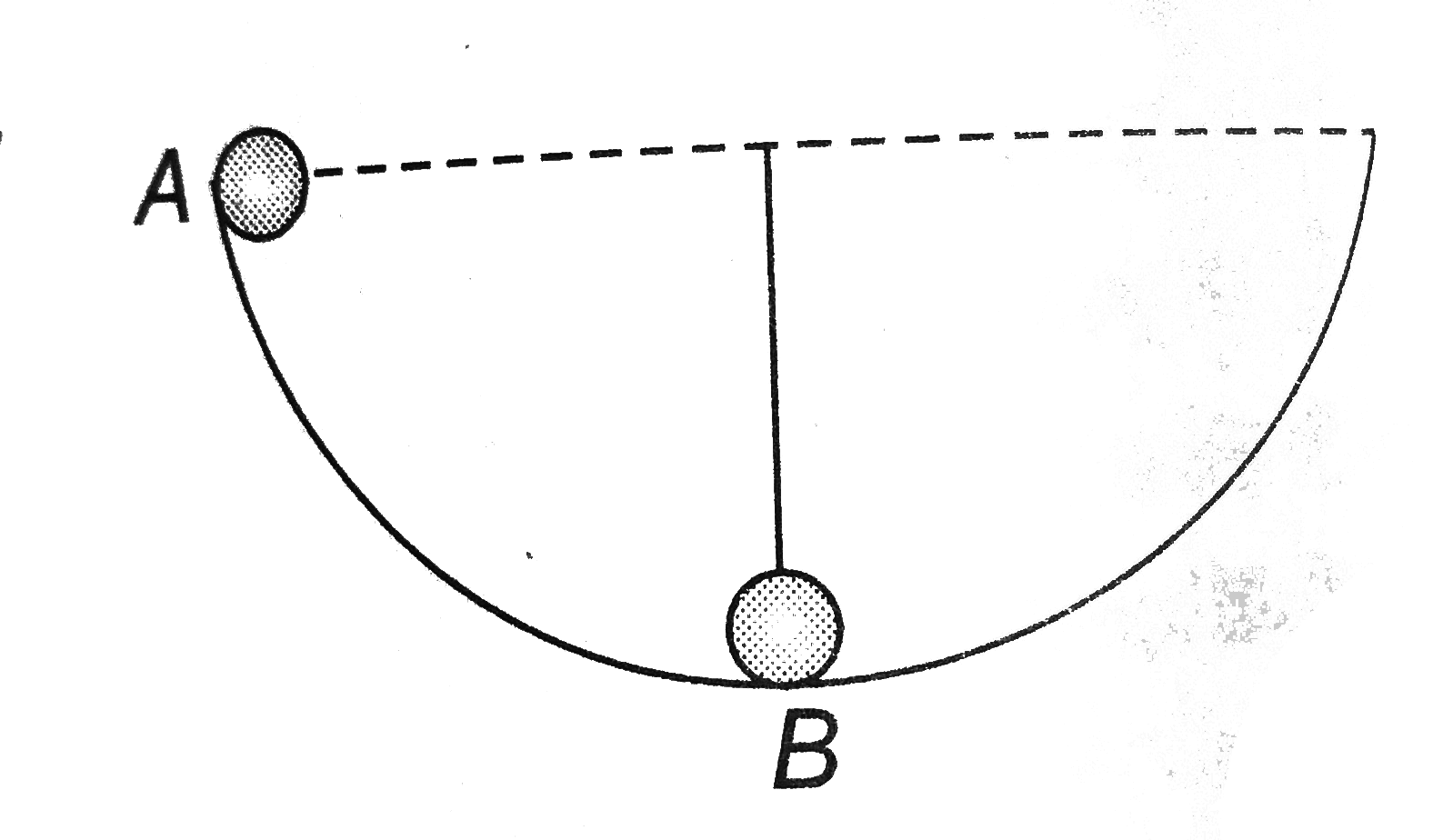 .View Solution
.View SolutionA Particle of mass 'M' moves in a uniform circular path of radius 'r' with a constant speed 'v' then its centripetal acceleration is .
View SolutionA particle of mass 2 kg is moving along a circular path of radius 1 m. If its angular speed is 2π rad s−1, the centripetal force on it is
View SolutionA car of mass 1000 kg is moving with a speed of 40ms−1 on a circular path of radius 400m. If its speed is increasing at the rate of 3ms−2 the total force acting on the car is
View SolutionA particle of mass M moves with constant speed along a circular path of radius r under the action of a force F. Its speed is
View Solution

