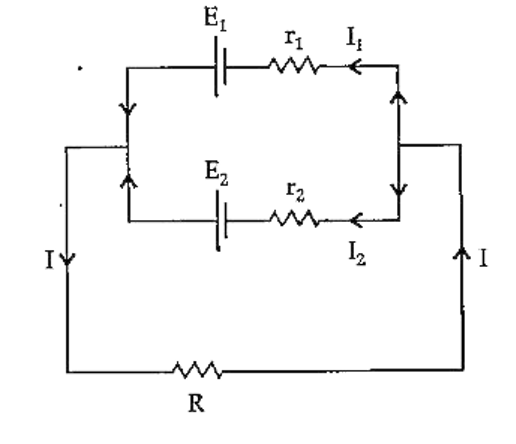
Two cells of emf E1=6V and E2=5 are joined in parallel with same polarity on same side without any external load. If their internal resistance are r1=2Ω and r2=3Ω respectively, then
terminal potentiasl difference across any cell is less than 5V
terminal potential differences any cell is 5.6V
current through the cells is 0.2A
current through the cells is zero if E1=E2
The correct Answer is:B, C, D
To solve the problem step by step, we will use Kirchhoff's voltage law (KVL) and the concepts of internal resistance in parallel circuits.
Step 1: Understand the Circuit Configuration
We have two cells connected in parallel:
- Cell 1 with emf E1=6V and internal resistance r1=2Ω
- Cell 2 with emf E2=5V and internal resistance r2=3Ω
Step 2: Apply Kirchhoff's Voltage Law (KVL)
To find the current flowing through the circuit, we can apply KVL to the loop formed by the two cells. We assume a current I flows through the circuit.
Starting from one point in the loop (let's call it point A) and going around the loop, we can write the KVL equation:
E1−Ir1−E2−Ir2=0
This simplifies to:
E1−E2=I(r1+r2)
Step 3: Substitute the Values
Substituting the given values into the equation:
6V−5V=I(2Ω+3Ω)
1V=I(5Ω)
Step 4: Solve for Current I
Now, we can solve for I:
I=1V5Ω=0.2A
Step 5: Calculate Terminal Potential Difference for Cell 1
To find the terminal potential difference across cell 1, we can use the formula:
VAB=E1−Ir1
Substituting the values:
VAB=6V−(0.2A×2Ω)=6V−0.4V=5.6V
Step 6: Calculate Terminal Potential Difference for Cell 2
Similarly, for cell 2, we can use:
VCD=E2+Ir2
Substituting the values:
VCD=5V+(0.2A×3Ω)=5V+0.6V=5.6V
Summary of Results
- Current flowing through the circuit I=0.2A
- Terminal potential difference across cell 1 VAB=5.6V
- Terminal potential difference across cell 2 VCD=5.6V
We have two cells connected in parallel:
- Cell 1 with emf E1=6V and internal resistance r1=2Ω
- Cell 2 with emf E2=5V and internal resistance r2=3Ω
To find the current flowing through the circuit, we can apply KVL to the loop formed by the two cells. We assume a current I flows through the circuit.
E1−Ir1−E2−Ir2=0
This simplifies to:
E1−E2=I(r1+r2)
Substituting the given values into the equation:
6V−5V=I(2Ω+3Ω)
1V=I(5Ω)
Now, we can solve for I:
I=1V5Ω=0.2A
To find the terminal potential difference across cell 1, we can use the formula:
VAB=E1−Ir1
Substituting the values:
VAB=6V−(0.2A×2Ω)=6V−0.4V=5.6V
Similarly, for cell 2, we can use:
VCD=E2+Ir2
Substituting the values:
VCD=5V+(0.2A×3Ω)=5V+0.6V=5.6V
- Current flowing through the circuit I=0.2A
- Terminal potential difference across cell 1 VAB=5.6V
- Terminal potential difference across cell 2 VCD=5.6V
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 2 Comprehension Example Type
2 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 2 Subjective
13 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 2 Single Correct
26 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
Two batteries with emf 12 V and 13V are connected in parallel across a load resistor of 10Ω . The internal resistances of the two batteries are 1Ωand2Ω respectively . The voltage across the load lies between
View SolutionTwo cells having emf's of 10 V and 8 V, respectively, are connected in series with a resistance of 24Ω In the external circuit. Their internal resistances are 2Ω/V (proportional to their emf's). Find the current in the circuit

View SolutionTwo electric bulbs , rated for the same voltage , have powers of 200Wand100W, respectively. If their resistances are r1andr2 , respectively , then
View SolutionIn the circuit shown E,F,G and H are cells of emf 2V,1V,3V and 1V respectively and their internal resistance are 2Ω,1Ω,3Ω and 1Ω respectively.
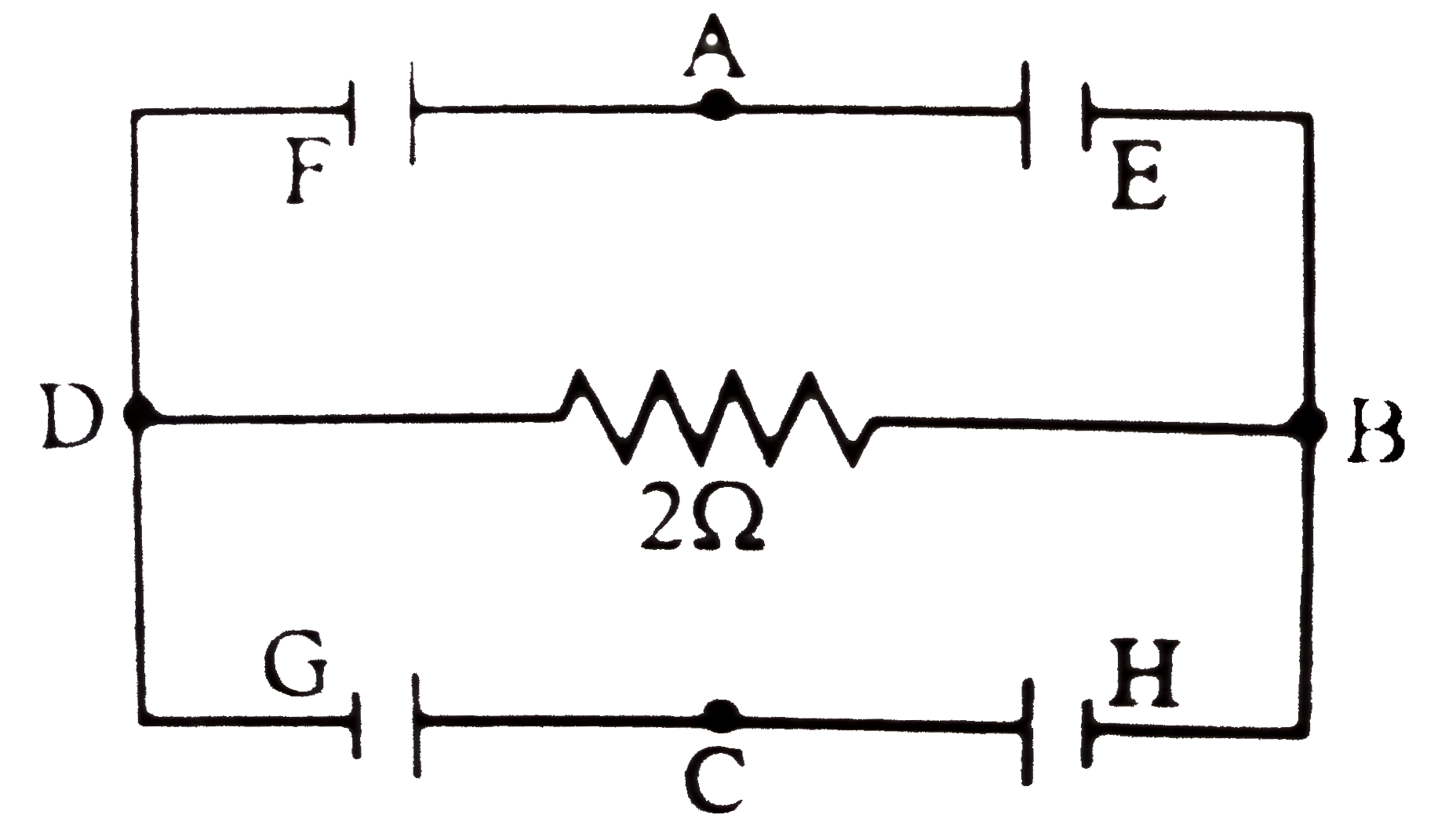
View SolutionIn the circuit shown E,F,G and H are cells of emf 2V,1V,3V and 1V respectively and their internal resistance are 2Ω,1Ω,3Ω and 1Ω respectively.

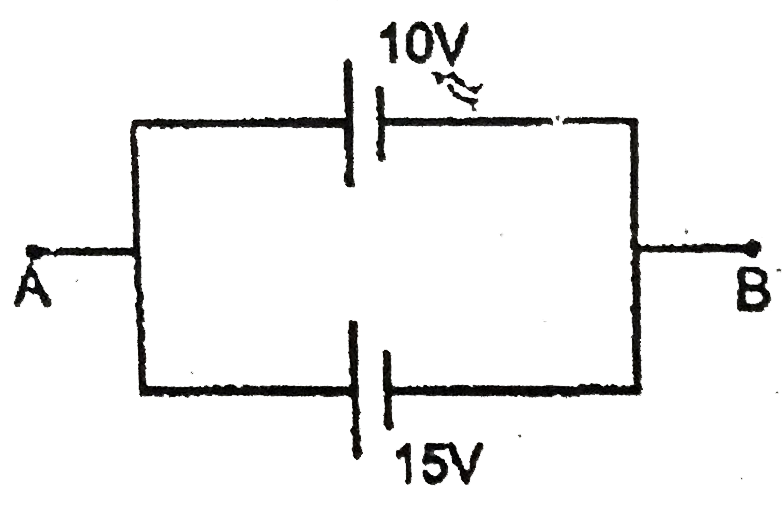
View SolutionTwo cells of e.m.f. 10V & 15V are connected in parallel to each other between points A&B. The cell of e.m.f. 10V is ideal but the cell of e.m.f. 15V has internal resistance 1Ω. The equivalent e.m.f. between A and B is:
View SolutionTwo cells, having the same emf, are connected in series through an external resistance R. Cells have internal resistance r1 and r2(r1>r2) respectively. When the circuit is closed, the potentail difference across the first cell is zero the value of R is
View SolutionTwo cells, having the same emf, are connected in series through an external resistance R. Cells have internal resistance r1 and r2(r1>r2) respectively. When the circuit is closed, the potential difference across the first cell is zero the value of R is
View SolutionTwo cells, having the same emf, are connected in series through an external resistance R. Cells have internal resistance r1 and r2(r1>r2) respectively. When the circuit is closed, the potentail difference across the first cell is zero the value of R is
View SolutionTwo cells of emf E1 and E2 have internal resistance r1 and r2. Deduce an expression for equivalent emf of their parallel combination.
View Solution