Two identical conducting spheres, fixed in space, attract each other with an electrostatic force of 0.108N when separated by 50.0cm, centre-to-centre. A thin conducting wire then connects the spheres. When the wire is removed, the spheres repel each other with an electrostatic force of 0.0360N. What were the initial charges on the spheres?
The correct Answer is:A, C
To solve the problem step by step, we will use Coulomb's law and the principle of conservation of charge.
Step 1: Understand the Initial Attraction
We know that two identical conducting spheres attract each other with an electrostatic force of F1=0.108N when separated by a distance r=50.0cm=0.5m. Let the charges on the spheres be Q1 and Q2. According to Coulomb's law, the force between two charges is given by:
F=k|Q1Q2|r2
Where k is Coulomb's constant, approximately 8.99×109N m2/C2.
Step 2: Set Up the Equation for Initial Attraction
Substituting the known values into Coulomb's law:
0.108=k|Q1Q2|(0.5)2
This simplifies to:
0.108=k|Q1Q2|0.25
Rearranging gives:
|Q1Q2|=0.108×0.25/k
Step 3: Calculate Q1Q2
Substituting k:
|Q1Q2|=0.108×0.258.99×109
Calculating this gives:
|Q1Q2|=0.0278.99×109≈3×10−12C2
Step 4: Understand the Effect of Shorting the Spheres
When the spheres are connected by a wire, they will share charge and reach the same potential. Let the new charge on each sphere be Q′. The total charge is conserved:
Q′+Q′=Q1+Q2⟹2Q′=Q1+Q2⟹Q′=Q1+Q22
Step 5: Set Up the Equation for Repulsion After Shorting
After the wire is removed, the spheres repel each other with a force of F2=0.0360N. Using Coulomb's law again:
0.0360=k(Q′)2(0.5)2
This simplifies to:
0.0360=k(Q′)20.25
Rearranging gives:
(Q′)2=0.0360×0.25/k
Step 6: Calculate (Q′)2
Substituting k:
(Q′)2=0.0360×0.258.99×109
Calculating this gives:
(Q′)2=0.0098.99×109≈1×10−12C2
Step 7: Solve for Q′
Taking the square root gives:
Q′=√1×10−12=1×10−6C
Step 8: Use Charge Conservation to Find Q1 and Q2
From the charge conservation equation:
Q1+Q2=2Q′=2×1×10−6=2×10−6C
Step 9: Solve the System of Equations
We have two equations:
1. |Q1Q2|=3×10−12
2. Q1+Q2=2×10−6
Let Q2=2×10−6−Q1 and substitute into the first equation:
|Q1(2×10−6−Q1)|=3×10−12
This is a quadratic equation in terms of Q1. Solving this gives:
Q1=3×10−6CandQ2=−1×10−6C
Final Result
The initial charges on the spheres are:
- Q1=3×10−6C
- Q2=−1×10−6C
We know that two identical conducting spheres attract each other with an electrostatic force of F1=0.108N when separated by a distance r=50.0cm=0.5m. Let the charges on the spheres be Q1 and Q2. According to Coulomb's law, the force between two charges is given by:
Substituting the known values into Coulomb's law:
Substituting k:
When the spheres are connected by a wire, they will share charge and reach the same potential. Let the new charge on each sphere be Q′. The total charge is conserved:
After the wire is removed, the spheres repel each other with a force of F2=0.0360N. Using Coulomb's law again:
Substituting k:
Taking the square root gives:
From the charge conservation equation:
We have two equations:
2. Q1+Q2=2×10−6
The initial charges on the spheres are:
- Q2=−1×10−6C
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Two positive charges separated by a distance 2m repel each other with a force of 0.36N. If the combined charge is 26μC, the charges are
View SolutionTwo identical particles are charged and held at a distance of 1m form each pther. They are found to be attraction each other with a force of 0.027N . Now they are connected by a conducting wire so that charge folws between them. Whe the charge flow stop, they are found to be repelling each other with a force of 0.009N. Find the initial charge on each particle.
View SolutionTwo identical conducting sphere carrying different charges attact each other with a force F when placed in air medium at a distance d apart. The spheres are brought into contact and then taken to their original positions. Now, the two sphere repel each other with a force whole magnitude is equal to the initial attractive force. The ratio between initial charges on the spheres is
View SolutionTwo identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
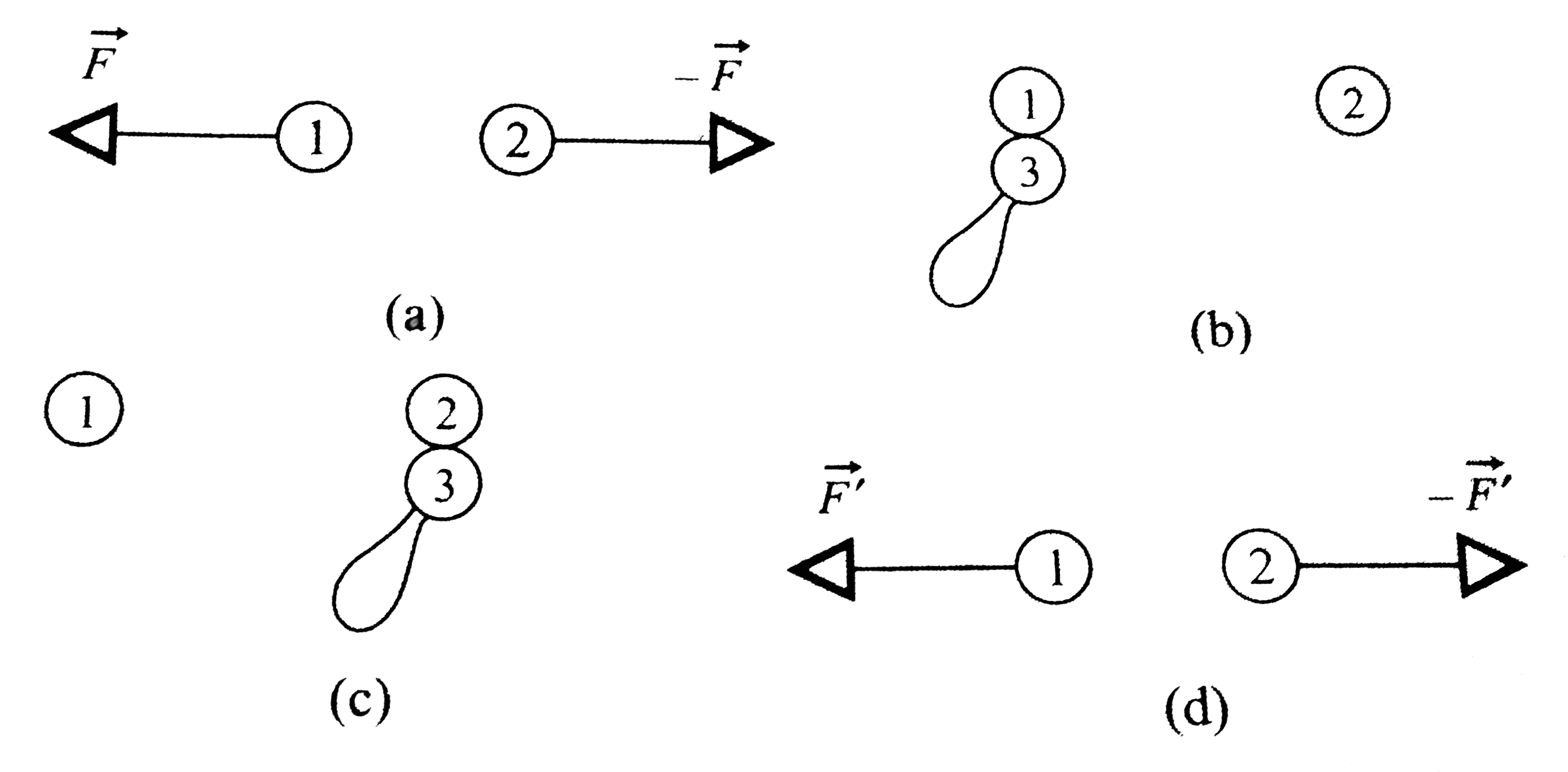 ,
,
View SolutionThe centres of two identical small conducting sphere are 1 m apart. They carry charge of opposite kind and attract each other with a force F. when they connected by conducting thin wire they repel each other with a force F/3. The ratio of magnitude of charges carried by the spheres initially in n:1. Find value of n
View SolutionThe centres of two identical small conducting spheres are 1m apart. They carry charge of opposite kind and attract each other with a force F. When they are connected by a conducting thin wire they repel each other with a force F/3. What is the ratio of magnitude of charge carried by the sphere initially ?
View SolutionTwo metallic spheres of radii a and b are placed faraway from each other and are connected for a thin conducting wire. A charge Q is given to one of the spheres . Calculate the charge on each sphere at electrostatic equilibrium.
View SolutionTwo conducting spheres of radii r and 2r are placed at very large separation. Each sphere possesses charge Q. These spheres are connected with a conducting wire of resistance R. Then, which of the following is true?
View SolutionTwo insulating small spheres are rubbed against each other and placed 1 cm apart. If they attract each other with a force of 0.1 N, how many electrons were transferred from one sphere to the other during rubbing?
View SolutionTwo identical conduction spheres, one having an initial charge +Q and the other initially uncharged, are brought into contact.
(a) What is the new charge on each sphere?
(b) While the spheres are in contact, a positively chaged rod is moved close to one sphere, causing a redistribution of hte charges on the two spheres so the charge on hte sphere closest to the rod has a charge-Q. What is the charge to the other sphere?
View Solution