In the relation F=q(V×B), which paiers are always perpendicular to each other.
To solve the question regarding the relation F=q(V×B) and to identify which pairs are always perpendicular to each other, we can follow these steps:
Step 1: Understand the Cross Product
The equation F=q(V×B) involves a cross product. By definition, the cross product of two vectors results in a third vector that is perpendicular to the plane formed by the original two vectors.
Step 2: Identify the Vectors
In this equation:
- V is the velocity vector of the charge.
- B is the magnetic field vector.
- F is the force experienced by the charge.
Step 3: Apply the Properties of the Cross Product
From the properties of the cross product, we know:
- The resulting vector F is perpendicular to both V and B.
Step 4: Conclusion
Thus, we can conclude that:
- The force F is perpendicular to the velocity V.
- The force F is also perpendicular to the magnetic field B.
- Therefore, the pairs that are always perpendicular to each other are:
- F and V
- F and B
Final Answer
In the relation F=q(V×B), the pairs that are always perpendicular to each other are:
- Force F is perpendicular to velocity V.
- Force F is perpendicular to magnetic field B.
---
The equation F=q(V×B) involves a cross product. By definition, the cross product of two vectors results in a third vector that is perpendicular to the plane formed by the original two vectors.
In this equation:
- V is the velocity vector of the charge.
- B is the magnetic field vector.
- F is the force experienced by the charge.
From the properties of the cross product, we know:
- The resulting vector F is perpendicular to both V and B.
Thus, we can conclude that:
- The force F is perpendicular to the velocity V.
- The force F is also perpendicular to the magnetic field B.
- Therefore, the pairs that are always perpendicular to each other are:
- F and V
- F and B
In the relation F=q(V×B), the pairs that are always perpendicular to each other are:
- Force F is perpendicular to velocity V.
- Force F is perpendicular to magnetic field B.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SCQ_TYPE5videos
MAGNETICS
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE1videos
MAGNETICS
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE1videos
MAGNETICS
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery59videos
MAGNETIC FIELD AND FORCES
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery1videos
MAGNETISM AND MATTER
Similar Questions
State the Lorenz's force and express it in vector form. Which pair of vectors are always perpendicular to each other? Derive the expression for the force acting on a current carrying conductor of length L in a uniform magnetic field 'B'.
View SolutionAn electromagnetic wave in vacuum has the electric and magnetic field →E and →B, which are always perpendicular to each other. The direction of polarization is given by →X and that of wave propagation by →K. Then
View Solution
are related to each other form?View SolutionStatement I: A light ray is incident on a glass slab. Some portion of it is reflected and some is refracted. Refracted and reflected rays are always perpendicular to each other.
Statement II: Angle of incidence is equal to angle of reflection.
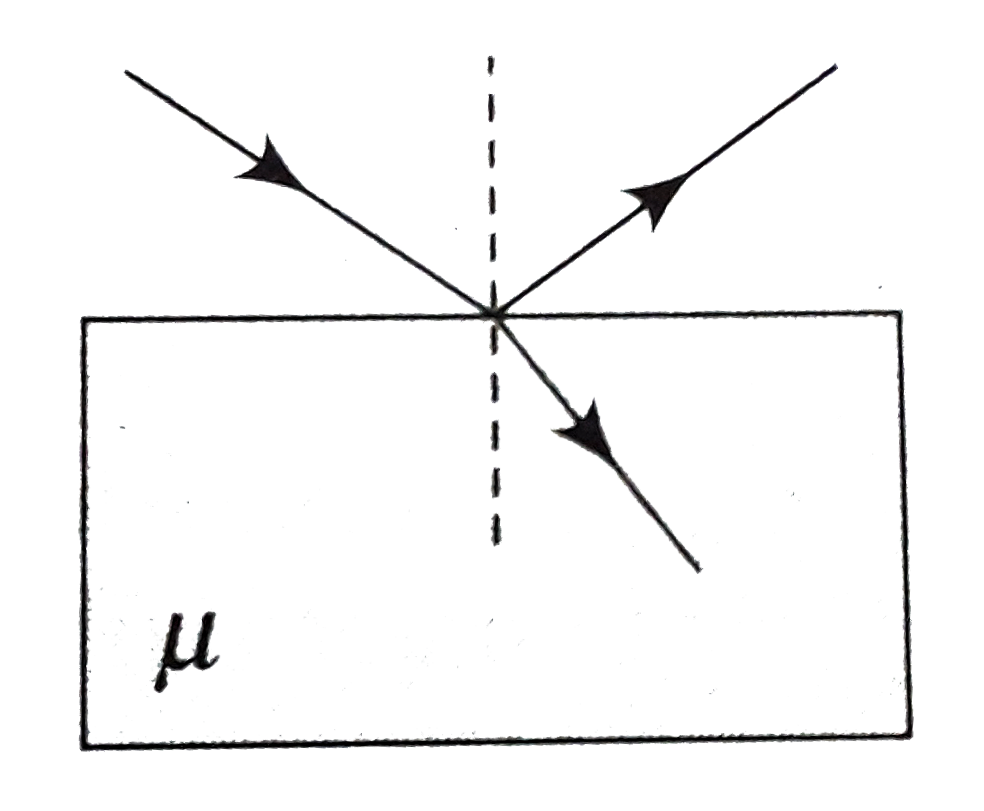 View Solution
View SolutionThe incident ray and the reflected ray from a mirror are mutually perpendicular to each other.
Find the angle of incidence.View SolutionIn periscope, the mirror surfaces are arranged perpendicular to each other.
View SolutionThree normals are drawn from the point (c, 0) to the curve y2=x. Show that c must be greater than 1/2. One normal is always the axis. Find c for which the other two normals are perpendicular to each other.
View SolutionTwo straight lines are cut by a transversal. If the bisectors of a pair of co-interior angles are perpendicular to each other , prove the two straight lines are parallel to each other.
View SolutionAn electric field (vec E) and a magnetic field (vec B)exist in a region . The fields are not perpendicular to each other.
View SolutionWhich bases are complimentary to each other?
View Solution

