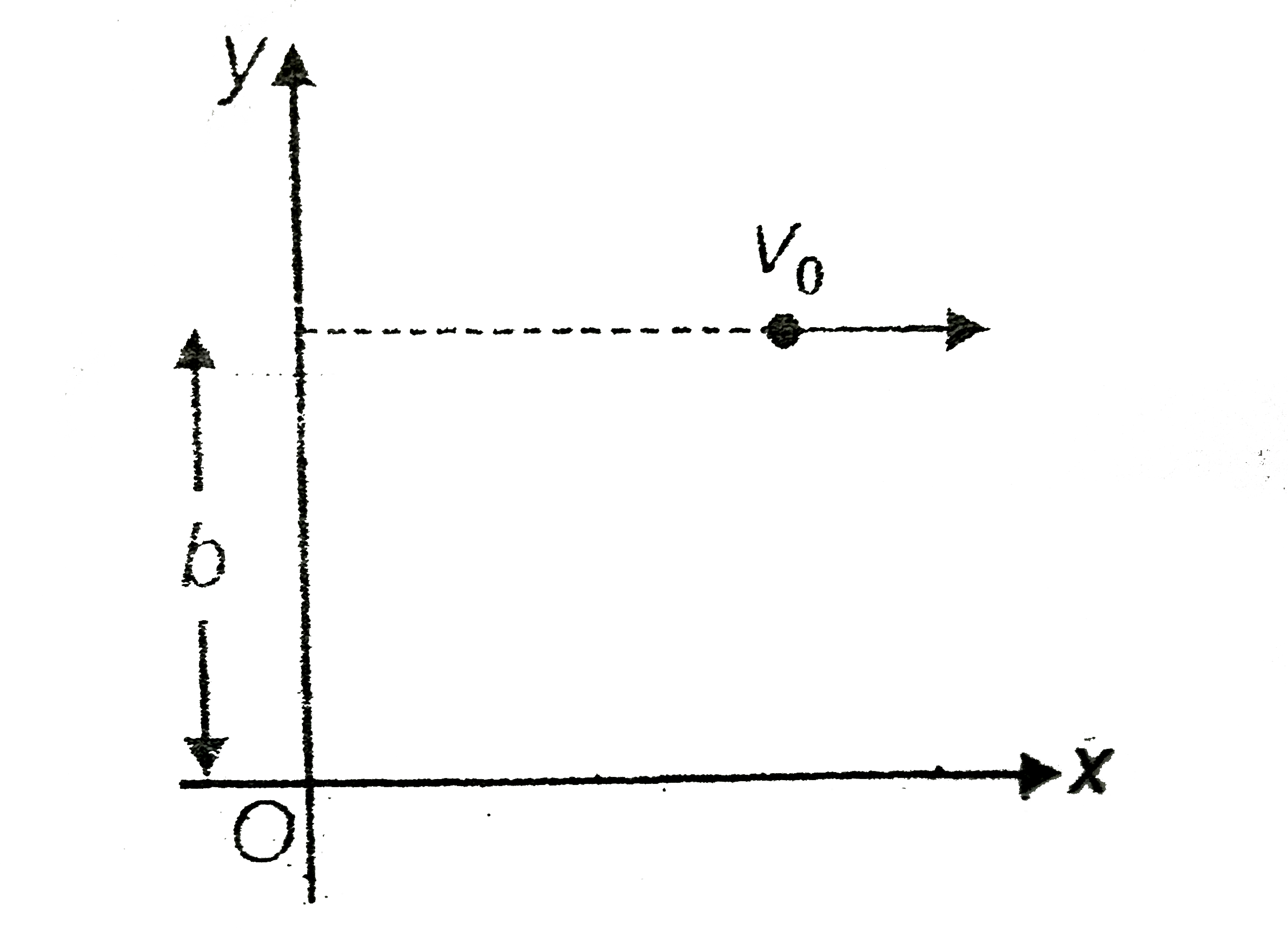
A particle of mass m is travelling with a constant velocity $v = v_{0} ˆ i$ along the line $y = b, z = 0$ . Let dA be the area swept out by the position vector from origin to the particle in time dt and L the magnitude of angular momentum of particle about origin at any time t. Then

A

L=constant

B

$L \neq constant$

C

$\frac{d A}{d t} = \frac{2 L}{m}$

D

$\frac{d A}{d t} = \frac{L}{2 m}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:A, D

To solve the problem step-by-step, we need to analyze the motion of the particle and calculate both the angular momentum $L$ and the area swept out $d A$ by the position vector from the origin to the particle.
Step 1: Understanding the Motion of the Particle
The particle of mass $m$ is moving with a constant velocity $\to v = v_{0}^i$ along the line defined by $y = b$ and $z = 0$ . This means the particle is moving in the x-direction while maintaining a constant y-coordinate of $b$ .
Step 2: Position Vector
The position vector $\to r$ of the particle at any time $t$ can be expressed as:
$\to r = x^i + b^j + 0^k = v_{0} t^i + b^j$
Step 3: Angular Momentum Calculation
The angular momentum $\to L$ of a particle about the origin is given by:
$\to L = \to r \times m \to v$
Where $\to v = v_{0}^i$ . The magnitude of angular momentum $L$ can be calculated as:
$L = | \to r \times m \to v |$
Calculating the cross product:
$\to r = (v_{0} t)^i + b^j$
$m \to v = m v_{0}^i$
The cross product $\to r \times m \to v$ is:
$\to r \times m \to v = ∣ ∣∣∣ ∣ \begin{matrix} ^i & ^j & ^k v_{0} t & b & 0 m v_{0} & 0 & 0 \end{matrix} ∣ ∣∣∣ ∣$
Calculating the determinant:
$=^i (b \cdot 0 - 0 \cdot 0) -^j (v_{0} t \cdot 0 - 0 \cdot m v_{0}) +^k (v_{0} t \cdot 0 - b \cdot m v_{0})$
$= - b m v_{0}^k$
Thus, the magnitude of angular momentum $L$ is:
$L = | b m v_{0} |$
Step 4: Area Swept Out Calculation
The area $d A$ swept out by the position vector in a small time $d t$ can be calculated using the formula for the area of a triangle:
$d A = \frac{1}{2} \times base \times height$
Here, the base is the distance traveled in the x-direction $v_{0} d t$ and the height is the constant distance $b$ :
$d A = \frac{1}{2} \times v_{0} d t \times b = \frac{1}{2} b v_{0} d t$
Step 5: Relating Area Swept Out to Angular Momentum
From the previous results, we can relate $d A$ to $L$ :
$\frac{d A}{d t} = \frac{1}{2} b v_{0}$
And since we have $L = b m v_{0}$ , we can express $d A$ in terms of $L$ :
$\frac{d A}{d t} = \frac{L}{2 m}$
Final Results
1. The magnitude of angular momentum $L$ is given by:
$L = b m v_{0}$
2. The area swept out in time $d t$ is given by:
$d A = \frac{1}{2} b v_{0} d t$
3. The relationship between the area swept out and angular momentum is:
$\frac{d A}{d t} = \frac{L}{2 m}$

Updated on:18/9/2024

|

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

DC PANDEY ENGLISH-ROTATIONAL MOTION-More than one option is correct

Home
Ask Doubt
Profile