Question

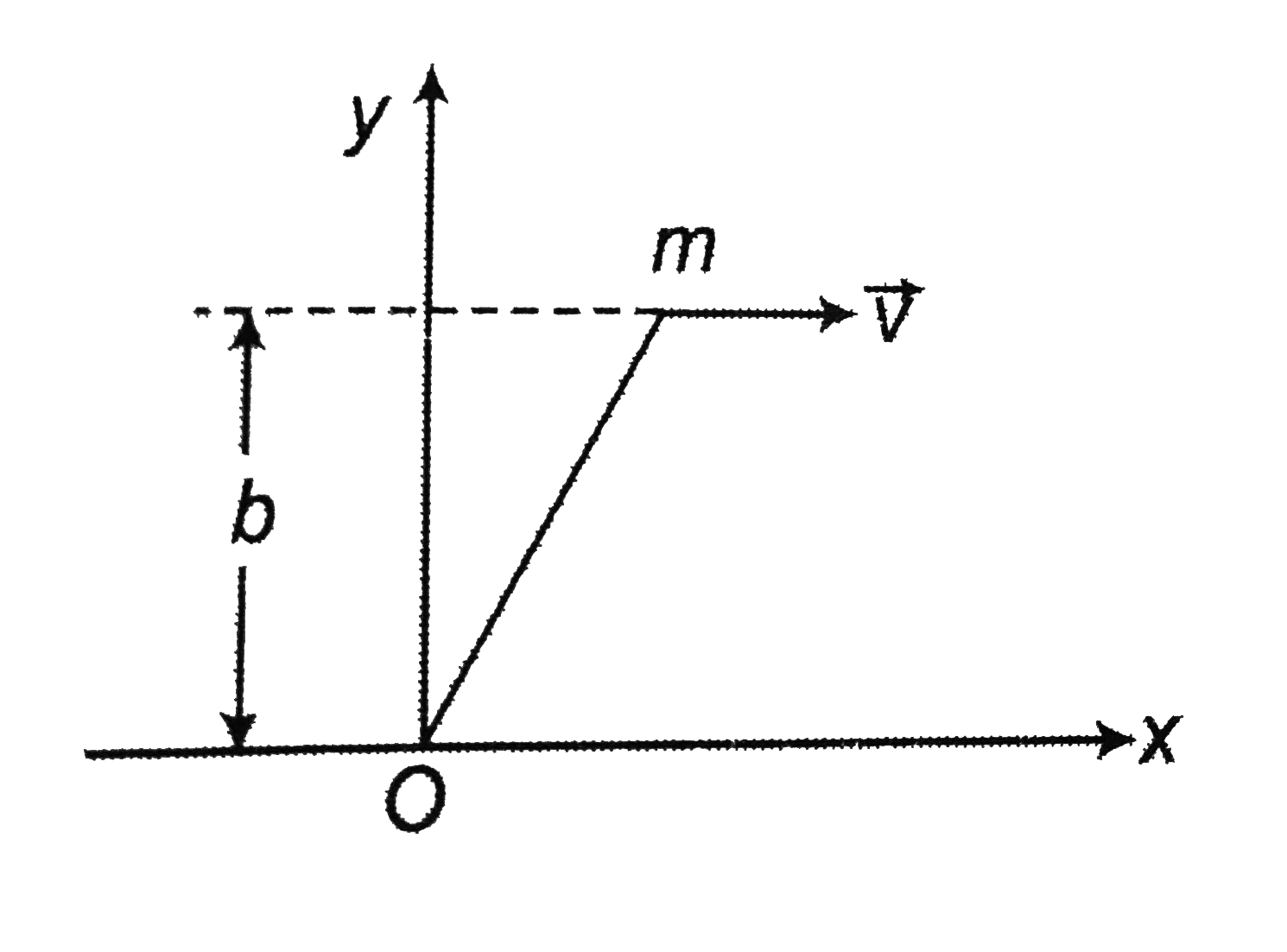
A particle of mass m is moving with constant speed v on the line y=b in positive x-direction. Find its angular momentum about origin, when position coordinates of the particle are (a, b).

Options

A. mvb
B. mvb/2
C. mvb/4
D. none

Answer

A

Explanation

To find the angular momentum of a particle of mass $$m$$ moving with constant speed $$v$$ along the line $$y=b$$ at the position coordinates $$(a,b)$$, we can follow these steps:

Step 1: Understand the Concept of Angular Momentum
Angular momentum \( L \) about a point (in this case, the origin) is given by the formula:
\( L = m \cdot v \cdot r \)
where:
- \( m \) is the mass of the particle,
- \( v \) is the velocity of the particle,
- \( r \) is the distance from the point about which we are calculating the angular momentum to the line of action of the velocity.

Step 2: Identify the Position and Velocity
The particle is at position \( (a, b) \) and is moving in the positive x-direction with a constant speed \( v \).

Step 3: Calculate the Distance \( r \)
The distance \( r \) from the origin to the particle can be calculated using the Pythagorean theorem:
\( r = \sqrt{a^2 + b^2} \)

Step 4: Determine the Perpendicular Component of Velocity
Since the velocity is in the x-direction, we need to find the component of this velocity that is perpendicular to the radius vector \( r \). The angle \( \theta \) between the radius vector and the x-axis can be found using:
\( \sin \theta = \frac{b}{\sqrt{a^2 + b^2}} \)
Thus, the perpendicular component of the velocity is:
\( v_{\perpendicular} = v \cdot \sin \theta = v \cdot \frac{b}{\sqrt{a^2 + b^2}} \)

Step 5: Substitute into the Angular Momentum Formula
Now we can substitute \( v_{\perpendicular} \) into the angular momentum formula:
\( L = m \cdot v_{\perpendicular} \cdot r = m \cdot \left(v \cdot \frac{b}{\sqrt{a^2 + b^2}}\right) \cdot \sqrt{a^2 + b^2} \)

Step 6: Simplify the Expression
The \( \sqrt{a^2 + b^2} \) terms cancel out:
\( L = m \cdot v \cdot b \)

Final Answer
Thus, the angular momentum \( L \) of the particle about the origin is:
\( L = m \cdot v \cdot b \)