In C−R circuit, answer the following two questions
During charging of C−R circuit let t1 and i1 be the time constant and initial charging current when capacitor is assumed to be filled by a perfect insulator and t2 and i2 be the corresponding values when it is assumed imperfect. Then
t1<t2
i1>i2
Both (a) and (b) are correct
Both (a) and (b) are wrong
The correct Answer is:C
To solve the problem regarding the charging of a capacitor in an RC circuit, we need to analyze the time constants and initial charging currents under two different assumptions about the capacitor's insulation.
1. Understanding Time Constant (τ):
- The time constant (τ) for an RC circuit is given by the formula:
τ=R⋅C
- Here, R is the resistance and C is the capacitance.
2. Initial Charging Current (I):
- The initial charging current (I0) can be calculated using Ohm's law:
I0=ER
- Where E is the electromotive force (EMF) of the circuit.
3. Case 1: Perfect Insulator:
- Let R1 be the resistance when the capacitor is assumed to be filled by a perfect insulator.
- The time constant and initial current in this case are:
τ1=R1⋅C
I1=ER1
4. Case 2: Imperfect Insulator:
- Let R2 be the resistance when the capacitor is assumed to be filled by an imperfect insulator.
- Since the insulation is imperfect, we assume R2>R1.
- The time constant and initial current in this case are:
τ2=R2⋅C
I2=ER2
5. Comparing Time Constants:
- Since R2>R1, we have:
τ2=R2⋅C>R1⋅C=τ1
- Therefore, τ2>τ1.
6. Comparing Initial Charging Currents:
- Since R2>R1, we have:
I2=ER2<ER1=I1
- Therefore, I2<I1.
7. Conclusion:
- We conclude that:
- The time constant when the capacitor is filled by an imperfect insulator is greater than that when filled by a perfect insulator: τ2>τ1.
- The initial charging current when the capacitor is filled by an imperfect insulator is less than that when filled by a perfect insulator: I2<I1.
Final Answers:
- τ2>τ1 (Time constant is greater for imperfect insulation)
- I2<I1 (Initial charging current is less for imperfect insulation)
- The time constant (τ) for an RC circuit is given by the formula:
τ=R⋅C
- Here, R is the resistance and C is the capacitance.
- The initial charging current (I0) can be calculated using Ohm's law:
I0=ER
- Where E is the electromotive force (EMF) of the circuit.
- Let R1 be the resistance when the capacitor is assumed to be filled by a perfect insulator.
- The time constant and initial current in this case are:
τ1=R1⋅C
I1=ER1
- Let R2 be the resistance when the capacitor is assumed to be filled by an imperfect insulator.
- Since the insulation is imperfect, we assume R2>R1.
- The time constant and initial current in this case are:
τ2=R2⋅C
I2=ER2
- Since R2>R1, we have:
τ2=R2⋅C>R1⋅C=τ1
- Therefore, τ2>τ1.
- Since R2>R1, we have:
I2=ER2<ER1=I1
- Therefore, I2<I1.
- We conclude that:
- The time constant when the capacitor is filled by an imperfect insulator is greater than that when filled by a perfect insulator: τ2>τ1.
- The initial charging current when the capacitor is filled by an imperfect insulator is less than that when filled by a perfect insulator: I2<I1.
- τ2>τ1 (Time constant is greater for imperfect insulation)
- I2<I1 (Initial charging current is less for imperfect insulation)
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Matrix Matching
2 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced
44 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
Similar Questions
Current growth in two L-R circuits (b) and (c ) as shown in figure (a). Let L1,L2,R1 and R2 be the corresponding values in two circuits. Then
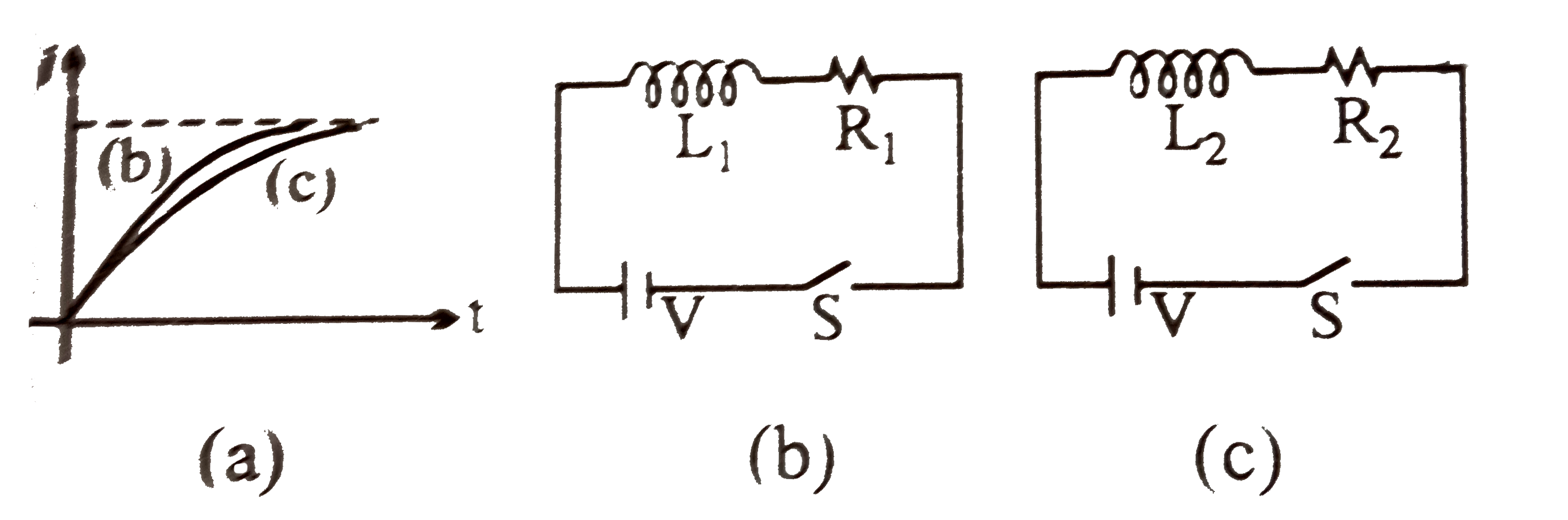
View SolutionIn charging singe loop R - C circuit, after how many time constant, the potential energy in the capacitor will be 25% of its maximum value.
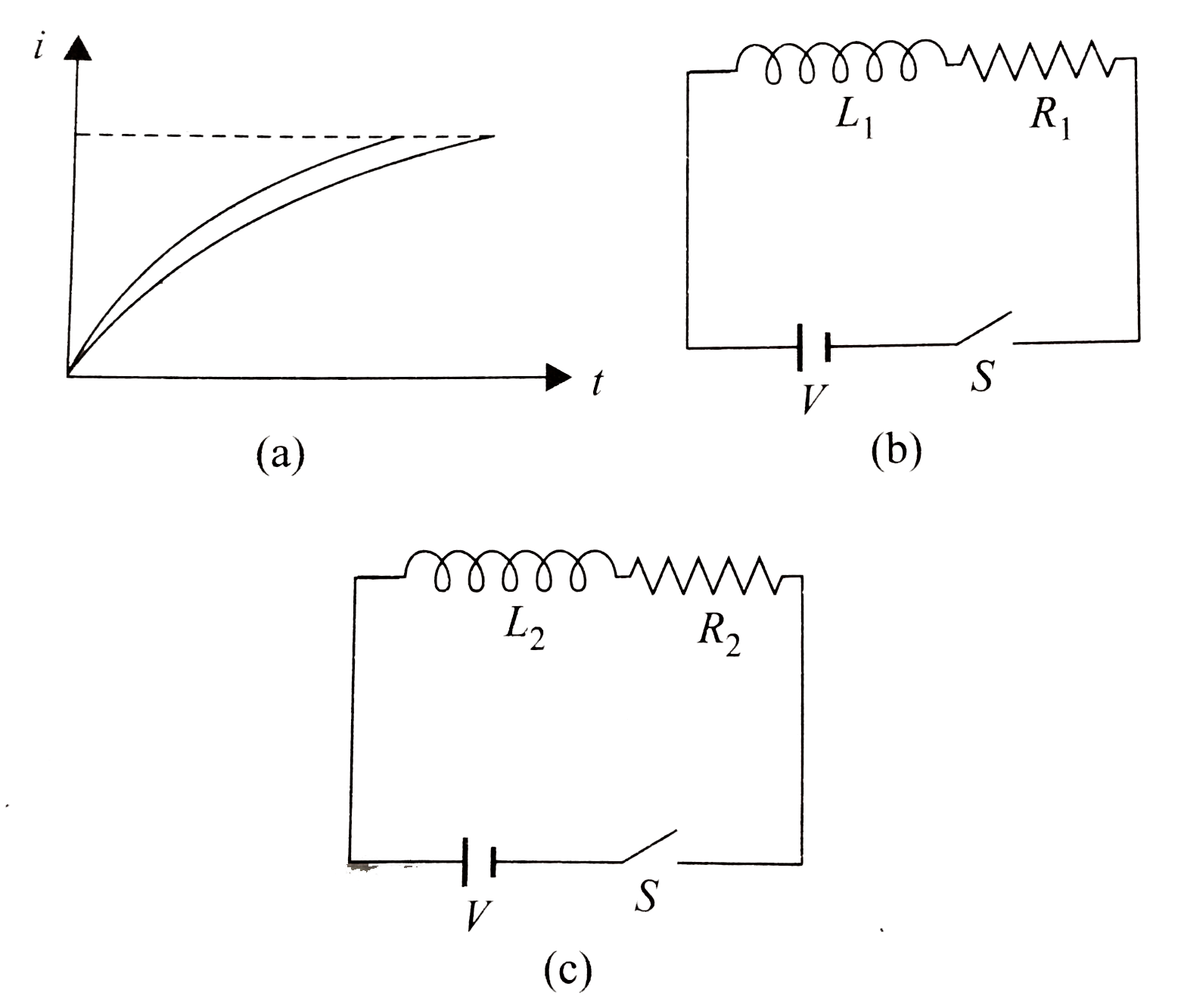
View SolutionThe current growth in two L-R circuits (b) and (c) is as shown in Fig. Let L1,L2,R1andR2 be the corresponding values in two circuits. Then
View SolutionWhen a capacitor discharges through a resistance R, the time constant is τ and the maximum current in the circuit is i0. Then,
View SolutionIn the given circuit find the ratio of i1toi2. Where is the initial (at t=0) current, and i2 is steady state (att=∞) current the battery

View SolutionTime constant of a C-R circuit is 2ln(2) second. Capacitor is discharged at time t=0. The ratio of charge on the capacitor at time t=2s and t=6s is
View SolutionThe following two questions refer to the circuit shown. Assume that the capacitors are initially uncharged.
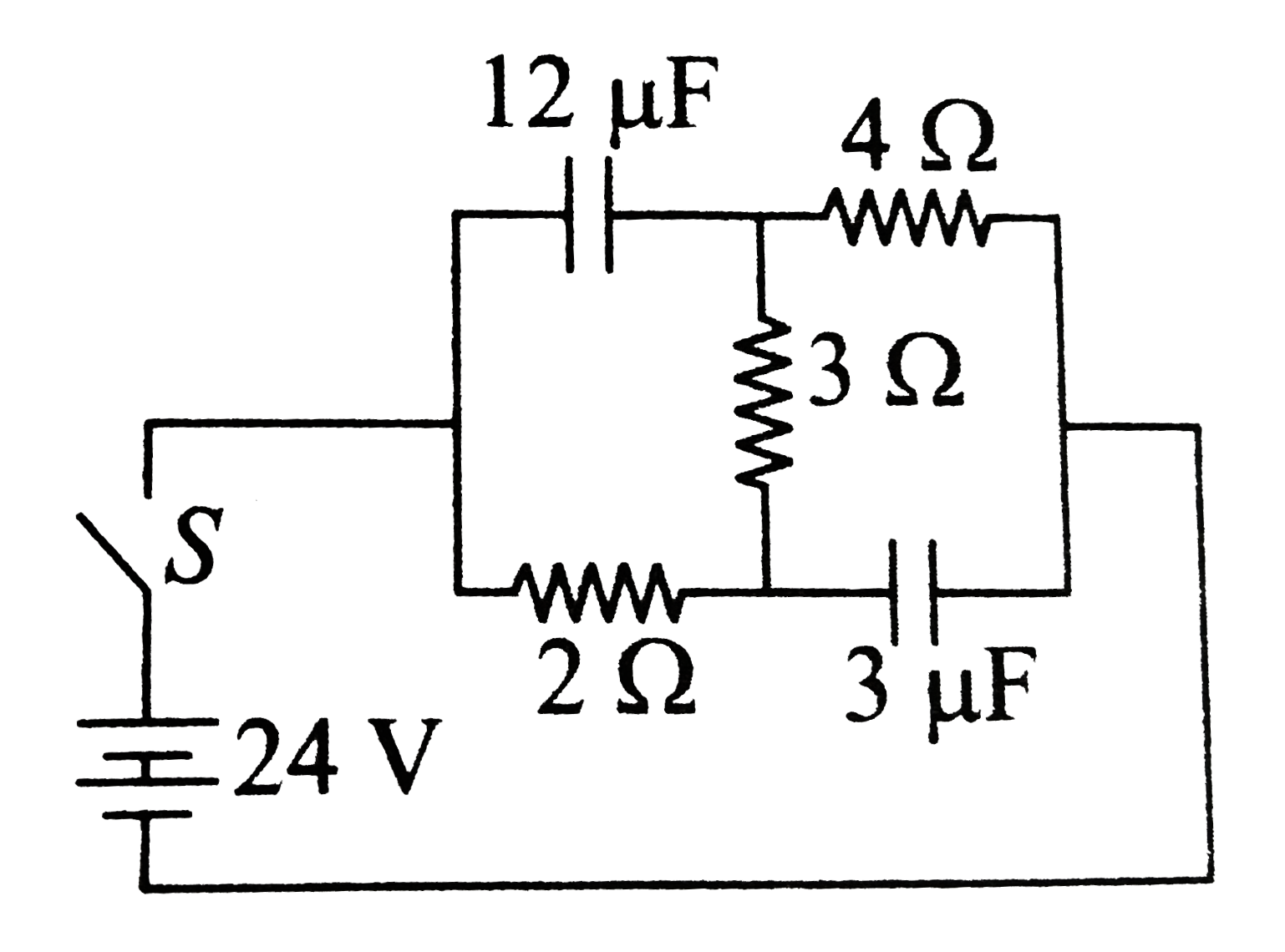
At time t = 0, the switch S in the circuit is closed. At that instant, what is the equivalent resistance of the circuit?
View SolutionIn a cirtain circuit current changes with time according to i=√t. Average value of current betweent=1 to t=2s is about to
View SolutionIn the given circuit, if I1andI2 be the current in resistances R1andR2, respectively, then
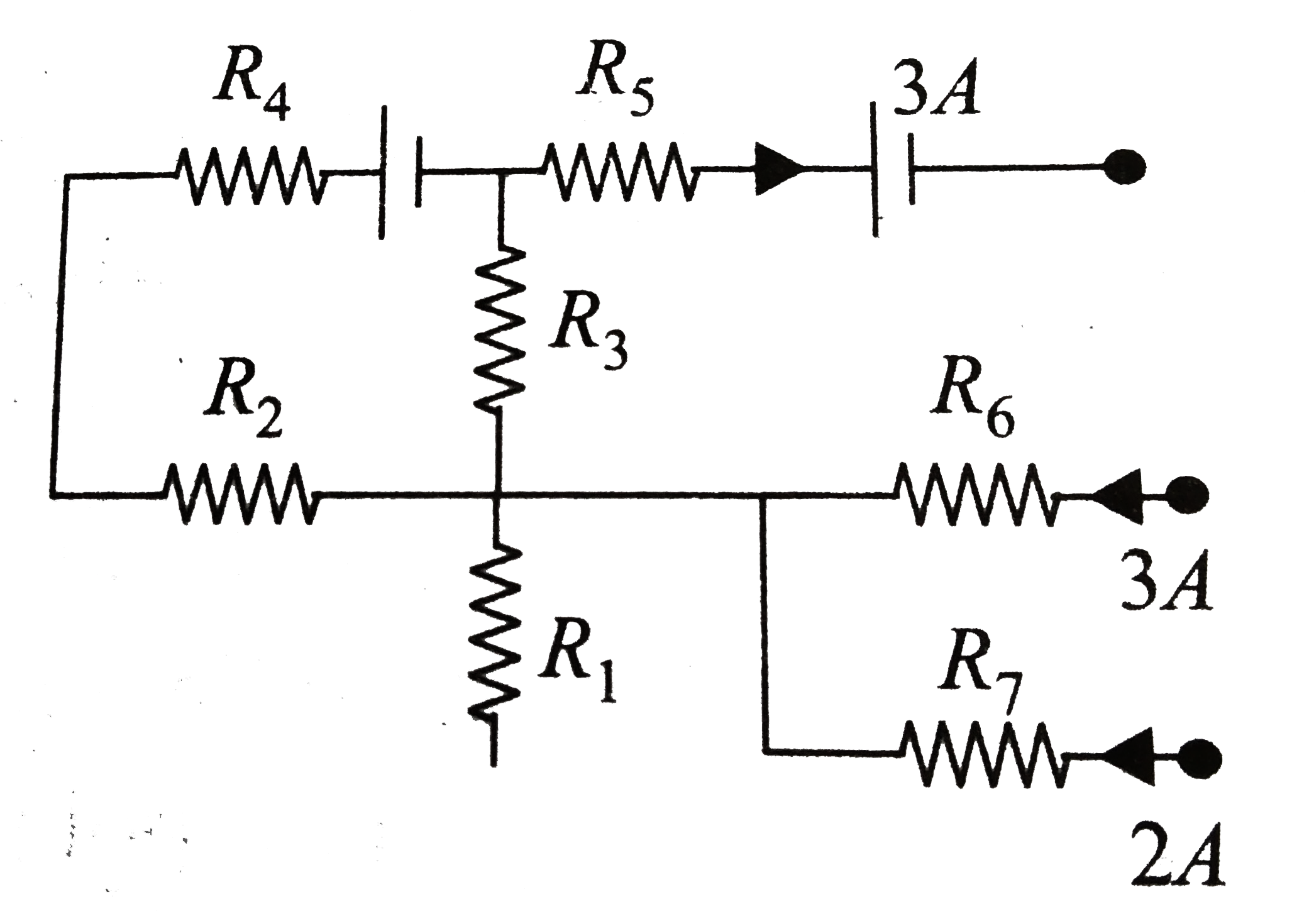 .
.
View SolutionThe following two questions refer to the circuit shown. Assume that the capacitors are initially uncharged.
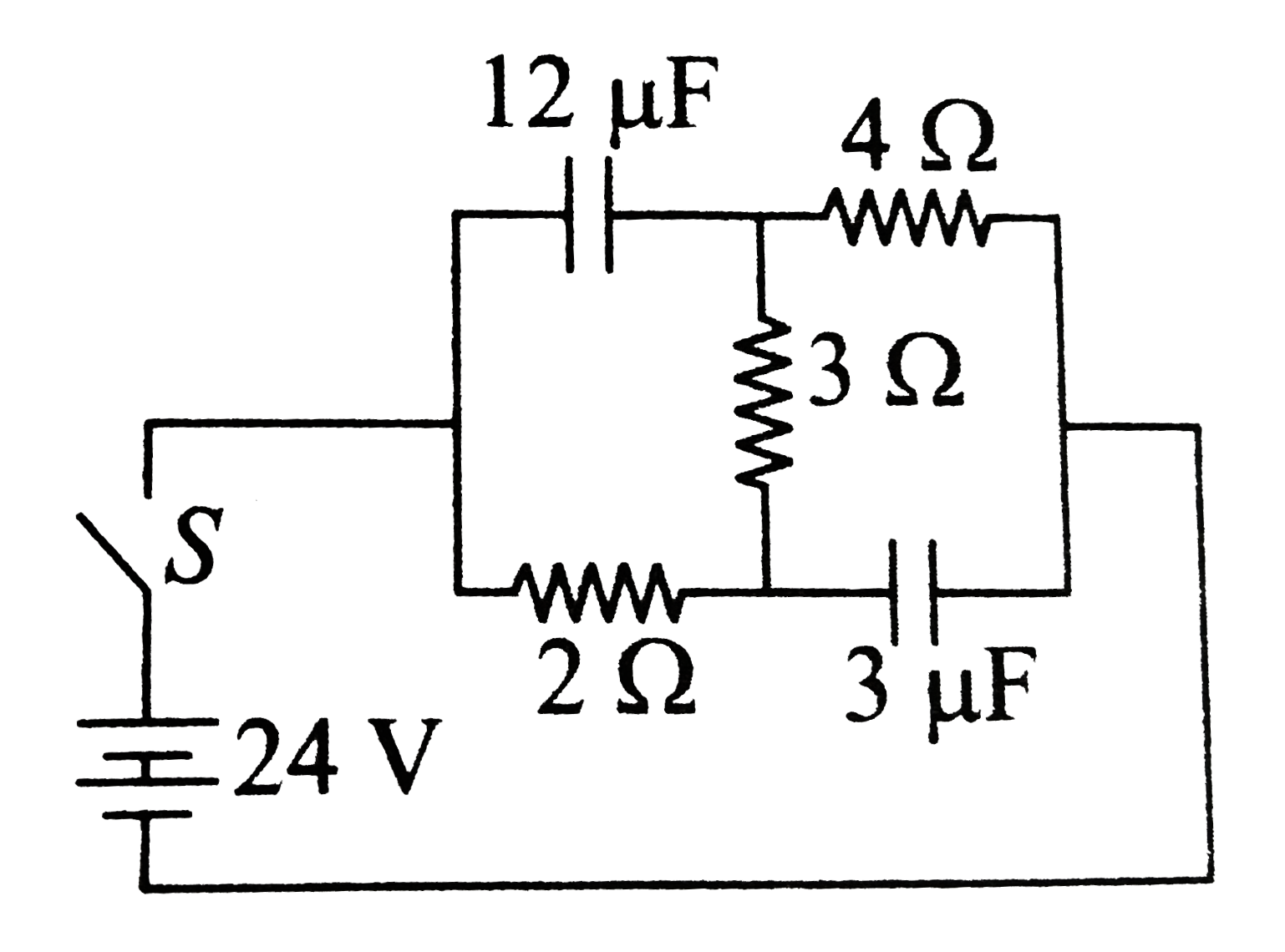
After the switch has been closed for a long time, how much charge is on the positive plate of the 3μF capacitor?
View Solution
