Question

Which of the following is the graph between the height (h) of a projectile and time (t), when it is projected from the ground

Options

A.
B.
C.
D.

Answer

C

Explanation

To determine the graph between the height (h) of a projectile and time (t) when it is projected from the ground, we can analyze the motion of the projectile step by step.

1. Understanding Projectile Motion:
- When a projectile is launched from the ground, it follows a parabolic trajectory. Initially, it rises to a maximum height and then falls back to the ground.

2. Initial Conditions:
- At time \( t = 0 \), the height \( h = 0 \) since the projectile is launched from the ground.

3. Equation of Motion:
- The height \( h \) of the projectile at any time \( t \) can be described by the equation:
\( h(t) = u_y t - \frac{1}{2} g t^2 \)
- Where:
- \( u_y = u_0 \sin(\alpha) \) is the initial vertical component of the velocity.
- \( g \) is the acceleration due to gravity (acting downwards).

4. Behavior of the Height with Time:
- As time increases from \( t = 0 \), the height \( h \) increases until it reaches the maximum height.
- After reaching the maximum height, the height \( h \) starts to decrease until it returns to the ground (height \( h = 0 \)).

5. Graph Characteristics:
- The graph starts at the origin (0,0).
- It rises to a peak (maximum height) and then falls back to the horizontal axis (height = 0).
- This behavior indicates that the graph is a concave down parabola.

6. Analyzing Options:
- We need to identify which option represents this behavior:
- Option 1: Incorrect, as it does not start from (0,0).
- Option 2: Incorrect, as it does not decrease after reaching a maximum.
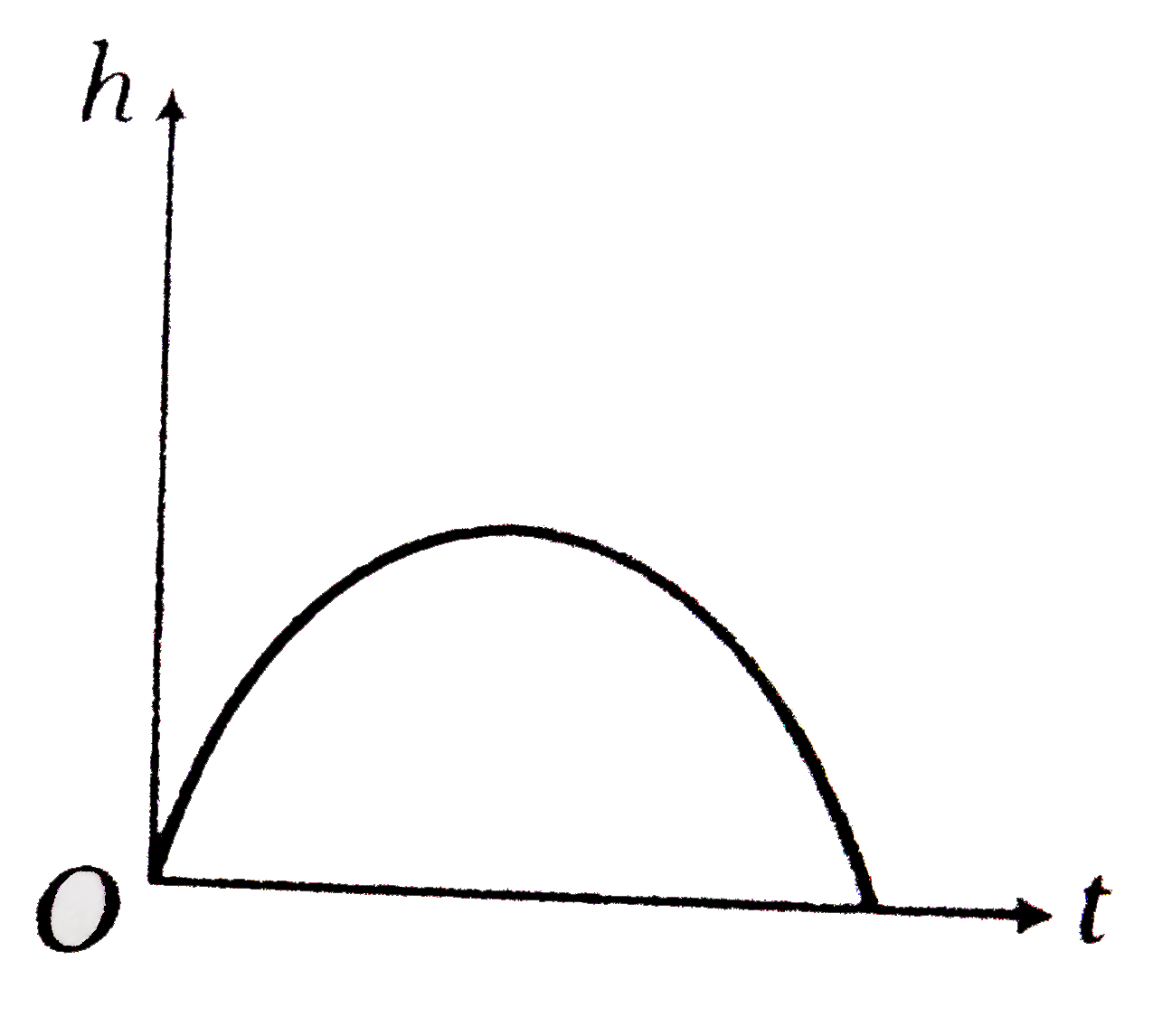
- Option 3: Correct, as it starts at (0,0), increases, and then decreases back to (0,0).
- Option 4: Incorrect, as it does not start from (0,0).

Conclusion:
The correct graph representing the height of a projectile as a function of time, when projected from the ground, is represented by Option 3.