Assertion : Two identical balls are charged by q. They are suspended from a common point by two insulating threads l each. In equilibrium, the angle between the tension in the threads is 180∘. (Ignore gravity).
Reason : In equilibrium tension in the spring is
T=14πε0q.ql2
If both Assertion and Reason are correct and Reason is the correct explanation of Assertion
If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion
If Assertion is true but Reason is false
If Assertion is false but Reason is true
The correct Answer is:C
To solve the problem step by step, we will analyze the assertion and the reason provided in the question.
Step 1: Understanding the Assertion
The assertion states that two identical balls, each charged with charge q, are suspended from a common point by two insulating threads of length l. In equilibrium, the angle between the tensions in the threads is 180∘.
- Since both balls are identical and have the same charge, they will repel each other due to electrostatic forces.
- In equilibrium, the only forces acting on each ball are the tension in the thread and the electrostatic force of repulsion between the two charged balls.
- The configuration where the angle between the threads is 180∘ means that the balls are directly opposite each other, maximizing the distance between them, which is consistent with the repulsive force.
Conclusion for Step 1
The assertion is correct because in equilibrium, the angle between the tensions in the threads can indeed be 180∘ when gravity is ignored.
Step 2: Analyzing the Reason
The reason states that in equilibrium, the tension in the spring (or thread) is given by the formula:
T=14πϵ0q⋅ql2
- The electrostatic force FE between the two charges q separated by a distance r is given by Coulomb's law:
FE=14πϵ0q2r2
- In this case, since the balls are at a distance of 2l apart (each thread has length l), the distance r=2l.
- Therefore, the electrostatic force becomes:
FE=14πϵ0q2(2l)2=14πϵ0q24l2=116πϵ0q2l2
Conclusion for Step 2
The tension T in the thread must equal the electrostatic force FE in equilibrium:
T=FE=116πϵ0q2l2
Thus, the reason provided in the question is incorrect because it does not account for the correct distance between the charges.
Final Conclusion
- The assertion is true.
- The reason is false.
The assertion states that two identical balls, each charged with charge q, are suspended from a common point by two insulating threads of length l. In equilibrium, the angle between the tensions in the threads is 180∘.
- In equilibrium, the only forces acting on each ball are the tension in the thread and the electrostatic force of repulsion between the two charged balls.
- The configuration where the angle between the threads is 180∘ means that the balls are directly opposite each other, maximizing the distance between them, which is consistent with the repulsive force.
The assertion is correct because in equilibrium, the angle between the tensions in the threads can indeed be 180∘ when gravity is ignored.
The reason states that in equilibrium, the tension in the spring (or thread) is given by the formula:
T=14πϵ0q⋅ql2
FE=14πϵ0q2r2
- In this case, since the balls are at a distance of 2l apart (each thread has length l), the distance r=2l.
- Therefore, the electrostatic force becomes:
FE=14πϵ0q2(2l)2=14πϵ0q24l2=116πϵ0q2l2
The tension T in the thread must equal the electrostatic force FE in equilibrium:
T=FE=116πϵ0q2l2
Thus, the reason provided in the question is incorrect because it does not account for the correct distance between the charges.
- The assertion is true.
- The reason is false.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Taking it together
105 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
Two identical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo pitch balls carrying equal charges are suspended from a common point by strings of equal length, the equilibrium separation between them is r. Now the strings are rigidly clamped at half the height. The equilibrium separation between the balls now become
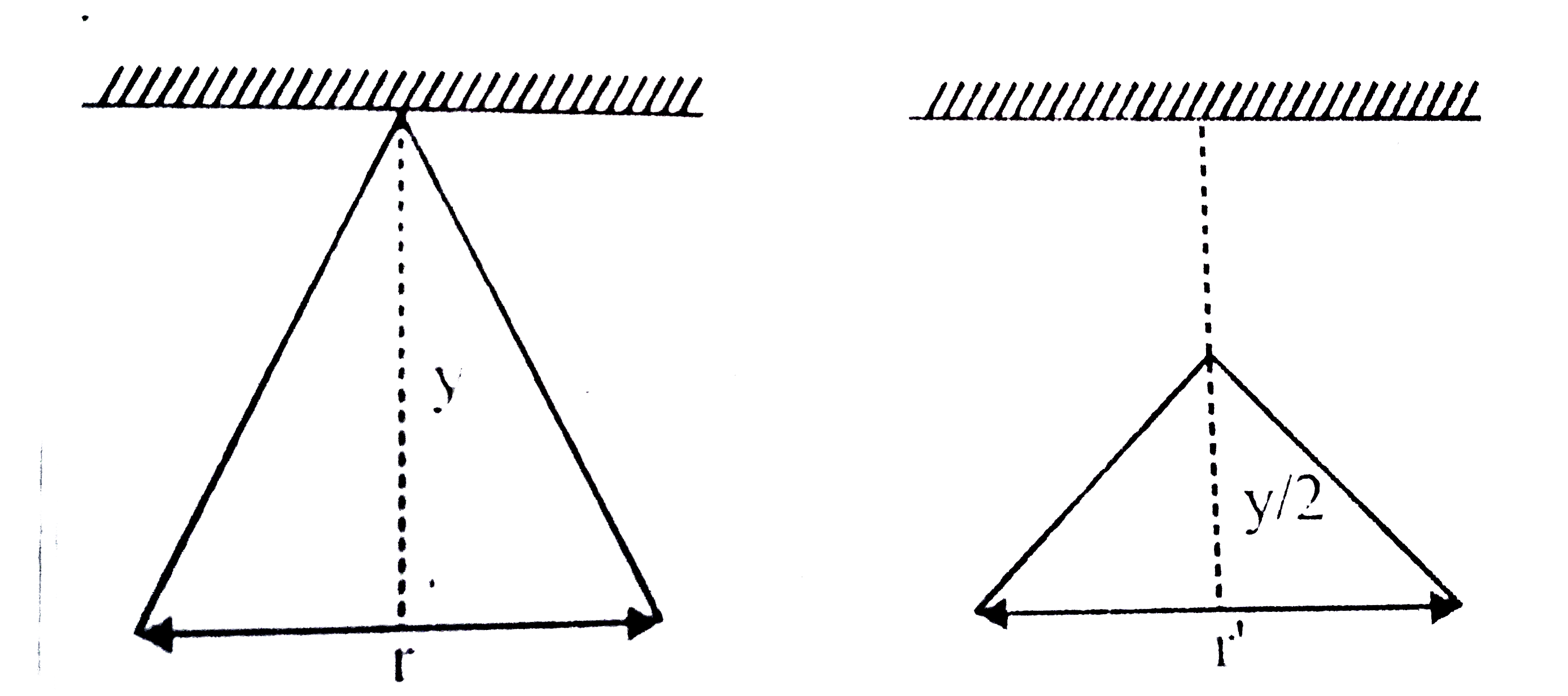
View SolutionTwo identical pith balls are charged by rubbing against each other. They are suspended from a horizontal rod through two strings of length 20 cm each, the separation between the suspension points being 5 cm. in equilibrium, the separation between the balls in 3 cm. Find the mass of each ball and the tension in the strings. the charge on each ball of a magnitude 2.0×10−8C.
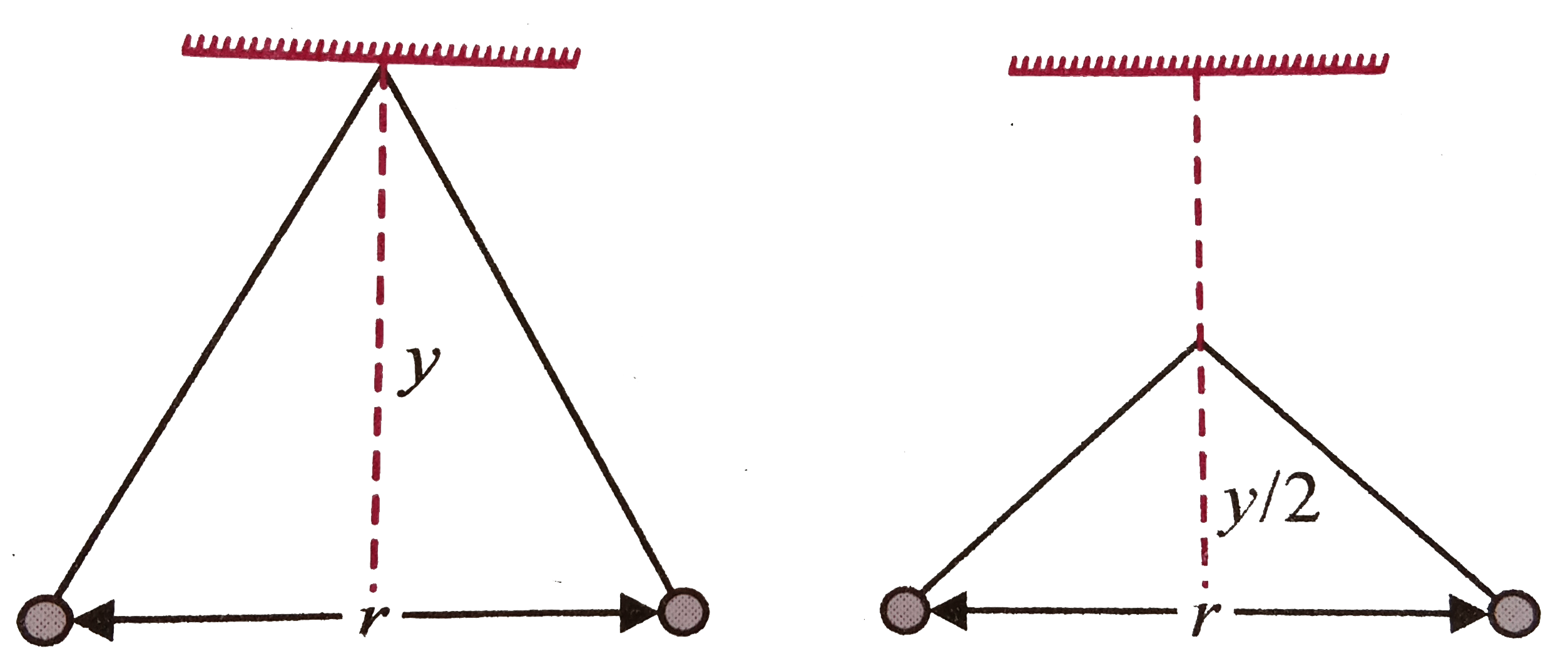
View SolutionTwo path balls carrying equal charges are suspended from a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View SolutionTwo identical balls each having a density ρ are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle θ with vertical. Now, both the balls are immersed in a liquid. As a result the angle θ does not change. The density of the liquid is σ. Find the dielectric constant of the liquid.
View SolutionTwo small balls having equal positive charge Q (coulumb) on each suspended by two insulating strings of equal length L (metre) from a hook fixed to a stand. The whole set-up is taken in a satellite into space where there is no gravity (state of weightlessness). Then tension (newtons) in each string is:
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo identical balls , each having a charge q and mass m , are suspended from a common point by two insultating strings each of length L. The balls are held at a separation x and then released. Find
(a) the electric force on each ball
(b) the component of the resultant force on a ball along and perpendicular to string
(c ) the tension in the string
(d) the acceleration of one of the balls. Consider the situation only for the instant just after the release.
View Solution
