In a closed circuit, the current I (in ampere) at an instant of time t (in second) is given by I=4−0.08t. The number of electrons flowing in 50 s through the cross-section of the conductor is
1.25×1019
6.25×1020
5.25×1019
2.25×1020
The correct Answer is:B
To solve the problem, we need to find the total charge flowing through the conductor in 50 seconds and then calculate the number of electrons corresponding to that charge. Here’s the step-by-step solution:
Step 1: Understand the relationship between current and charge
The current I is defined as the rate of flow of charge Q with respect to time t:
I=dQdt
This can be rearranged to express the charge in terms of current:
dQ=Idt
Step 2: Substitute the expression for current
We are given the current as a function of time:
I=4−0.08t
Substituting this into the equation for charge gives:
dQ=(4−0.08t)dt
Step 3: Integrate to find total charge over the interval
To find the total charge Q that flows through the conductor from t=0 to t=50 seconds, we integrate:
Q=∫500(4−0.08t)dt
Step 4: Perform the integration
Calculating the integral:
Q=∫5004dt−∫5000.08tdt
Calculating each part:
1. ∫5004dt=4t∣∣500=4×50−4×0=200
2. ∫5000.08tdt=0.08⋅t22∣∣500=0.08⋅5022=0.08⋅1250=100
Thus, we have:
Q=200−100=100Coulombs
Step 5: Calculate the number of electrons
The charge of a single electron e is approximately 1.6×10−19 Coulombs. The number of electrons n can be calculated using:
Q=n⋅e
Rearranging gives:
n=Qe=1001.6×10−19
Step 6: Perform the calculation
Calculating n:
n=1001.6×10−19=62.5×1020=6.25×1020
Conclusion
The number of electrons flowing through the cross-section of the conductor in 50 seconds is:
6.25×1020
The current I is defined as the rate of flow of charge Q with respect to time t:
I=dQdt
This can be rearranged to express the charge in terms of current:
dQ=Idt
We are given the current as a function of time:
I=4−0.08t
Substituting this into the equation for charge gives:
dQ=(4−0.08t)dt
To find the total charge Q that flows through the conductor from t=0 to t=50 seconds, we integrate:
Q=∫500(4−0.08t)dt
Calculating the integral:
Q=∫5004dt−∫5000.08tdt
Calculating each part:
1. ∫5004dt=4t∣∣500=4×50−4×0=200
2. ∫5000.08tdt=0.08⋅t22∣∣500=0.08⋅5022=0.08⋅1250=100
Q=200−100=100Coulombs
The charge of a single electron e is approximately 1.6×10−19 Coulombs. The number of electrons n can be calculated using:
Q=n⋅e
Rearranging gives:
n=Qe=1001.6×10−19
Calculating n:
n=1001.6×10−19=62.5×1020=6.25×1020
The number of electrons flowing through the cross-section of the conductor in 50 seconds is:
6.25×1020
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Taking it together
Explore 142 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:B. Assertion and reason
Explore 18 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Example
Explore 65 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:DC PANDEY ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Medical entrances gallery
Explore 25 Videos
Similar Questions
In a coil of self-inductance 10mH, the current I (in ampere) varies with time t (in second) as i=8t+2amp
View SolutionIn a coil of self-inductance 10mH, the current I (in ampere) varies with time t (in second) as i=8t+2amp
View SolutionA current of 4 A is flowing in a cylindrical conductor. The number of free electrons passing per second through the cross-section of conductor is
View SolutionThe length of a current-carrying cylindrical conductor is l, its area of cross-section is A, the number density of free electrons in it is n, and the drift velocity of electrons in it is vd . The number of electrons passing through a particular cross-section of the conductor per unit time is given by:
View SolutionA current of 1.6 mA flows through a conductor. If charge on an electron is -1.6×10−19 coulomb, find the number of electrons that will pass each second through the cross section of that conductor.
View SolutionThe current in a conductor varies with time .t. as I=3t+4t2. Where I in amp and t in sec. The electric charge flows through the section of the conductor between t = 1s and t = 3s
View SolutionA stready current i is flowing through a conductor of uniform cross-section. Any segment of the conductor has
View SolutionThe current I1 (in A) flowing through the 1Ω resistor in the given circuit is
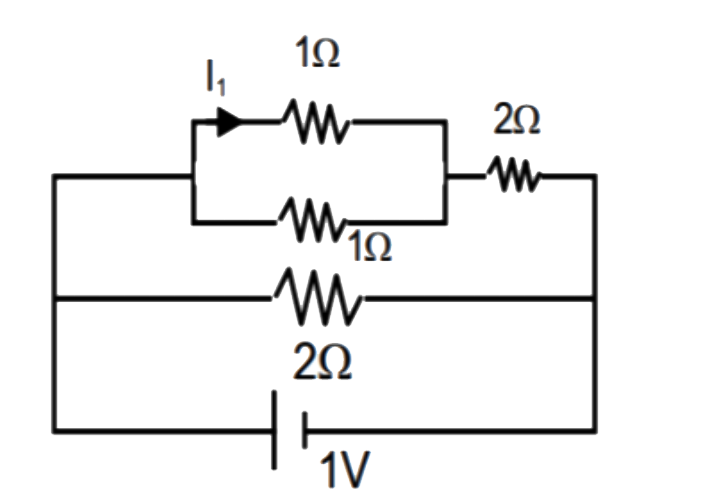
View SolutionThe current flowing through wire depends on time as, I=3t2+2t+5
The charge flowing through the cross - section of the wire in time t=0 to t=2 second is
View SolutionThe current in conductor varies with time t as I=2t+3t2 where I is in ampere and t in seconds. Electric charge flowing through a section of the conductor during t=2sec to t=3sec is
View Solution