Show that the percentage error in the measurement of resistance by a metre bridge is minimum when the null point is near about the centre of the wire.
20 cm
50 cm
80 cm
100 cm
The correct Answer is:C
To show that the percentage error in the measurement of resistance by a metre bridge is minimum when the null point is near about the center of the wire, we can follow these steps:
Step 1: Define the variables
Let:
- R = the resistance to be measured
- L = the length of the wire from one end to the null point
- The total length of the wire = 100 cm
Step 2: Establish the relationship using the metre bridge principle
According to the principle of the metre bridge, we have:
xR=L100−L
Where x is the resistance in the segment of the wire corresponding to the length L.
Step 3: Rearranging the equation
From the above equation, we can express x as:
x=R⋅L100−L
Step 4: Calculate the differential error in x
To find the error in the measurement of resistance x, we differentiate x:
Δx=R⋅(ΔL(100−L)−LΔL(100−L)2)
This simplifies to:
Δx=R⋅ΔL⋅(100−2L)(100−L)2
Step 5: Calculate the relative error in x
The relative error in x is given by:
Δxx=ΔL⋅(100−2L)L(100−L)
Step 6: Express the percentage error
To express this as a percentage error, we multiply by 100:
Percentage Error=ΔL⋅(100−2L)L(100−L)⋅100
Step 7: Minimize the percentage error
To minimize the percentage error, we need to maximize the term L(100−L). This is a quadratic function that achieves its maximum value when:
L=100−L⟹2L=100⟹L=50
Conclusion
Thus, the percentage error in the measurement of resistance by a metre bridge is minimum when the null point is at the center of the wire, i.e., L=50 cm.
Let:
- R = the resistance to be measured
- L = the length of the wire from one end to the null point
- The total length of the wire = 100 cm
According to the principle of the metre bridge, we have:
xR=L100−L
Where x is the resistance in the segment of the wire corresponding to the length L.
From the above equation, we can express x as:
x=R⋅L100−L
To find the error in the measurement of resistance x, we differentiate x:
Δx=R⋅(ΔL(100−L)−LΔL(100−L)2)
This simplifies to:
Δx=R⋅ΔL⋅(100−2L)(100−L)2
The relative error in x is given by:
Δxx=ΔL⋅(100−2L)L(100−L)
To express this as a percentage error, we multiply by 100:
Percentage Error=ΔL⋅(100−2L)L(100−L)⋅100
To minimize the percentage error, we need to maximize the term L(100−L). This is a quadratic function that achieves its maximum value when:
L=100−L⟹2L=100⟹L=50
Thus, the percentage error in the measurement of resistance by a metre bridge is minimum when the null point is at the center of the wire, i.e., L=50 cm.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Taking it together
142 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - B. Assertion and reason
18 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Example
65 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
Resistance of a given wire is obtained by measuring the current flowing in it and the voltage difference applied across it. If the percentage errors in the measurment of the current and the voltage difference are 3% each, then error in the value of resistance of the wire iS :
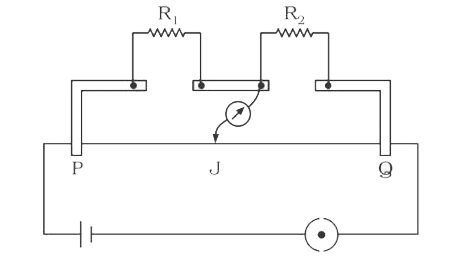
View SolutionThe circuit diagram given in the figure shown the experimental setup for the measurement of unknown resistance by using a metre bridge. The wire connected between the points P and Q(PQ = 100 cm) has uniform cross-sectional area and its resistivity is directly proportional to the distance from point P. Null point is obtained with the jockey J with R1andR2 in the gaps. If R1andR2 are interchanged, the jockey has to be displaced through a distance from the previous position along the wire to establish the null point. If the ratio of R1R2=3 , the value of Δ (in cm) is: [Ignore any end corrections]
View SolutionWhat is the condition for the error in measuring resistance on a Wheatstone bridge to be a minimum? How can it be achieved?
View SolutionDetermination of unknonw resistance in metre bridge is most accurate , when the balancing length is
View SolutionThe percentage error in measurement of a physical quantity [m given by m=πtanθ] is minimum when
(Assume that error in θ remain constant)
View SolutionThe percentage error in the measurement of the voltage V is 3% and in the measurement of the current is 2%. The percentage error in the measurement of the resistance is
View SolutionThe heat dissipated in a resistance can be obtained by the measurement of resistance, current and time. If the maximum percentage error in the measurement of these quantities are 1%,2%and1% respectively. The maximum percentage error in the determination of the dissipated heat is -
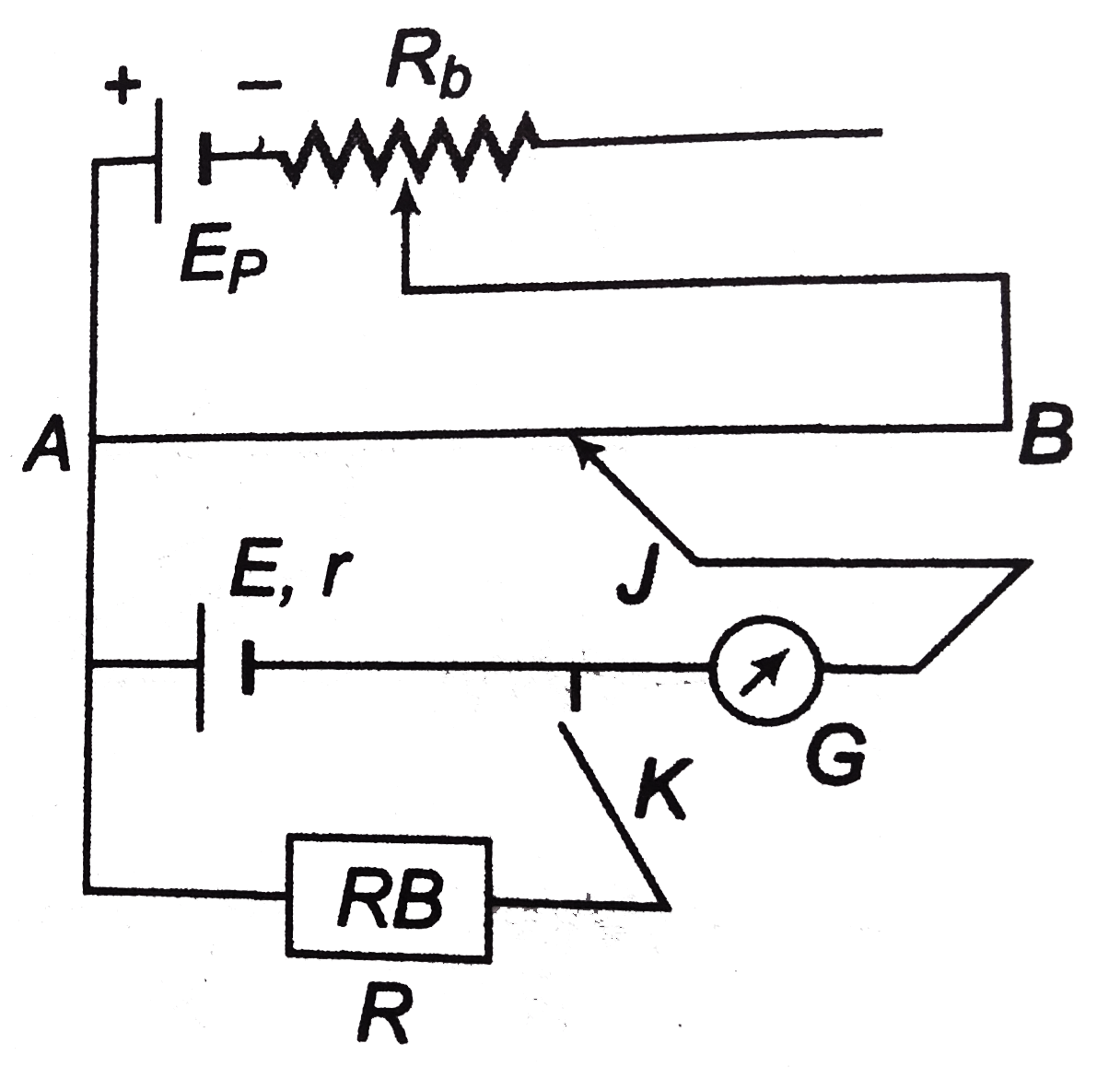
View SolutionIn an experiment on the measurement of internal resistance of as cell by using a potentionmeter, when the key K is kept open then balancing length is obtained at y metre. When the key K is closed and some resistance R is inserted in the resistance box, then the balancing length is found to be x metre. Then the internal resistance is
View SolutionThe resistance R of a wire is given by the relation R=ρlπr2. Percentage error in the measurement of ρ,landr is 1 % , 2% and 3% respectively. Then the percentage error in the measurement of R is
View SolutionThe resistance R of a wire is found by determining its length l and radius r. The percentage errors in measurement of l and r are respectively 1% and 2%. The percentage error in measurement of R is
View Solution
