Six resistance each of value r=5Ω are connected between points A, B and C as shown in figure. If R1,R2 and R3 are the net resistance between A and B, between B and C and between A and C respectively, then R1:R2:R3 will be equal to
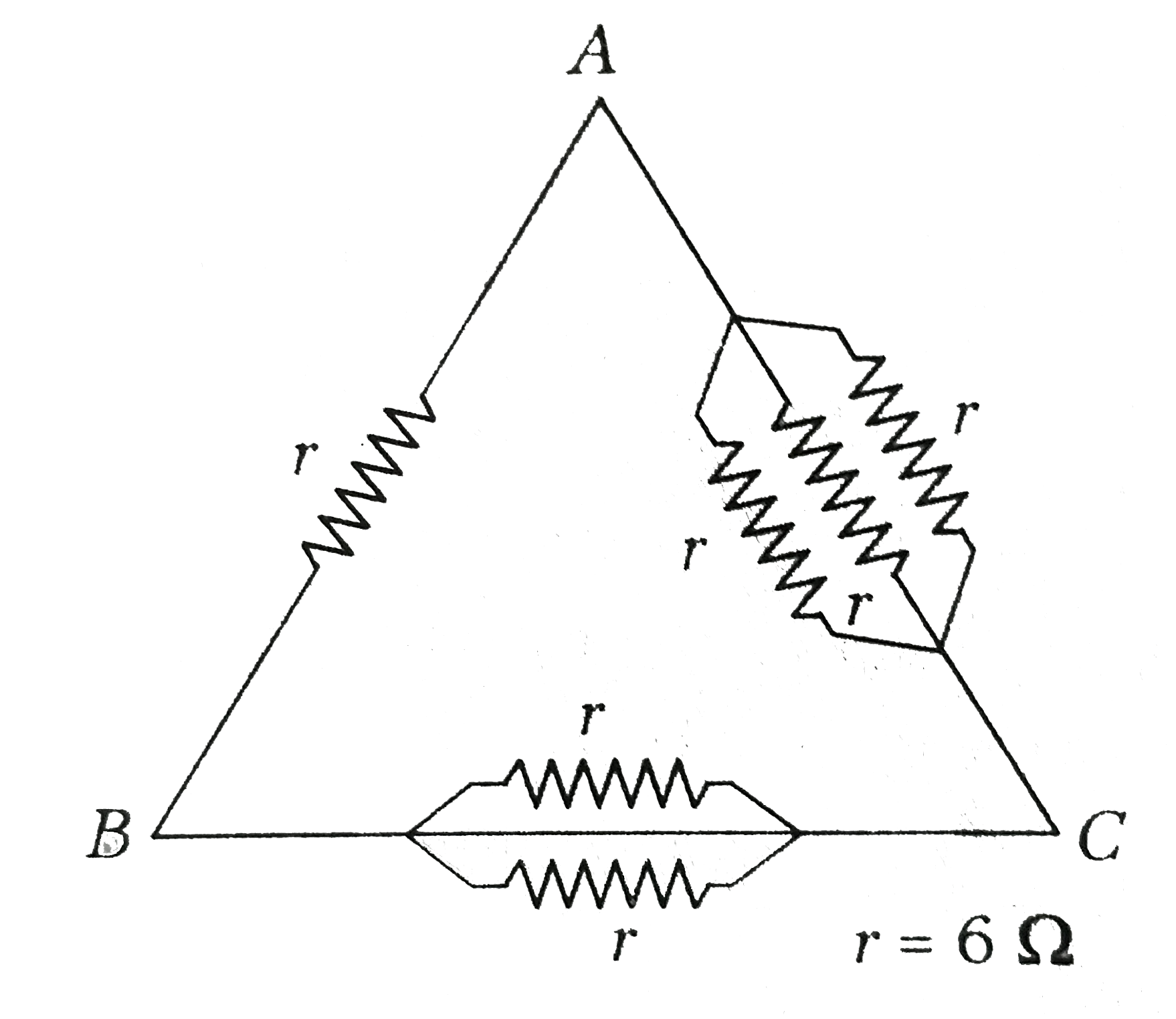
6:3:2
1:2:3
5:4:3
4:3:2
The correct Answer is:C
Step by step video & image solution for Six resistance each of value r=5 Omega are connected between points A, B and C as shown in figure. If R_(1), R_(2) and R_(3) are the net resistance between A and B, between B and C and between A and C respectively, then R_(1):R_(2):R_(3) will be equal to by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Match the columns
4 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
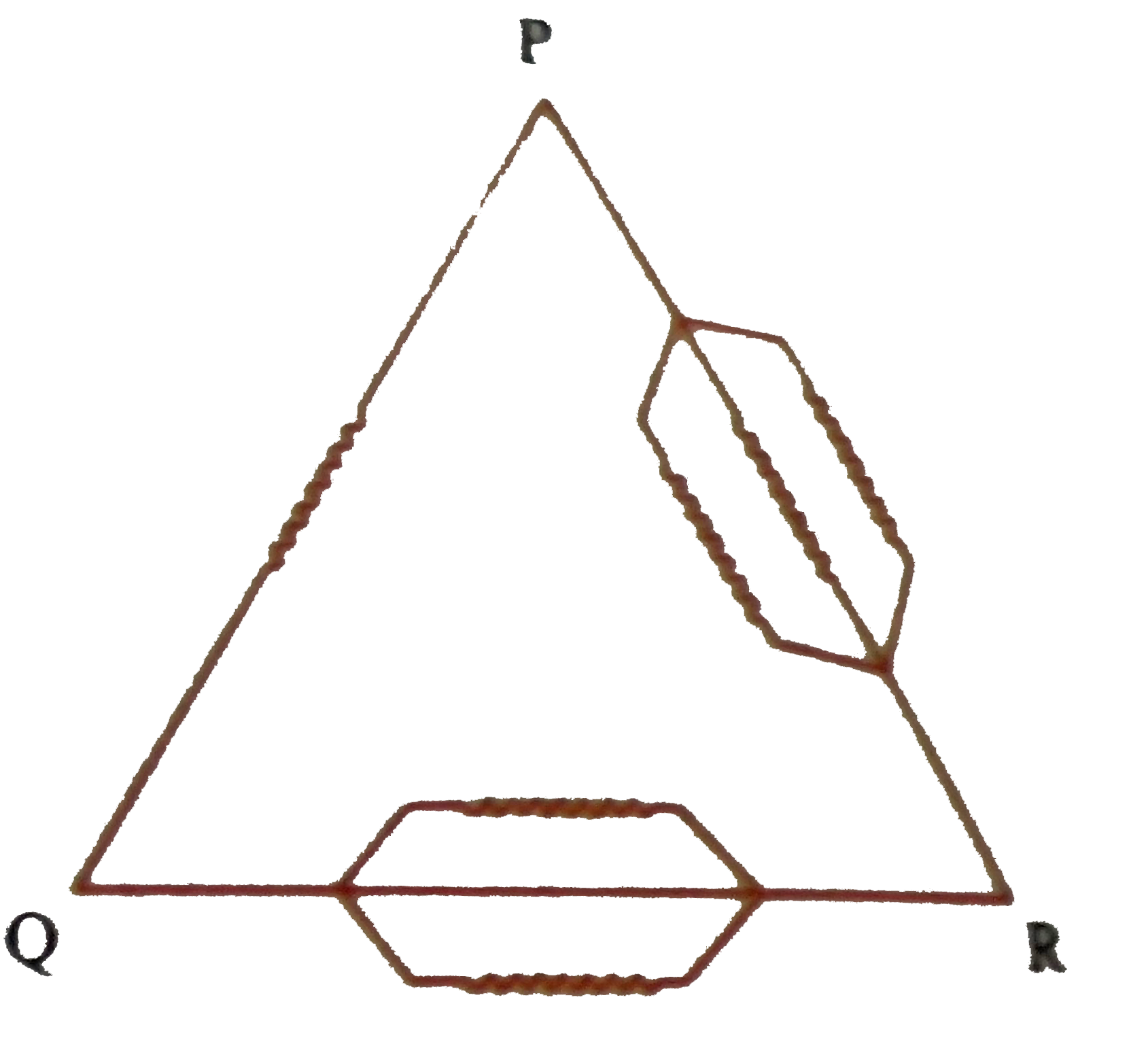
Six equal resistances are connected between points P, Q and R as shown in the figure. Then the net resistance will be maximum between
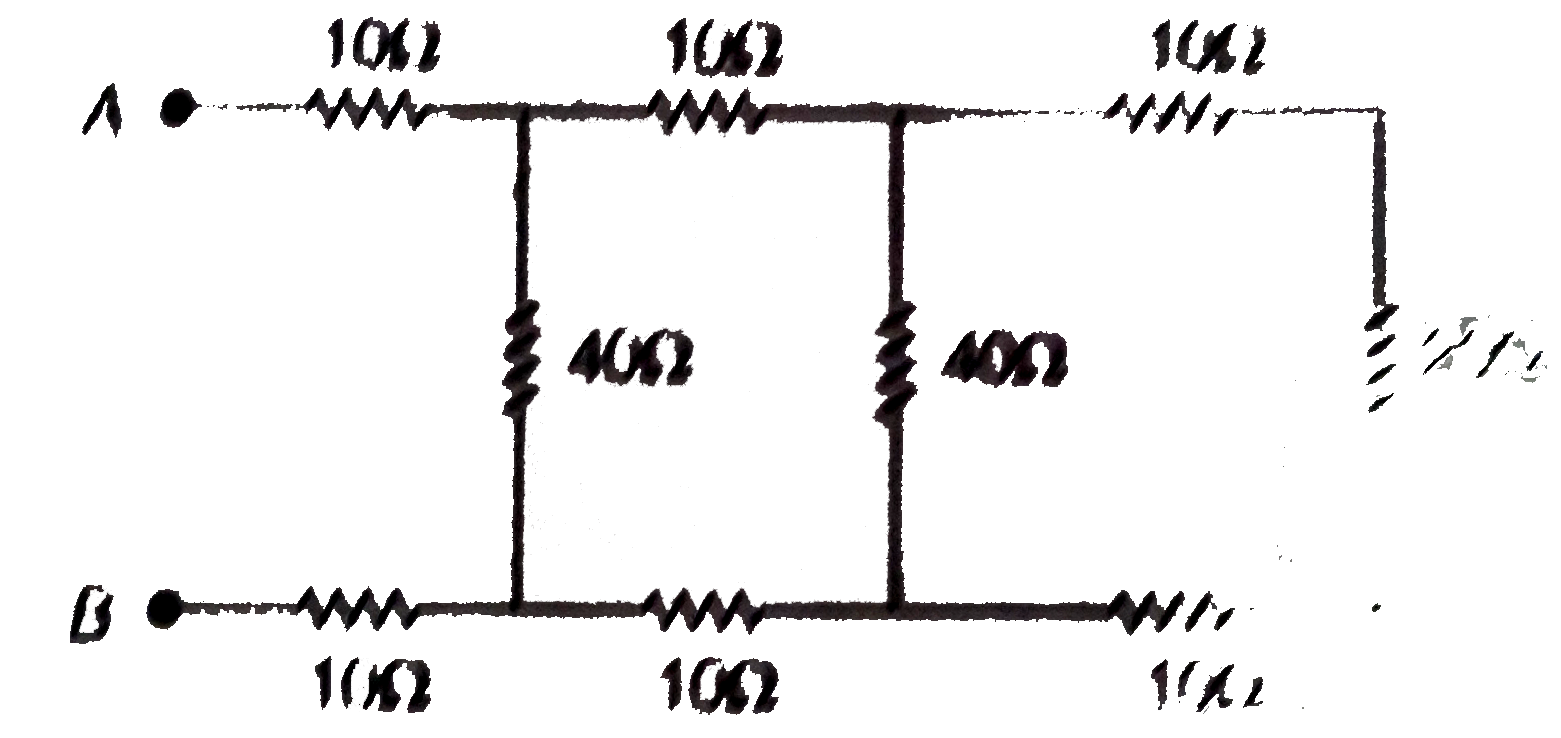
View SolutionFind R≠t between A and B.
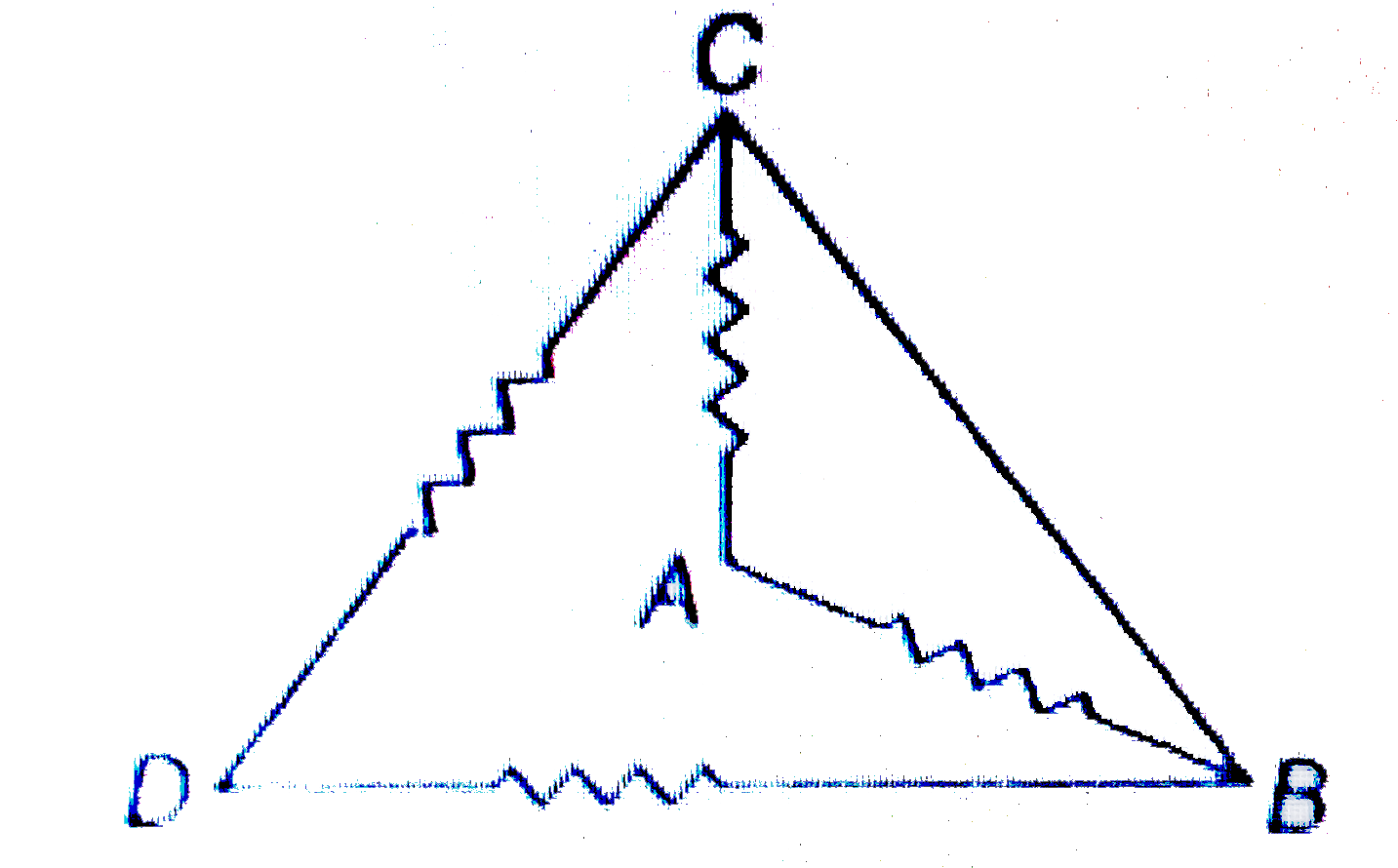
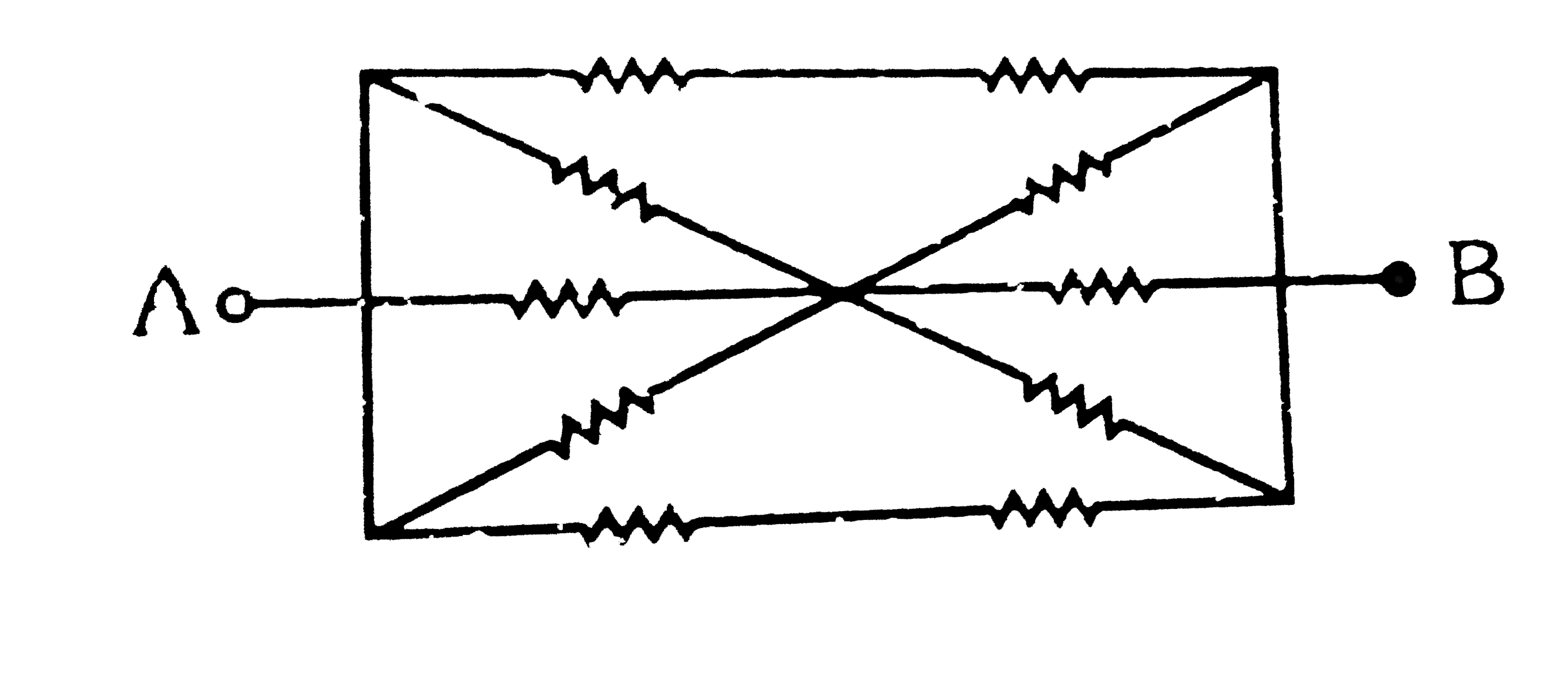
View SolutionEach resistance of the circuit shown in figure is r. The equivalent resistance between A and B is
View SolutionIn the network shown in figure, each resistance is R. The equvalent resistance between points A and B is

View SolutionFour identical resistances each having value R are arranged as shown in Figure. Find the equivalent resistance between A and B.

View SolutionCalculate effective resistance between A and B if each resistance is R.
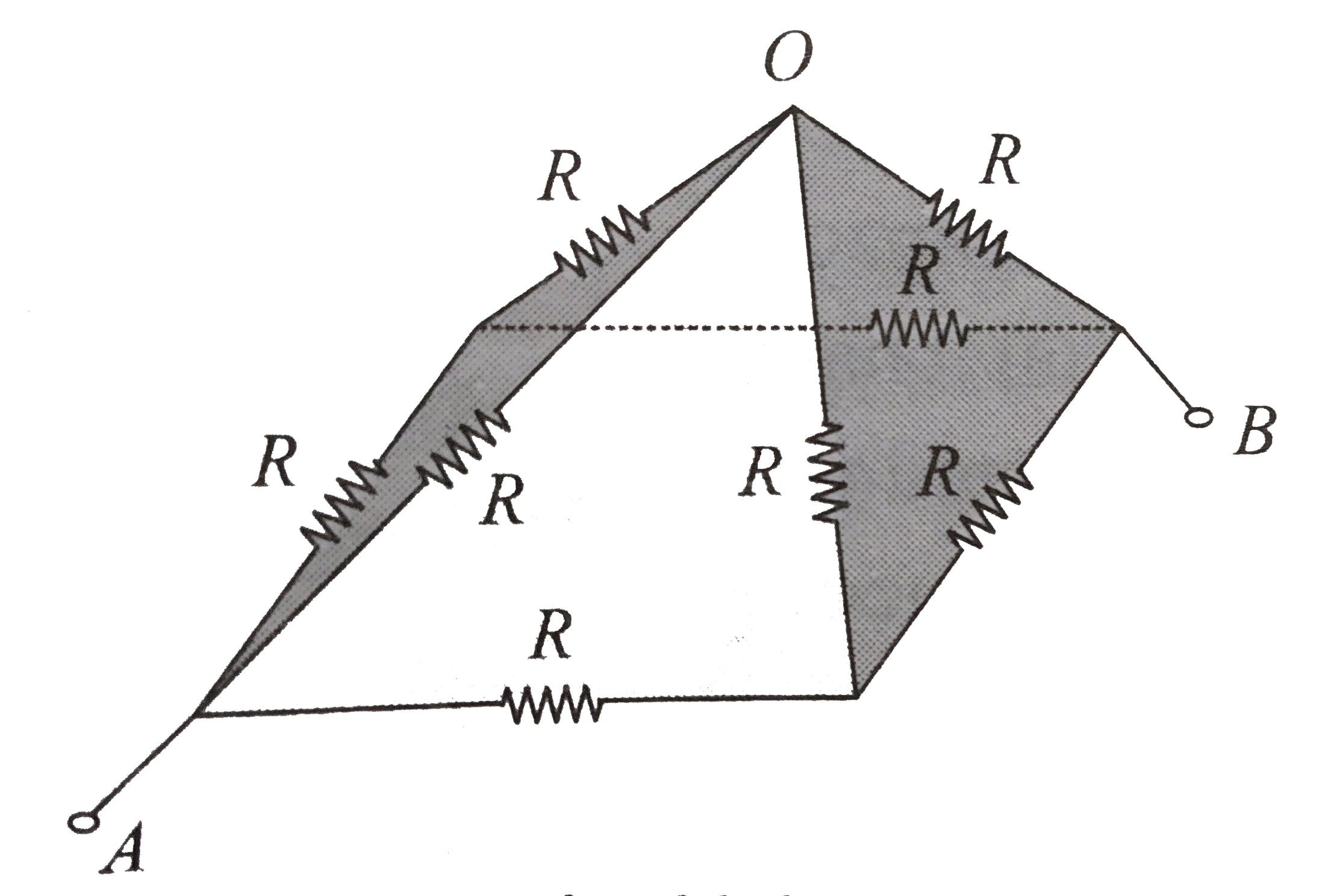
View SolutionIn the given figure, R=3Ω. Determine equivalent
resistance between points A and B.
 .
.
View SolutionIn the network shown in fig. each resistance is R.

The equivalent resistance between A and C is
View SolutionIn the netwrok shown in fig. each resistance is R.

The equivalent resistance between A and B is
View SolutionIn the network shown, each resistance is R. Calculate the equivalent resistance between A and B.

View Solution
