If G is the uciversal gravitational constant and p is the uniform density of a spherical planet. Then shortest possible period o0f rotation around a planet can be
The correct Answer is:D
To find the shortest possible period of rotation around a spherical planet with uniform density ρ, we can follow these steps:
1. Understand the Concept of Orbital Motion:
The shortest possible period of rotation (or orbital period) for an object around a planet occurs when the object is at the surface of the planet. This is because the gravitational force is strongest at the surface.
2. Use the Gravitational Force Equation:
The gravitational force acting on a satellite of mass m at a distance R (the radius of the planet) from the center of the planet is given by:
F=GMmR2
where M is the mass of the planet and G is the universal gravitational constant.
3. Relate Mass to Density:
The mass M of the planet can be expressed in terms of its uniform density ρ and volume V:
M=ρV=ρ(43πR3)
4. Substitute Mass into the Gravitational Force Equation:
Substitute M into the gravitational force equation:
F=G(ρ43πR3)mR2=4πGρmR3
5. Set Gravitational Force Equal to Centripetal Force:
For an object in circular motion, the centripetal force required is given by:
Fc=mv2R
where v is the orbital speed. Setting the gravitational force equal to the centripetal force gives:
4πGρmR3=mv2R
6. Cancel Mass and Rearrange:
Cancel m from both sides (assuming m≠0):
4πGρR3=v2R
Rearranging gives:
v2=4πGρR23
7. Relate Orbital Speed to Period:
The orbital speed v is also related to the orbital period T by:
v=2πRT
Squaring both sides gives:
v2=4π2R2T2
8. Set the Two Expressions for v2 Equal:
Set the two expressions for v2 equal to each other:
4π2R2T2=4πGρR23
9. Cancel R2 and Solve for T2:
Cancel R2 (assuming R≠0):
4π2T2=4πGρ3
Rearranging gives:
T2=3πGρ
10. Take the Square Root to Find T:
Finally, taking the square root gives:
T=√3πGρ
Final Answer:
The shortest possible period of rotation around the planet is:
T=√3πGρ
The shortest possible period of rotation (or orbital period) for an object around a planet occurs when the object is at the surface of the planet. This is because the gravitational force is strongest at the surface.
The gravitational force acting on a satellite of mass m at a distance R (the radius of the planet) from the center of the planet is given by:
F=GMmR2
where M is the mass of the planet and G is the universal gravitational constant.
The mass M of the planet can be expressed in terms of its uniform density ρ and volume V:
M=ρV=ρ(43πR3)
Substitute M into the gravitational force equation:
F=G(ρ43πR3)mR2=4πGρmR3
For an object in circular motion, the centripetal force required is given by:
Fc=mv2R
where v is the orbital speed. Setting the gravitational force equal to the centripetal force gives:
4πGρmR3=mv2R
Cancel m from both sides (assuming m≠0):
4πGρR3=v2R
Rearranging gives:
v2=4πGρR23
The orbital speed v is also related to the orbital period T by:
v=2πRT
Squaring both sides gives:
v2=4π2R2T2
Set the two expressions for v2 equal to each other:
4π2R2T2=4πGρR23
Cancel R2 (assuming R≠0):
4π2T2=4πGρ3
Rearranging gives:
T2=3πGρ
Finally, taking the square root gives:
T=√3πGρ
The shortest possible period of rotation around the planet is:
T=√3πGρ
Topper's Solved these Questions
Similar Questions
If ε0 is permittivity of free space, e is charge of proton, G is universal gravitational constant and mp is mass of a proton then the dimensional formula for
e24πε0Gm2p is
View SolutionA planet is revolving around the sun. its distance from the sun at apogee is rA and that at perigee is rp. The masses of planet and sun are 'm' and M respectively, VA is the velocity of planet at apogee and VP is at perigee respectively and T is the time period of revolution of planet around the sun, then identify the wrong answer.
View SolutionA point mass m, is placed at the surface of a hypothetical planet where acceleration due to gravity is g2, where g acceleration due to gravity at the poles the planet. The latitude of that position is [T is time period of planet rotation about its own axis and R is radius of planet]
View SolutionSuppose the gravitational force varies inversely as the nthpower of distance. Then the time period of a planet in circular orbit of radius R around the sun will be proportional to-
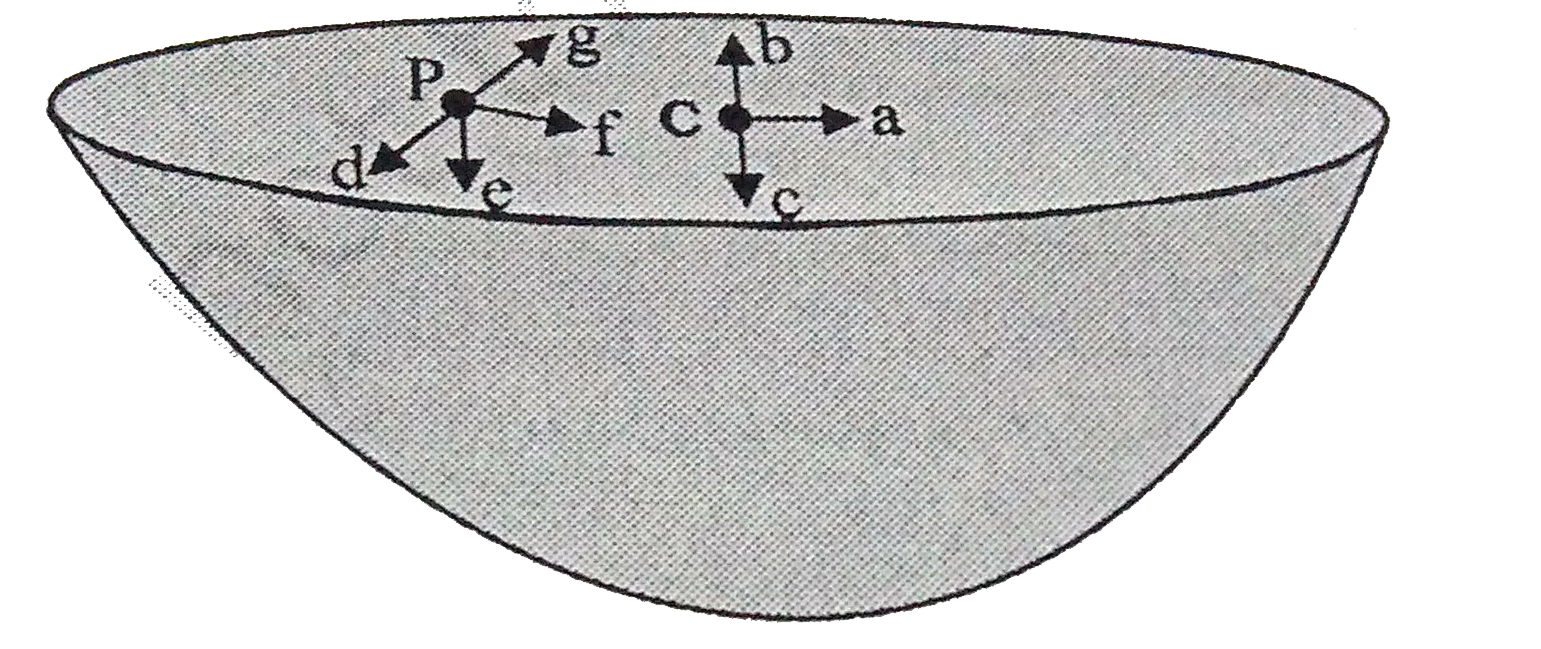
View SolutionIn the following two exercises, choose the correct answer from among the given ones: The gravitational intensity at the centre of a hemispherical shell of uniform mass density has the direction indicated by the arrow (see Fig.) (i) a, (ii) b, (iii) c, (iv) 0. For the above problem, the direction of the gravitational intensity at an arbitrary point P is indicated by the arrow (i) d, (ii), e, (iii) f (iv) g.
View SolutionA spherical uniform planet is rotating about its axis. The velocity of a point on its equator is V. Due to the rotation of planet about its axis the acceleration due to gravity g at equator is 1/2 of g at poles. The escape velocity of a particle on the planet in terms of V.
View SolutionGravitational acceleration on the surface of a planet is √611g. where g is the gravitational acceleration on the surface of the earth. The average mass density of the planet is 23 times that of the earth. If the escape speed on the surface of the earht is taken to be 11kms−1 the escape speed on the surface of the planet in kms−1 will be
View SolutionThe fastest possible of rotation of a planet is that for which the gravitational force on material at the equtor barely provides the centripetal force needed for the rotation. Show then that the corresponding shortest period of rotation is given by T=√3πGρ where ρ is the density of the planet, assumed to be homogeneous. Evalute the rotation period assuming a density of 3.0gm/cm2, typical of many planets, satellites, and asteroids. No such object is found to be spinning with a period shorter than found by this analysis.
View SolutionThe diameter and density of a planet are twice that of the earth. Assuming the earth and the planet to be of uniform density, find the ratio of the lengths of two simple pendulums, of equal time periods, on the surface of the planet and the earth.
View SolutionThe distance between the centres of the Moon and the earth is D. The mass of the earth is 81 times the mass of the Moon. At what distance from the centre of the earth, the gravitational force will be zero?
View Solution
