Three immiscibles transparent liquids with erefractive indices 3/2,4/3 and 6/5 are arranged one on top of another. The depth of the liquid are 3 cm, 4 cm and 6 cm respectively. The apparent depth of the vessel is
10 cm
9 cm
8 cm
7 cm
The correct Answer is:A
To solve the problem of finding the apparent depth of three immiscible transparent liquids with given refractive indices and depths, we can follow these steps:
Step 1: Identify the given data
- Refractive indices:
- Liquid 1: μ1=32
- Liquid 2: μ2=43
- Liquid 3: μ3=65
- Depths:
- Liquid 1: d1=3cm
- Liquid 2: d2=4cm
- Liquid 3: d3=6cm
Step 2: Use the formula for apparent depth
The relationship between real depth and apparent depth is given by:
μ=dh′
Where:
- μ is the refractive index,
- d is the real depth,
- h′ is the apparent depth.
From this, we can rearrange the formula to find the apparent depth:
h′=dμ
Step 3: Calculate the apparent depths for each liquid
1. For Liquid 1:
h′1=d1μ1=3cm32=3×23=2cm
2. For Liquid 2:
h′2=d2μ2=4cm43=4×34=3cm
3. For Liquid 3:
h′3=d3μ3=6cm65=6×56=5cm
Step 4: Calculate the total apparent depth
Now, we can find the total apparent depth h′ by summing the apparent depths of all three liquids:
h′=h′1+h′2+h′3=2cm+3cm+5cm=10cm
Final Answer
The apparent depth of the vessel is 10cm.
---
- Refractive indices:
- Liquid 1: μ1=32
- Liquid 2: μ2=43
- Liquid 3: μ3=65
- Depths:
- Liquid 1: d1=3cm
- Liquid 2: d2=4cm
- Liquid 3: d3=6cm
The relationship between real depth and apparent depth is given by:
μ=dh′
Where:
- μ is the refractive index,
- d is the real depth,
- h′ is the apparent depth.
h′=dμ
1. For Liquid 1:
h′1=d1μ1=3cm32=3×23=2cm
h′2=d2μ2=4cm43=4×34=3cm
h′3=d3μ3=6cm65=6×56=5cm
Now, we can find the total apparent depth h′ by summing the apparent depths of all three liquids:
h′=h′1+h′2+h′3=2cm+3cm+5cm=10cm
The apparent depth of the vessel is 10cm.
Topper's Solved these Questions
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.4
20 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.5
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.2
10 Videos
- NUCLEIBOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY
46 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
Similar Questions
A beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
View SolutionA vessel contains water up to a height of 20 cm and above it an oil up to another 20 cm. The refractive indices of the water and the oil are 1.33 and F30 respectively. Find the apparent depth of the vessel when viewed from above.
View SolutionA vessel of depth 2h is half filled with a liquid of refractive index 2√2 and the upper half with another liquid of refractive index √2. The liquids are immiscible. The apparent depth of the inner surface of the bottom of vessel will be:
View SolutionA vessel is quarter filled with a liquid of refractive index μ. The remaining parts of the vessel is filled with an immiscible liquid of refractive index 3μ/2. The apparent depth of the vessel is 50% of the actual depth. The value of μ is
View SolutionA vessel of depth 2d cm is half filled with a liquid of refractive index μ1 and the upper half with a liquid of refractive index μ2. The apparent depth of the vessel seen perpendicularly is
View SolutionA vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionA vessle of depth d is first filled to (23)rd of its depth with a liquid of refractive index 32 and the rest of the vessel is filled with a liquid of refractive index 43. What is the apparent depth of the inner surface of the bottom of the vessle.
View SolutionCan a triangle have
three sides whose lengths are 3 . 5 cm, 4 . 8 cm and 9 . 3 cm ?
View SolutionA metal coin at the bottom of a beaker filled with a liquid of refractive index 4/3 to height of 6cm. To an observer looking from above the surface of the liquid, coin will appear at a depth of (consider paraxial rays).
View SolutionA tank contains three layers of immiscible liquids. The first layer is of water with refractive index 4/3 and thickness 8cm. The second layer is of oil with refractive index 3/2 and thickness 9cm while the third layer is of glycerine with refractive index 2 and thickness 4cm. Find the apparent depth of the bottom of the container.
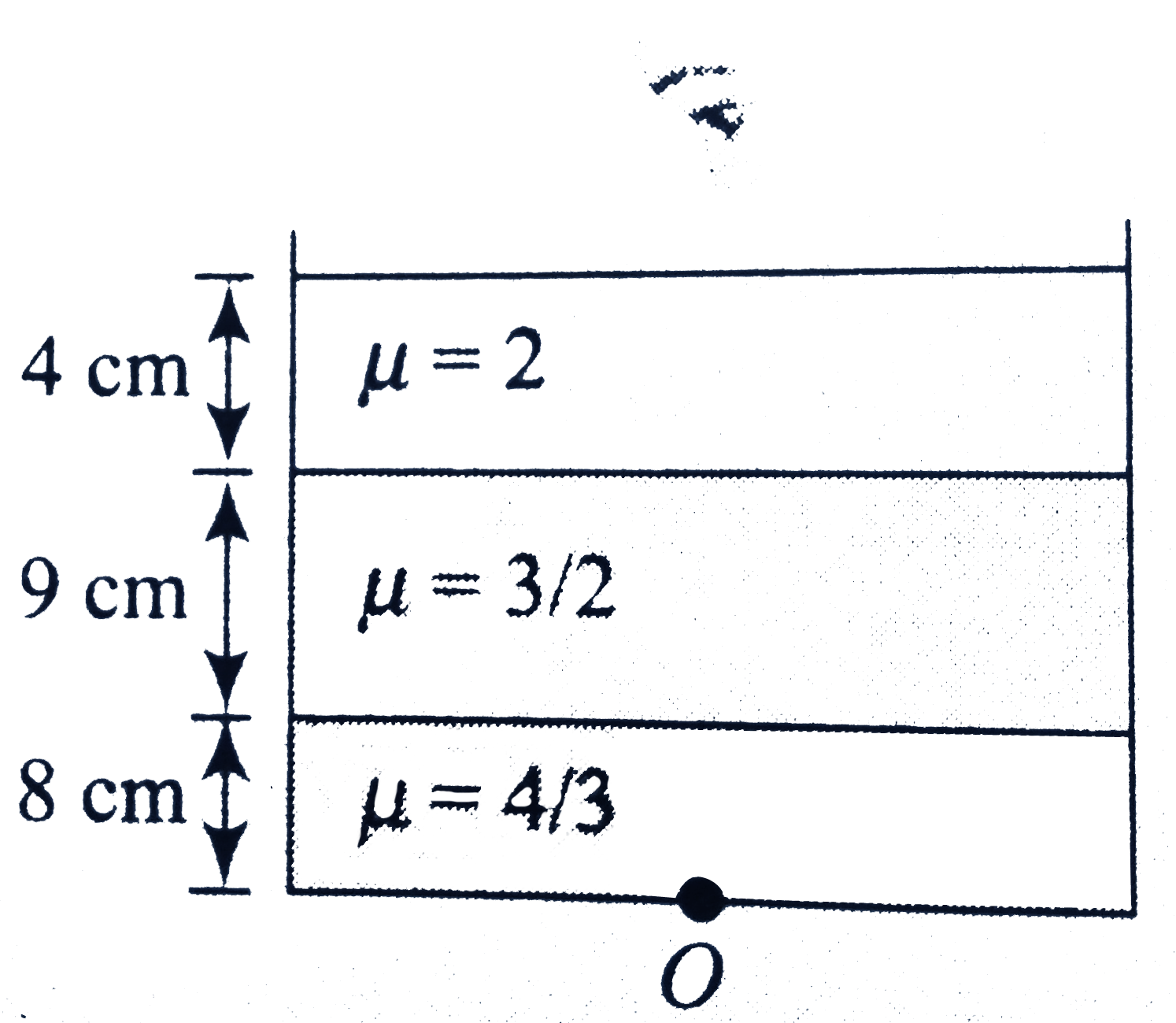
View Solution
