Question

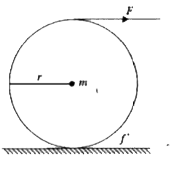
The mass of a spool of wire in the form of a uniform solid cylinder is m and its radius is r. The wire is unwound under a constant force F. Assume that the cylinder does not slip, find (i) the acceleration of the centre of mass, (ii) the force of friction, (iii) what is the speed attained by the centre of mass after the cylinder has rolled through a distance, assume that the cylinder starts from rest and it rolls without slipping ?

Explanation

Mass of the cylinder =m
Radius =r
Force = F
Due to the external force applied F, the cylinder starts rotating in the clockwise direction. The force of friction/acts towards right as shown in Fig. 7.2.53. For the linear motion of the centre of mass, if a is its acceleration, then,
`F +f = ma`......(i)
The torque causing rotational motion is:
`tau = I alpha`
`I =1/2 mr^(2), alpha = a/r`
`Fr - fr =1/2 mr^(2) xx a/r`
`F -f = (ma)/2`......(ii)
From eqns. (i) and (ii)
`2F =ma + (ma)/2 = (3ma)/2, a = (4F)/(3m)`
Substituting the value of a in equation (i)
`F + f = m xx (4F)/(3m)`
`f = (4F)/3 - F = F/3`
`v^(2) = u^(2) + 2as, v^(2) = 2 xx (4F)/(3m) xx s, v = sqrt((8Fs)/(3m))`