In a hydraulic machine, the two pistons are of area of cross section in the ratio 1:10. What weight force in needed on the narrow piston to overcome a force of 100 N on the wide piston?
To solve the problem, we will use Pascal's law, which states that the pressure applied to an enclosed fluid is transmitted undiminished throughout the fluid.
1. Identify the Given Information:
- The force on the wide piston (F1) is given as 100 N.
- The ratio of the areas of the two pistons (A2/A1) is given as 1:10.
2. Understand the Relationship Between Forces and Areas:
- According to Pascal's law, the pressure exerted on both pistons is equal. Therefore, we can write the equation:
F2A2=F1A1
- Rearranging this gives us:
F2=F1×A2A1
3. Substitute the Area Ratio:
- We know from the problem that A2A1=110.
- Substitute this into the equation:
F2=F1×110
4. Substitute the Value of F1:
- Now substitute F1=100N:
F2=100×110
5. Calculate F2:
- Performing the calculation:
F2=100÷10=10N
6. Conclusion:
- The weight force needed on the narrow piston (F2) to overcome the force of 100 N on the wide piston is 10 N.
Final Answer:
The weight force needed on the narrow piston is 10 N.
---
- The force on the wide piston (F1) is given as 100 N.
- The ratio of the areas of the two pistons (A2/A1) is given as 1:10.
- According to Pascal's law, the pressure exerted on both pistons is equal. Therefore, we can write the equation:
F2A2=F1A1
- Rearranging this gives us:
F2=F1×A2A1
- We know from the problem that A2A1=110.
- Substitute this into the equation:
F2=F1×110
- Now substitute F1=100N:
F2=100×110
- Performing the calculation:
F2=100÷10=10N
- The weight force needed on the narrow piston (F2) to overcome the force of 100 N on the wide piston is 10 N.
The weight force needed on the narrow piston is 10 N.
Topper's Solved these Questions
- FLUIDSBOOK - ICSECHAPTER - FLUIDSEXERCISE - TOPIC 1 (4 Marks Questions )
10 Videos
- FLUIDSBOOK - ICSECHAPTER - FLUIDSEXERCISE - TOPIC 2 (2 Marks Questions )
45 Videos
- FLUIDSBOOK - ICSECHAPTER - FLUIDSEXERCISE - TOPIC 2 (4 Marks Questions )
16 Videos
- ELECTRICITY AND MAGNETISM BOOK - ICSECHAPTER - ELECTRICITY AND MAGNETISM EXERCISE - TOPIC 2 Magnetism (4 Marks Questions )
6 Videos
- HEAT AND ENERGYBOOK - ICSECHAPTER - HEAT AND ENERGYEXERCISE - EXERCISE -6(D) Multiple Choice Question)
4 Videos
Similar Questions
In a hydraulic machine, a force of 2 N is applied on the piston of area of cross section 10cm2 . What force is obtained on its piston of area of cross section 100cm2 ?
View SolutionIn an arrangement similar to a hydraulic lift, the diameters of smaller and larger pistons are 1.0 cm and 3.0 cm respectively. What is the force exerted on the larger piston when a force of 10 N is applied to the smaller piston?
View SolutionIn a hydraulic lift, used at a service station the radius of the large and small piston are in the ration of 20:1. What weight placed on the small piston will be sufficient to lift a car of mass 1500kg ?
View SolutionIn a hydraulic lift at a service station, the radii of the large and small piston are in the ratio of 20 : 1. What weight placed on the small piston will be sufficient to lift a car of mass 1200 kg ?
View SolutionTwo pistons of a hydraulic machine have diameters of 30.0 cm and 2.5 cm. What is the force exerted on the larger piston when 40 kg wt. is placed on the smaller piston? If the smaller piston moves in through 6 cm, how much does the other piston move out ?
View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionFigure shown a hydraulic press with the larger piston if diameter 35 cm at a height of 1.5 cm at a height of 1.5 m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20 kg. What is the force exerted on the load by the larger piston? The density of oil in the press is 750kh/m3 . (Take g=9.8m/s2)
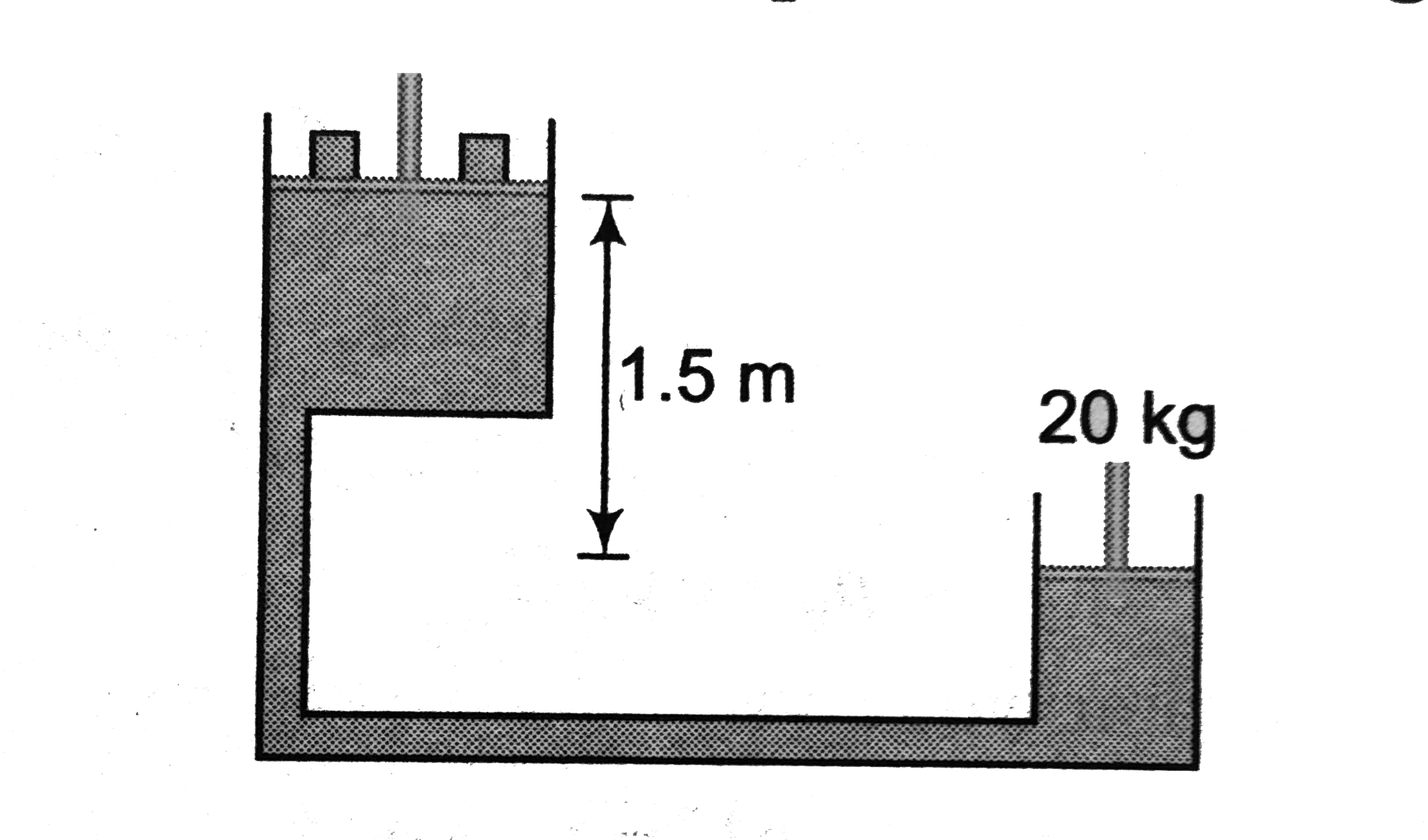 .
.
View SolutionWhat force is applied on a piston of area of cross section 2cm2 to obtain a force 150 N on the piston of area of cross section 12cm2 in a hydraulic machine ?
View SolutionTwo syringes of different cross-sections (without needles) filled with water are connected with a tightly fitted rubber tube filled with water. Diameters of the smaller piston and larger piston are 1.0cm and 3.0 cm respectively.
(a) Find the force on the larger piston when a force of 10N is applied to the smaller piston.
(b) The smaller piston is paused in through 6.0 cm, much does the larger piston move out?
View Solution
