If AP, BQ and CR are the altitudes of acute △ABCand9AP+4BQ+7CR=0 ∠ABC is equal to
a. cos−1(2)√7
b. π2
c. cos−1(√73)
d. π3
The correct Answer is:A
To solve the problem, we need to find the angle ∠ABC given the equation 9AP+4BQ+7CR=0, where AP,BQ, and CR are the altitudes of triangle ABC.
1. Understanding the Equation:
The equation 9AP+4BQ+7CR=0 implies a relationship between the altitudes of triangle ABC. Since the altitudes are directed towards the opposite vertices, we can interpret this as a vector equation.
2. Setting Up the Triangle:
Let AP=ha, BQ=hb, and CR=hc. The equation can be rewritten as:
9ha+4hb+7hc=0
This suggests that the altitudes are proportional to the sides of the triangle.
3. Using the Area of Triangle:
The area A of triangle ABC can be expressed using any of the altitudes:
A=12×BC×ha=12×AC×hb=12×AB×hc
From this, we can derive relationships between the sides and the altitudes.
4. Setting Ratios:
From the equation 9ha+4hb+7hc=0, we can express the ratios of the sides:
ha9=hb4=hc7
Let k be a constant such that:
ha=9k,hb=4k,hc=7k
5. Finding the Sides:
Using the area relationships:
A=12×BC×ha=12×AC×hb=12×AB×hc
We can express the sides a,b,c as:
a=7k,b=9k,c=4k
6. Using the Cosine Rule:
To find ∠ABC, we can use the cosine rule:
cosB=a2+c2−b22ac
Substituting the values:
a=7k,b=9k,c=4k
cosB=(7k)2+(4k)2−(9k)22⋅(7k)(4k)
Simplifying:
cosB=49k2+16k2−81k256k2=−16k256k2=−27
7. Finding the Angle:
Now, we find B:
B=cos−1(−27)
Final Answer:
Thus, the angle ∠ABC is given by:
∠ABC=cos−1(−27)
The equation 9AP+4BQ+7CR=0 implies a relationship between the altitudes of triangle ABC. Since the altitudes are directed towards the opposite vertices, we can interpret this as a vector equation.
Let AP=ha, BQ=hb, and CR=hc. The equation can be rewritten as:
9ha+4hb+7hc=0
This suggests that the altitudes are proportional to the sides of the triangle.
The area A of triangle ABC can be expressed using any of the altitudes:
A=12×BC×ha=12×AC×hb=12×AB×hc
From this, we can derive relationships between the sides and the altitudes.
From the equation 9ha+4hb+7hc=0, we can express the ratios of the sides:
ha9=hb4=hc7
Let k be a constant such that:
ha=9k,hb=4k,hc=7k
Using the area relationships:
A=12×BC×ha=12×AC×hb=12×AB×hc
We can express the sides a,b,c as:
a=7k,b=9k,c=4k
To find ∠ABC, we can use the cosine rule:
cosB=a2+c2−b22ac
Substituting the values:
a=7k,b=9k,c=4k
cosB=(7k)2+(4k)2−(9k)22⋅(7k)(4k)
Simplifying:
cosB=49k2+16k2−81k256k2=−16k256k2=−27
Now, we find B:
B=cos−1(−27)
Thus, the angle ∠ABC is given by:
∠ABC=cos−1(−27)
Topper's Solved these Questions
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - JEE Type Solved Examples: Passage Based Type Questions
3 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise For Session 1
12 Videos
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
54 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
Similar Questions
If AP, BQ and CR are the altitudes of acute △ABCand9AP+4BQ+7CR=0 Q. ∠ACB is equal to
View SolutionIn Figure 7. find the perimeter of △ABC, if AP = 12 cm
View SolutionP,Q,R are the points on the sides AB, BC and CA respectively of triangle ABC such that AP:PB=BQ:QC=AR:RC=1:2. Show that PBQR is a parallelogram.
View SolutionStatement- 1: If the sines of the angles of a triangle are in A.P., then the altitudes ef the triangle are also in A.P.
Statement-2: Twice the area of a triangle is equal to the product of the lengths of a side and the altitude on it.
View SolutionIn △ABC,P is any point inside a triangle such that area of △APB,△BPC, △CPA are equal.If the line AP cuts ¯¯¯¯¯¯BC at M such that area of △PMC is 4.5 sq units.Then area of △ABC is
View SolutionAP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (in the given figure). Show that AP||BQ.
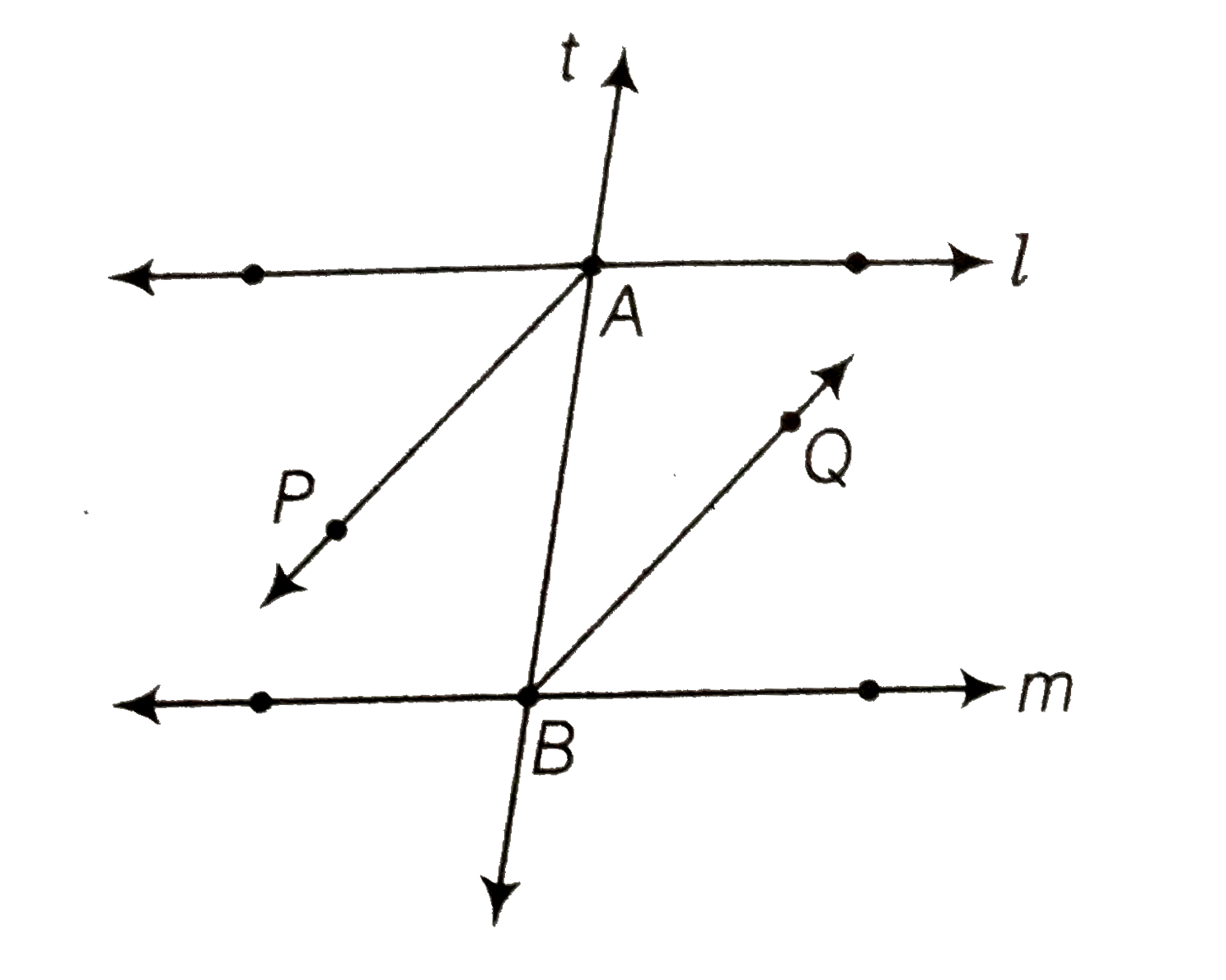
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PQ = QR
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PB = QC
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
If angle PQR is a rt. Angle find angle PRQ
View SolutionThe points A (x1,y1),B(x2,y2)andC(x3,y3) are the vertices of △ABC.
(i) The median from A Meets Bc at D. Find the coordinates of the points D.
(ii) Find the coordinates of the point P on Ad such that AP:PD=2:1.
(iii) Find the coordinates of points Q and R on medians BE and CF, respectively such that BQ:QE=2:1andCR:RF=2:1.
What are the coordinates of the centroid of the △ ABC?
View Solution
