Let p, q, r be three mutually perpendicular vectors of the same magnitude. If a vector x satisfies the equation p x ((x-q) x p) + q x ((x-r) x q) + r x ((x-p) x r)=0 Then x is given by :
The correct Answer is:x=a+b+c2
To solve the problem, we start with the equation given in the question:
p×x+(x−q)×p+q×(x−r)×q+r×(x−p)×r=0
Step 1: Rewrite the equation
We can rewrite the equation as follows:
p×x+(x×p−q×p)+(q×x−r×q)+(r×x−p×r)=0
Step 2: Combine like terms
Now, we can combine the terms involving x:
(p×x+x×p+q×x+x×q+r×x+x×r)−(q×p+r×q+p×r)=0
Step 3: Factor out x
Notice that p×x+x×p can be simplified, as can the other terms. Thus, we can factor out x:
(p+q+r)×x−(q×p+r×q+p×r)=0
Step 4: Solve for x
From the equation above, we can isolate x:
(p+q+r)×x=q×p+r×q+p×r
Step 5: Express x
Now, we can express x:
x=(q×p+r×q+p×r)(p+q+r)
Step 6: Recognize the vectors are mutually perpendicular
Since p, q, and r are mutually perpendicular vectors of the same magnitude, we can denote their magnitudes as k. Thus, we have:
|p|=|q|=|r|=k
Step 7: Final expression for x
Given that p, q, and r are mutually perpendicular, we can conclude that:
x=p+q+r
Final Answer
Thus, the vector x is given by:
x=p+q+r
---
We can rewrite the equation as follows:
Now, we can combine the terms involving x:
Notice that p×x+x×p can be simplified, as can the other terms. Thus, we can factor out x:
From the equation above, we can isolate x:
Now, we can express x:
Since p, q, and r are mutually perpendicular vectors of the same magnitude, we can denote their magnitudes as k. Thus, we have:
Given that p, q, and r are mutually perpendicular, we can conclude that:
Thus, the vector x is given by:
Topper's Solved these Questions
- PRODUCT OF VECTORSBook:ARIHANT MATHS ENGLISHChapter:PRODUCT OF VECTORSExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 51 Videos
- PRODUCT OF VECTORSBook:ARIHANT MATHS ENGLISHChapter:PRODUCT OF VECTORSExercise:Exercise (Single Integer Answer Type Questions)
Explore 15 Videos
- PROBABILITYBook:ARIHANT MATHS ENGLISHChapter:PROBABILITYExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 54 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES Book:ARIHANT MATHS ENGLISHChapter:PROPERTIES AND SOLUTION OF TRIANGLES Exercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 21 Videos
Similar Questions
Knowledge Check
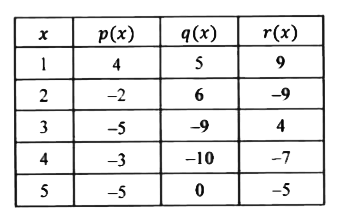
The table abvoe gives some value of the functions p, q, and r. At which value x does q(x)=p(x)+r(x)?A1B2C3D4
If p,q,r,s∈R, then equaton (x2+px+3q)(−x2+rx+q)(−x2+sx−2q)=0 has
View SolutionIf p,q,rands are real numbers such that pr=2(q+s), then show that at least one of the equations x2+px+q=0 and x2+rx+s=0 has real roots.
View Solution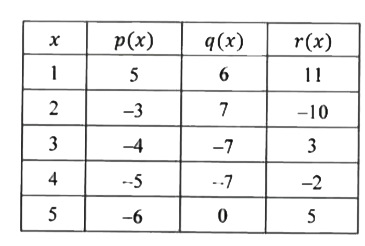
The table gives some values of the functions p, q and r. At which value of x does q(x)=p(x)+r(x)?
View SolutionFor p, q∈R, the roots of (p2+2)x2+2x(p+q)−2=0 are
View SolutionProve that pxq−r+qxr−p+rxp−q>p+q+r,wherep,q,r are distinct and x≠1.
View SolutionIf P={x∈R:f(x)=0} and Q={x∈R:g(x)=0}, then P∪Q is
View SolutionLet p,q∈R. If 2−√3 is a root of the quadratic equation, x2+px+q=0, then
View Solution