The torque acting on a body is the rotational, analogue of
mass of the body
linear kinetic energy of the body
linear velocity of the body
force in linear motion
The correct Answer is:D
To solve the question, we need to understand the relationship between torque, power, and their analogs in rotational and translational motion.
1. Understanding Torque:
Torque (τ) is a measure of the rotational force that causes an object to rotate about an axis. It is defined as τ = r × F, where r is the distance from the axis of rotation to the point where the force is applied, and F is the force applied.
Hint: Recall that torque depends on both the magnitude of the force and the distance from the pivot point.
2. Power in Rotational Motion:
Power (P) in rotational motion is defined as the rate at which work is done. The work done by torque can be expressed as the integral of torque over angular displacement (θ). Mathematically, this is given by:
P=dWdt=ddt(τ⋅θ)
Hint: Remember that power is the derivative of work with respect to time.
3. Relating Work Done to Angular Displacement:
The work done by torque can be expressed as:
W=∫τ⋅dθ
Therefore, differentiating this with respect to time gives us the power:
P=τ⋅dθdt
Hint: Recognize that dθdt is the angular velocity (ω).
4. Final Expression for Power:
Substituting dθdt with angular velocity (ω), we get:
P=τ⋅ω
This shows that the power associated with torque is indeed the product of torque and angular speed.
Hint: This relationship mirrors the translational power equation P=F⋅v.
5. Translational Analog:
In translational motion, power is given by the product of force (F) and velocity (v):
P=F⋅v
Here, force is the translational analog of torque, and velocity is the translational analog of angular velocity.
Hint: Compare the forms of the equations for rotational and translational power to see the analogs.
Conclusion:
The torque acting on a body is the rotational analog of force in translational motion, and the power associated with torque is given by the product of torque and angular speed, paralleling the relationship in linear motion.
Torque (τ) is a measure of the rotational force that causes an object to rotate about an axis. It is defined as τ = r × F, where r is the distance from the axis of rotation to the point where the force is applied, and F is the force applied.
Power (P) in rotational motion is defined as the rate at which work is done. The work done by torque can be expressed as the integral of torque over angular displacement (θ). Mathematically, this is given by:
P=dWdt=ddt(τ⋅θ)
The work done by torque can be expressed as:
W=∫τ⋅dθ
Therefore, differentiating this with respect to time gives us the power:
P=τ⋅dθdt
Substituting dθdt with angular velocity (ω), we get:
P=τ⋅ω
This shows that the power associated with torque is indeed the product of torque and angular speed.
In translational motion, power is given by the product of force (F) and velocity (v):
P=F⋅v
Here, force is the translational analog of torque, and velocity is the translational analog of angular velocity.
The torque acting on a body is the rotational analog of force in translational motion, and the power associated with torque is given by the product of torque and angular speed, paralleling the relationship in linear motion.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - GRAVITATION25videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - PROPERTIES OF MATTER (ELASTICITY )22videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - UNIFORM CIRCULAR MOTION25videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)24videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)9videos
DIMENSIONS
Similar Questions
Assertion:The couple acting on a body is not equal to the rotational KE of the body
Reason: Couple and KE have different dimensionsView SolutionWhat is the torque acting on a body moving with constant angular velocity ?
View SolutionWhat is the torque acting on a body moving with constant angular velocity ?
View SolutionIf the torque on a body is zero, then?
View SolutionShow that the torque acting on a body is equal to the product of moment of inertia and the angular acceleration of the body.
View SolutionIf the force acting on a body is inversely proportional to its speed, the kinetic energy of the body is
View SolutionFind the charge on the capacitor shown in figure
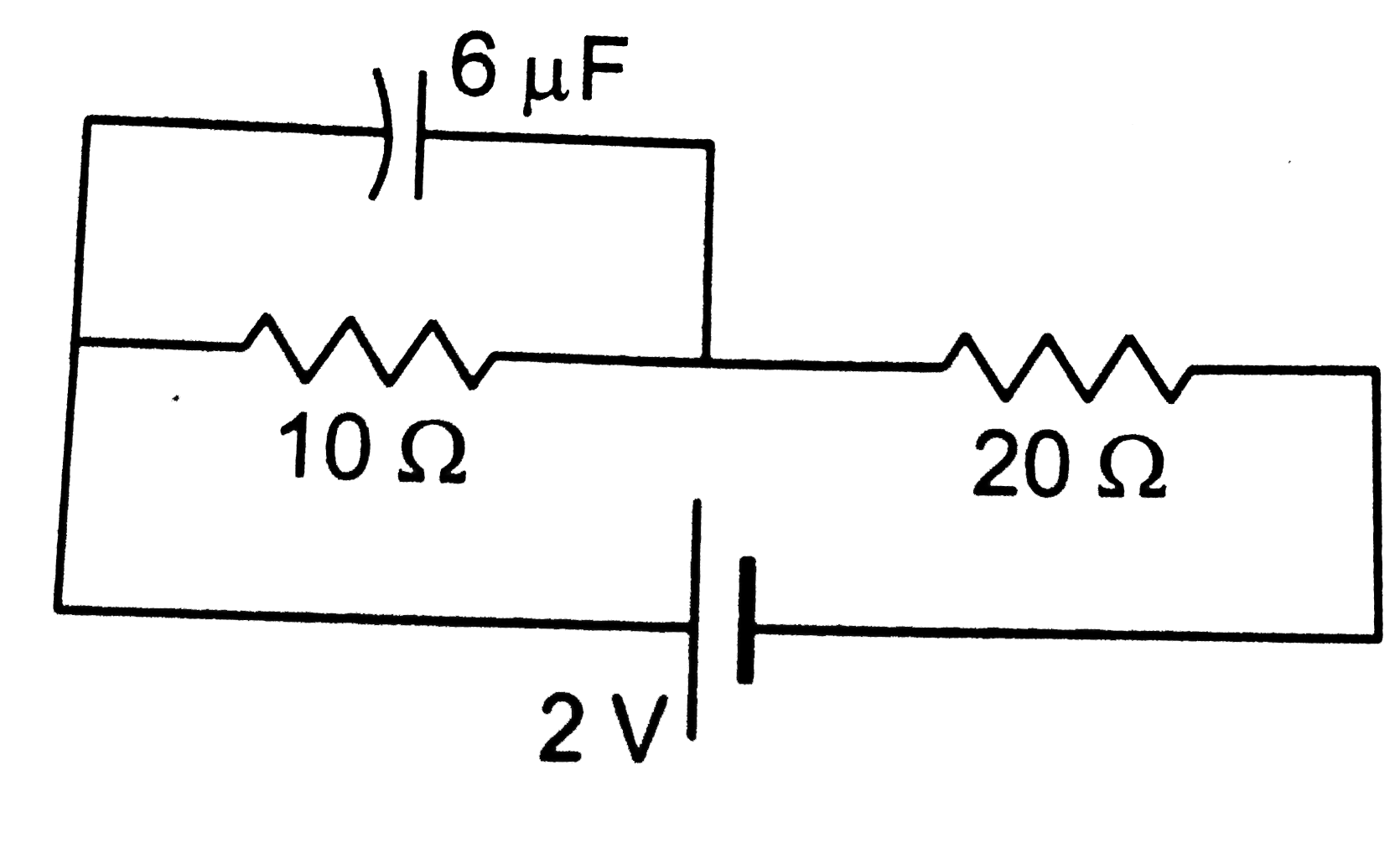 View Solution
View SolutionIf the torque acting on a system is zero then, which of the followin quantities is conserced ?
View SolutionCan you calculate the torque acting on a rigid body without specifying a centre of rotation ?
View SolutionIf the resultant torque of all the forces acting on a body is zero about a point is it necessary that it will be zero about any other point?
View Solution

