A block of mass m is on an inclined L plane of angle 0. The coefficient of M, friction between the block and the plane is $μ and tan θ > μ$ . The block is e held stationary by applying a force P parallel to the plane. The direction of force pointing up the plane is taken to be positive. As P is varied from $P_{1} = m g (sin 0 - μ cos θ) to P_{2} = m g (sin θ + μ cos θ)$ , the frictional force f versus P graph will look like

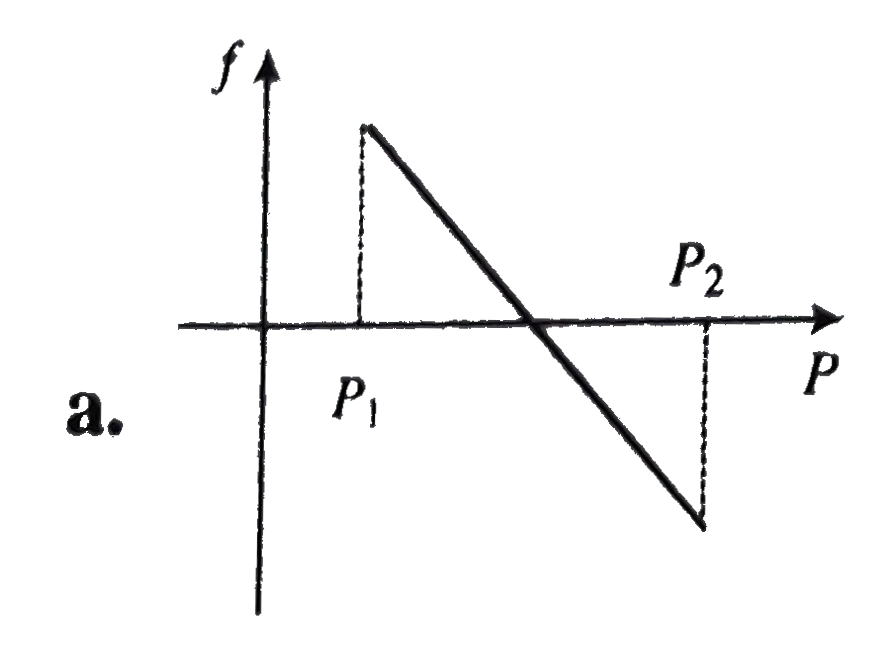
ocr_image

Video Solution

Text Solution

Verified by Experts

The correct Answer is:a

A block of mass m is on an inclined plane of angle $θ$ . The coefficient of friction between the block and the plane is $μ$ and $tan θ > μ$ . The block is held stationary by applying a force P parallel to the plane. The direction of force pointing up the plane is taken to be positive. As P is varied from $P_{1} = m g (sin θ - μ cos θ)$ to $P_{2} = m g (sin θ + μ cos θ)$ , the frictional force f versus P graph will look like
A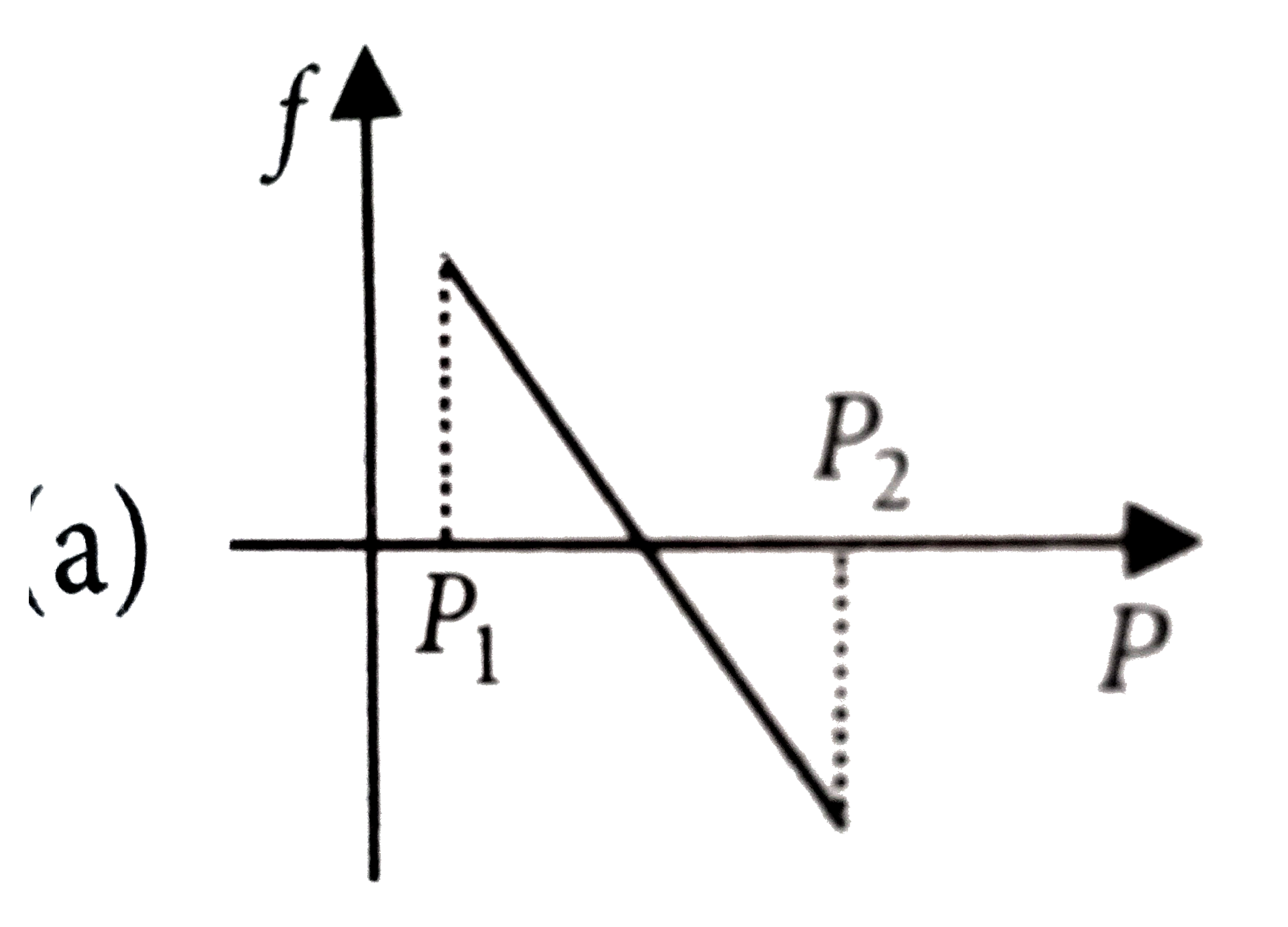
B
C
D