A small ball of mass 1kg is kept in circular path of radius 1m Inside a concentric smooth horizontal fixed casing of radius R. Angular speed of the ball in the circular motion is 1rads−1. At a certain moment the string, which kept the ball in the circular path breaks and the ball goes off tangentially to the wall of rigid casing and bounces off elastically and again hits the casing and bounces off. This way, the ball traces a regular hexagon. Consider all the collisions to be elastic.
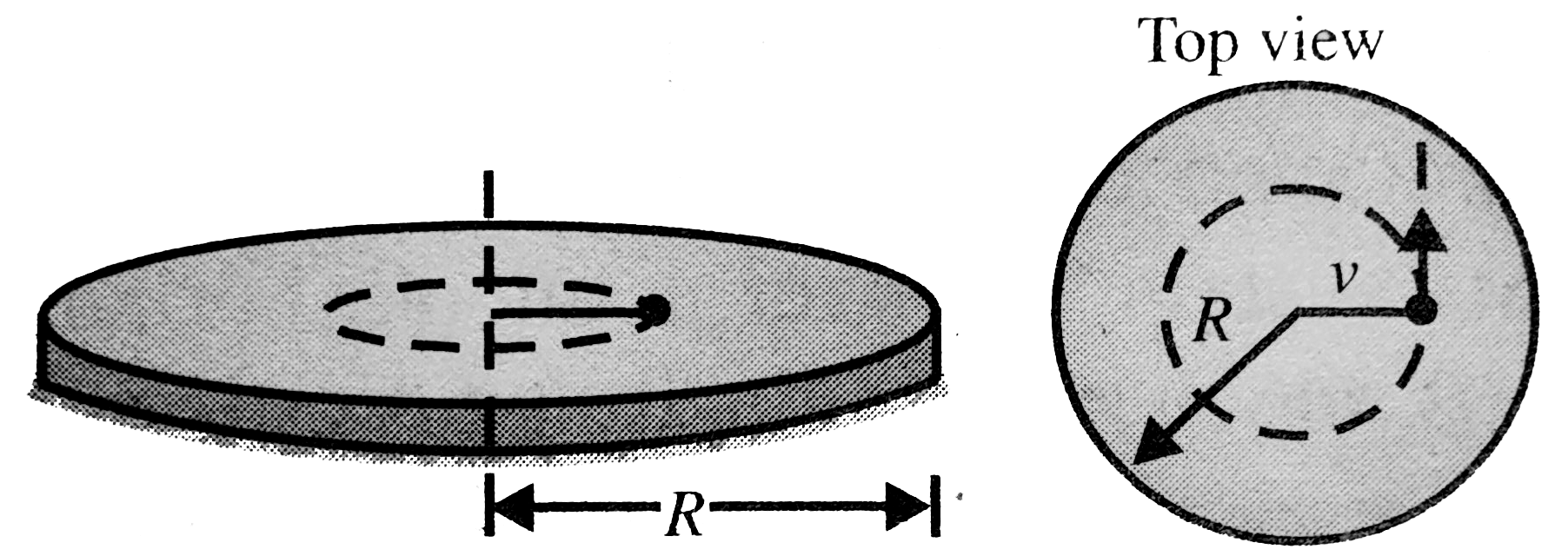
Following quantities of the ball will remain a constant before and after any collision
linear momentum
kinetic energy, angular momentum about the centre of the circle
velocity, angular momentum about the centre of the circle, kinetic energy
none of these
The correct Answer is:B
Since an impulse acts on the ball during each collision, so linear momentum does not remain constant.
The impulsive force is directed towards the centre of hexagon, therefore the lever arm of this imupulsive force is zero.
As are result, it does not produce any torque and angular momentum remains costant. Further, kinetic energy also remains same for elastic collision.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Integer
16 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Fill In The Blanks
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A small ball of mass 1kg is kept in circular path of radius 1m Inside a concentric smooth horizontal fixed casing of radius R. Angular speed of the ball in the circular motion is 1rads−1. At a certain moment the string, which kept the ball in the circular path breaks and the ball goes off tangentially to the wall of rigid casing and bounces off elastically and again hits the casing and bounces off. This way, the ball traces a regular hexagon. Consider all the collisions to be elastic.
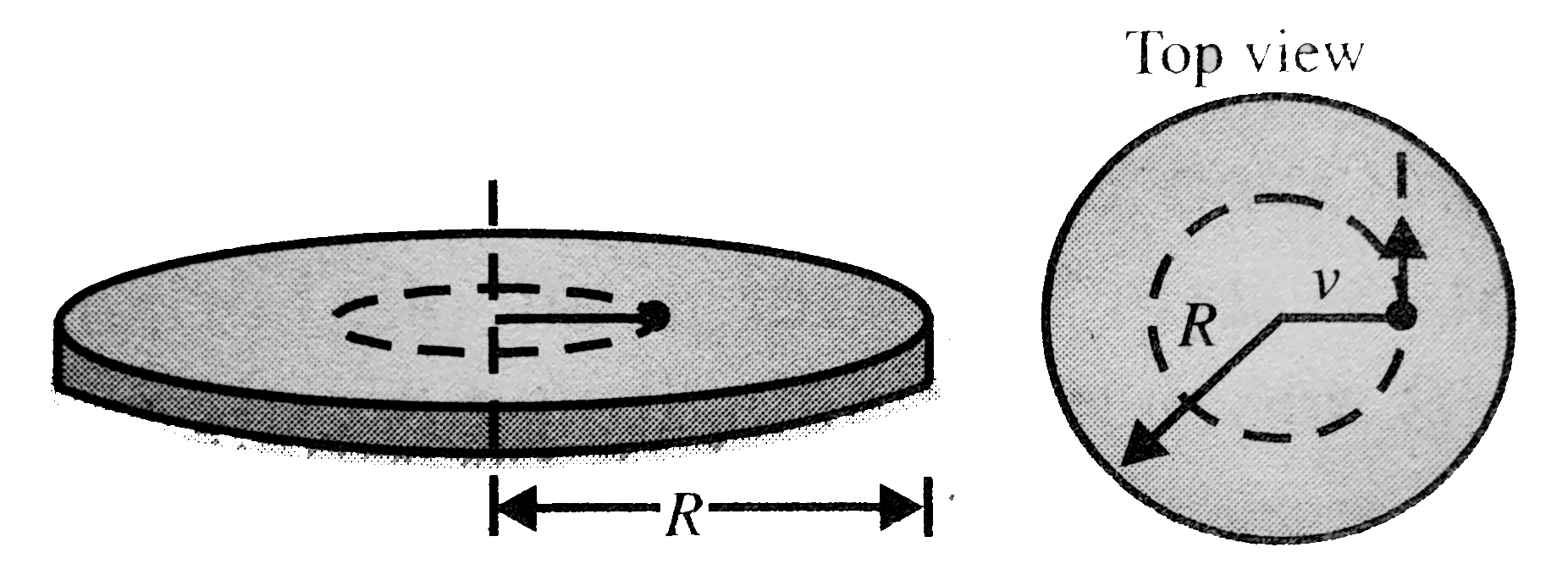
Total time between the first collision and the seventh collision will
View SolutionA particle in a circular motion covers a distance of 0.5 πr where r is the radius of the circular path. What is the angular displacement ?
View SolutionA ball of mass m=1kg strikes a smooth horizontal floor as shown in figure. The impulse exerted on the floor is

View SolutionA car of maas M is moving on a horizontal circular path of radius r. At an instant its speed is v and is increasing at a rate a.
View SolutionA satellite of mass m goes round the earth along a circular path of radius r. Let mE be the mass of the earth and RE its radius then the linear speed of the satellite depends on.
View SolutionA particle is revolving in a circular path of radius 2 m with constant angular speed 4 rad/s. The angular acceleration of particle is
View Solution
A ball of mass m and radius r rolls along a circular path of radius R its speed at the bottom (θ=0∘) of the path is v0 find the force of the path on the ball as a function of θ.
View SolutionA particle is moving around a circular path with uniform angular speed (x) . The radius of the circular path is (r). The acceleration of the particle is:
View SolutionA particle of mass 2 kg is moving along a circular path of radius 1 m. If its angular speed is 2π rad s−1, the centripetal force on it is
View SolutionA ball of mass 'm' is rotating in a circle of radius 'r' with speed v inside a smooth cone as shown in figure. Let N be the normal reaction on the ball by the cone .then choose the wrong option.
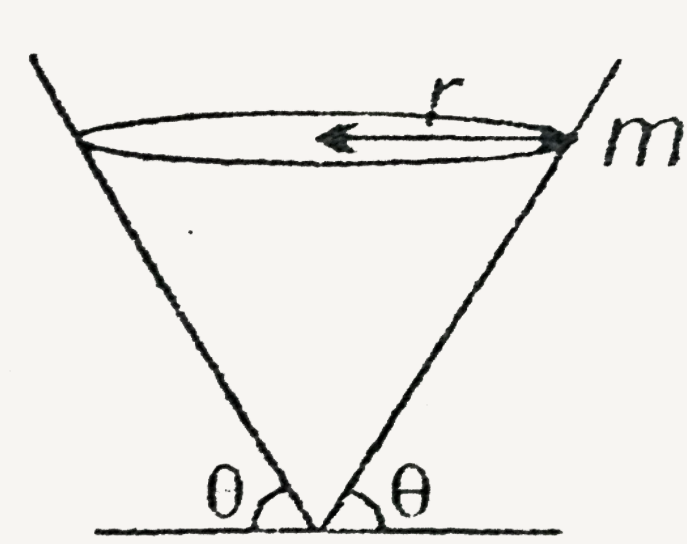
View Solution
