A weightless rod of length $l$ with a small load of mass $m$ at the end is hinged at point $A$ as shown and occupies a strictly vertical position, touching a body of mass $M$ . A light jerk sets the system in motion.
a. For what mass ratio $M / m$ will the rod form an angle $α = π / 6$ with the horizontal at the moment of the separation from the body?
b. What will he the velocity $u$ of the body at this moment? Friction should be neglected.

Video Solution

Text Solution

Verified by Experts

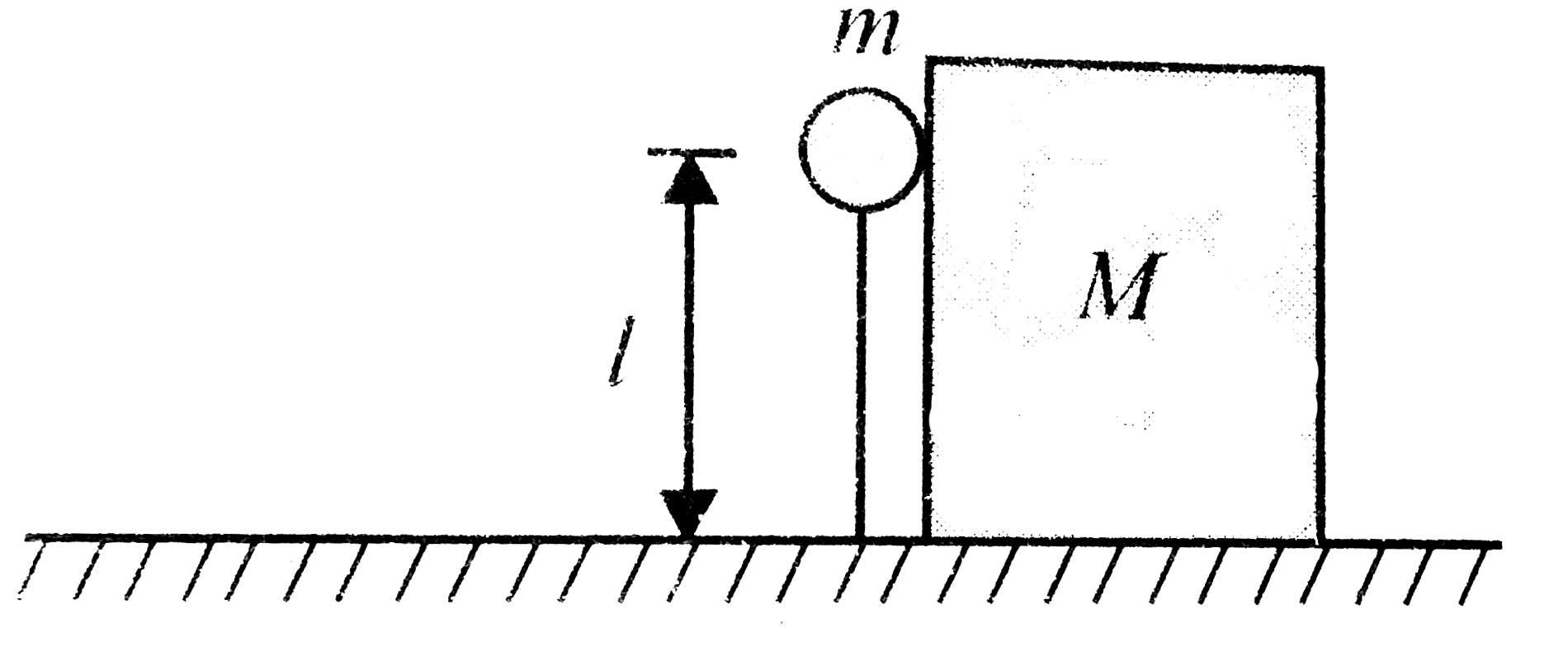
The correct Answer is: $\sqrt{\frac{g l}{2}}$

$a = a_{t} sin α - a_{c} cos α$
At the time of separation
For $M, Σ F_{x} = 0$ and $a = 0$

Acceleratin of the load in horizontal direction
$a = 0 = a_{1} sin α - \frac{v^{2}}{l} cos α$
$a_{t} sin α = \frac{v^{2}}{l} cos α$
But $a_{t} = g cos α$
Hence $v = \sqrt{g l sin α}$
$u = v sin α = sin α \sqrt{g l sin α}$
$m g l = m g l sin α + \frac{m v^{2}}{2} + \frac{1}{2} M v^{2} sin α$
$\frac{M}{m} = \frac{2 - 3 sin α}{{sin}^{3} α} = 4$
$u = v sin α = \frac{1}{2} \sqrt{\frac{g l}{2}}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-RIGID BODY DYNAMICS 1-Subjective

Home
Ask Doubt
Profile