A partical having charge that of an electron and mass 1.6×10−30kg is projected with an initial speed u at the angle 45∘ to the horizontal from the lower plate of a parallel-plate capacitor as shown in fig. The plates are suffiecienly long and have a separation of 2 cm, find the maximum value of the velocity of he particle so that it does not hit the upper plate. Take the electric field between the plates as 103Vm−1 directed upward.

Resolving the velosity of the particle parallel and perpendicular to the plate, we get
u∣∣=ucos45∘=u√2andu⊥=usin45∘=u√2
force on the charged particle in the downward direction and normal to the plate is eE. Therefore, acceleration a=eE/m, where m is the mass of the charged paricle. the particle will not hit the upper plate if the velocity component normal to hte plate becomes zero before reaching it . i.e. 0=−u2⊥−2ay with y≤d, where d is the distnce between the plates. therefore, the maximum velocity for the particle not to hit the upper plate (for this y=d=2cm)is
u⊥=√2ay=√2×1.6×10−19×103×2×10−21.6×10−30
=2×106ms−1
or, umax=u⊥/cos45∘=2√2×106ms−1.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples10videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises58videos
COULOMB LAW AND ELECTRIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type51videos
CENGAGE PHYSICS DPP
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits15videos
ELECTRIC CURRENT & CIRCUITS
Similar Questions
A parallel plate capacitor is charged. If the plates are pulled apart
View SolutionConsider a parallel plate capacitor having charge Q. Then,
 View Solution
View SolutionFor a charged parallel plate capacitor shown in the figure, the force experienced by an alpha particle will be :
 View Solution
View SolutionThe plates of a parallel plate capacitor are not exactly parallel. The surface charge density is therefore,
View SolutionThe plates of a parallel plate capacitance 1.0 F are separated by a distance d =1 cm. Find the plate area .
View SolutionA parallel plate capacitor is charged and then isolated. On increasing the plate separation
View SolutionFind out the capacitance of parallel plate capacitor of plate area A and plate separation d.
View SolutionIf the separation between the plates of a capacitor is 5 mm , then area of the plate of a 3 F parallel plate capacitor is
View SolutionThe electrostatic force between the metal plate of an isolated parallel plate capacitor C having charge Q and area A, is
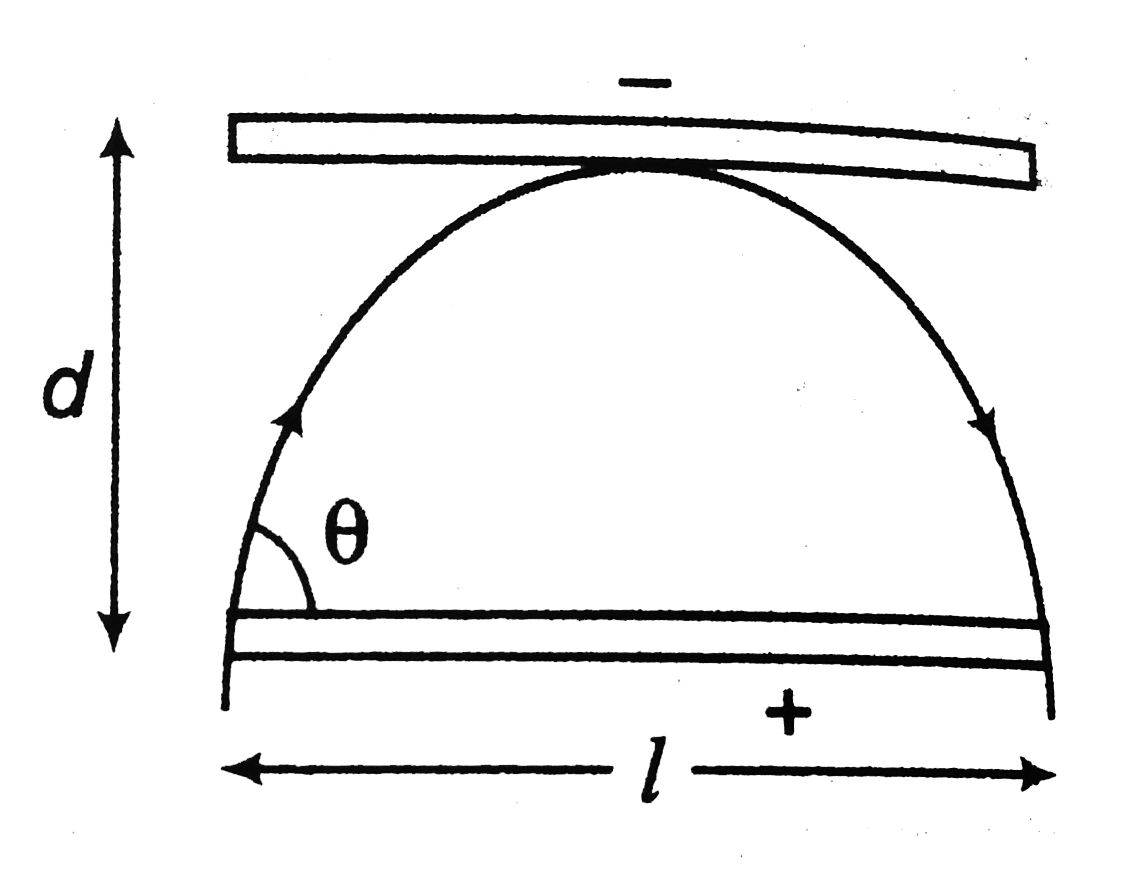
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at an angle θ to the plates. The plate width is l, the plate separation is d. The electron follows the path shown just missing the upper plate. Neglect gravity. Then,
 View Solution
View Solution
