An electric dipole is kept on the axis of a uniformly charged ring at distance from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is p, charge of the ring is Q & radius of the ring is R. The force on the dipole is
4kPQ3√3R2
4kPQ3√3R3
2kPQ3√3R3
zero
The correct Answer is:D
To find the force on an electric dipole placed on the axis of a uniformly charged ring, we can follow these steps:
Step 1: Understand the Configuration
We have a uniformly charged ring with total charge Q and radius R. An electric dipole with dipole moment p is placed along the axis of the ring at a distance d from its center. The dipole consists of two equal and opposite charges, +q and −q, separated by a small distance 2a.
Step 2: Electric Field Due to the Ring
The electric field E at a point along the axis of the ring (at distance d from the center) can be calculated using the formula:
E=14πϵ0⋅Qd(d2+R2)3/2
where ϵ0 is the permittivity of free space.
Step 3: Force on the Dipole
The force F on a dipole in an electric field is given by:
F=p⋅∇E
However, since the dipole is placed at a distance much larger than its length, the force on the dipole can be approximated by considering the forces acting on the individual charges +q and −q.
Step 4: Analyzing Forces on Charges
The charge +q experiences a force F+=+qE in the direction of the electric field, while the charge −q experiences a force F−=−qE in the opposite direction. Therefore, the net force on the dipole is:
Fnet=F++F−=+qE−qE=0
Step 5: Conclusion
Since the forces acting on the two charges of the dipole cancel each other out, the net force on the dipole is zero:
Fnet=0
Final Answer
The force on the dipole is F=0.
---
We have a uniformly charged ring with total charge Q and radius R. An electric dipole with dipole moment p is placed along the axis of the ring at a distance d from its center. The dipole consists of two equal and opposite charges, +q and −q, separated by a small distance 2a.
The electric field E at a point along the axis of the ring (at distance d from the center) can be calculated using the formula:
E=14πϵ0⋅Qd(d2+R2)3/2
where ϵ0 is the permittivity of free space.
The force F on a dipole in an electric field is given by:
F=p⋅∇E
However, since the dipole is placed at a distance much larger than its length, the force on the dipole can be approximated by considering the forces acting on the individual charges +q and −q.
The charge +q experiences a force F+=+qE in the direction of the electric field, while the charge −q experiences a force F−=−qE in the opposite direction. Therefore, the net force on the dipole is:
Fnet=F++F−=+qE−qE=0
Since the forces acting on the two charges of the dipole cancel each other out, the net force on the dipole is zero:
Fnet=0
The force on the dipole is F=0.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Multiple Correct Answer Type
1 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
Similar Questions
A small electric dipole is kept on the axis of a uniformly charged ring at distance R/√2 from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is P, charge of the ring is Q and radius of the ring is R. The force on the dipole is nearly _____
View SolutionA short dipole is placed on the axis of a uniformly charged ring (total charge -Q, radius R) at a distance R√2 from centre of ring as shown in figure. Find the Force on the dipole due to that ring

View SolutionThe maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be
View SolutionThe electric field of an electric dipole at a point on its axis , at a distance d from the centre of the dipole, varies as
View SolutionThe electric field at a point on equatorial of a dipole and direction of the dipole moment
View SolutionA dipole is kept near an infinite line of charge. If the net force on the dipole is zero, then its dipolse moment is
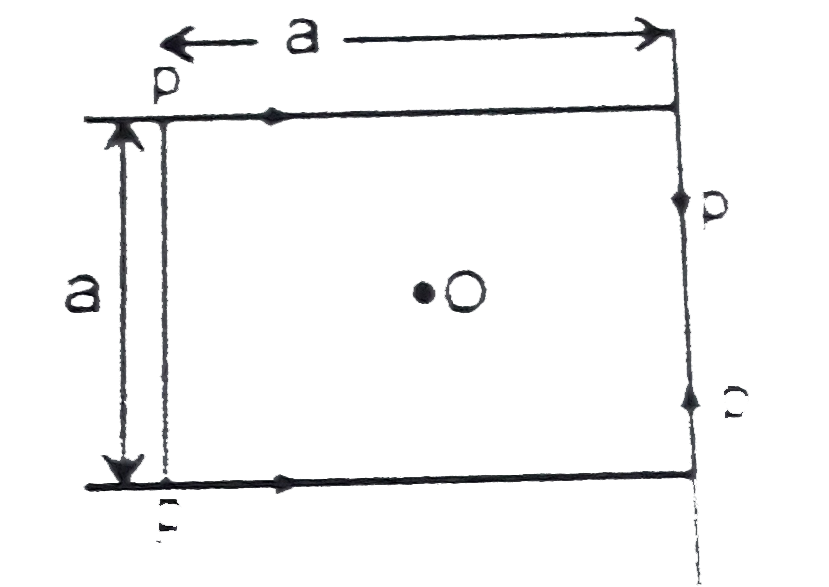
View SolutionFour short dipoles each of dipole moment 'p' are placed at the vertices of a square of side a. The direction of the dipole moments are shown in the figure.
View SolutionAlong the axis of a dipole, direction of electric field is always in the direction of electric dipole moment p. Is this statement true of false?
View SolutionTwo equal charges q of opposite sign separated by a distance 2a constitute an electric dipole of dipole moment p. If P is a point at a distance r from the centre of the dipole and the line joining the centre of the dipole to this point makes an angle θ with the axis of the dipole, then then potential at P is given by (r>>2a) where (p = 2 qa)
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View Solution
