This question concerna a symmetrical lens shown, along with its two focal points. It is made of plastice with n=1.2 and has focal length f. Four different regions are shown:
Here,
A. −∞x<−f B. −f<x<0
C. 0<x<f D. f<x<∞
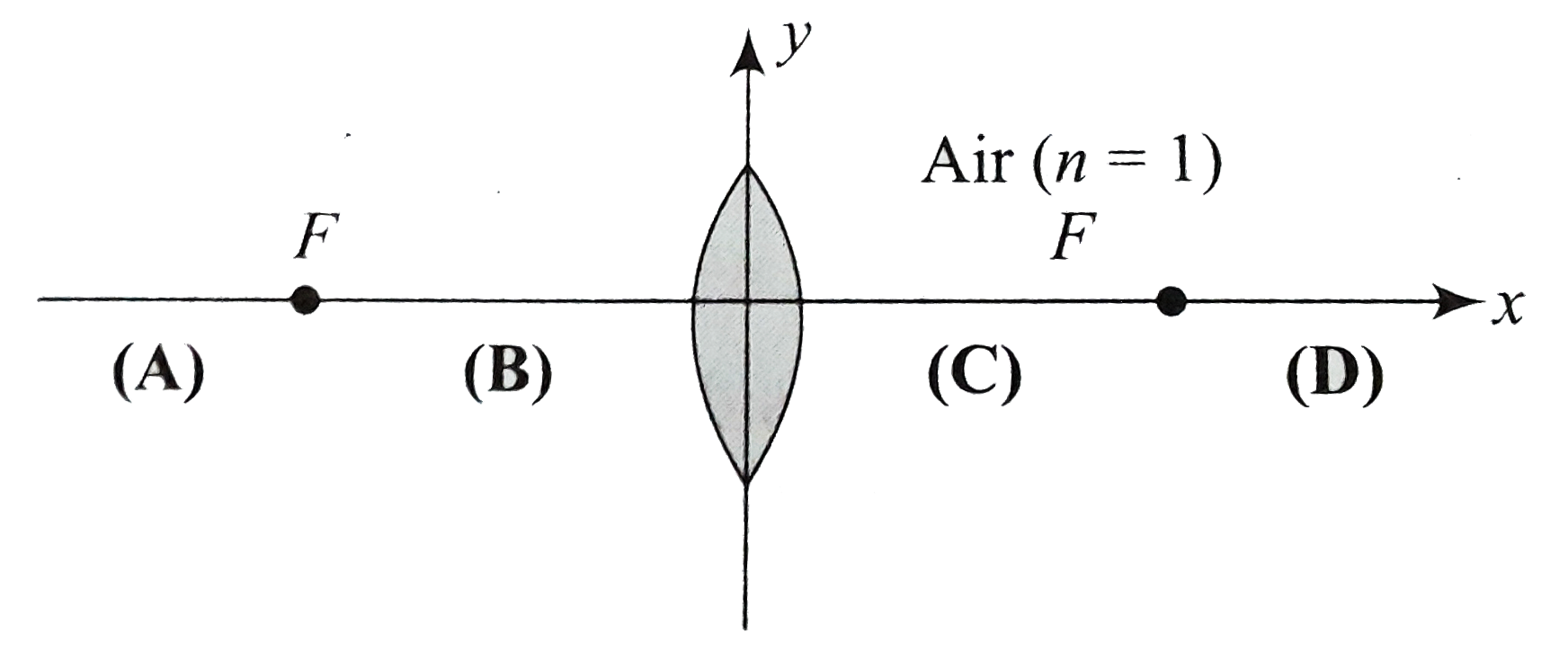
Q. If incident rays are converging, then in which region does the image appear?
A
B
C
D
The correct Answer is:d
From the formula of equivalent focal length of thin lenses in contact, we get
1F=1f1+1f2=1f−13forF=3f2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Integer
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Archives
7 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
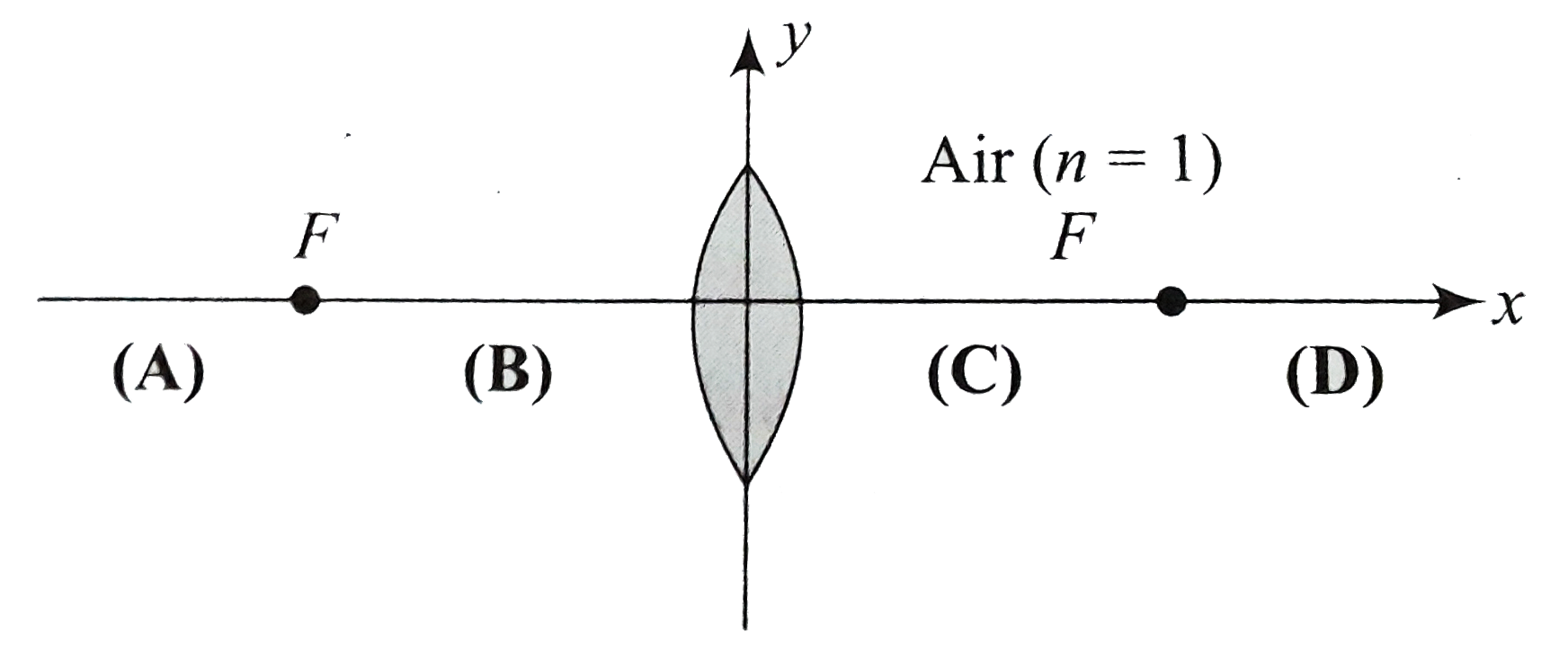
This question concerns a symmetrical lens shown, along with its two focal points. It is made of plastic with n=1.2 and has focal length f. Four different regions are shown:
A. −∞<x<−f
B. −f<x<0
C. 0<x<f
D. f<x<∞
A second lens is now placed adjacent to the first in contact with it. The new lens is concave, with a focal length f=−3f. In what region does the focal length of the two-lens system lie? Treat the lenses as thin.
View SolutionThe number of points at which f(x)=[x]+|1−x|,−1<x<3 is not differentiable is (where [.] denotes G.I.F)
View SolutionIdentify the null sets among the following
F = {x : x ∈N,5<x<6.}
View SolutionFind the inverse of each of the following functions :
f(x)=⎧⎪⎨⎪⎩x,−∞<x<1x2,1≤x≤42x,4<x<∞
View SolutionLet f(x)={a−3x−2≤x<04x±30≤x<1,iff(x) has smallest valueat x=0, then range of a, is
View SolutionLet f(x)={2−x,−3≤x≤0x−2,0<x<4 Then f−1 (x) is discontinous at x=
View SolutionThe function f(x)={x2a , if 0≤x<1 a , if 1≤x is continuous then find the value of constant term
View SolutionThe function f(x)={x2a , 0≤x<1, a, 1≤x is continuous then find the value of constant term
View SolutionLet f(x)=ax2+bx+c, a≠0, a, b, c∈I. Suppose that f(1)=0, 50<f(7)<60 and 70<f(8)<80.
Number of integral values of x for which f(x)<0 is
View SolutionLet f(x)=ax2+bx+c, a≠0, a, b, c∈I. Suppose that f(1)=0, 50<f(7)<60 and 70<f(8)<80.
The least value of f(x) is
View Solution
