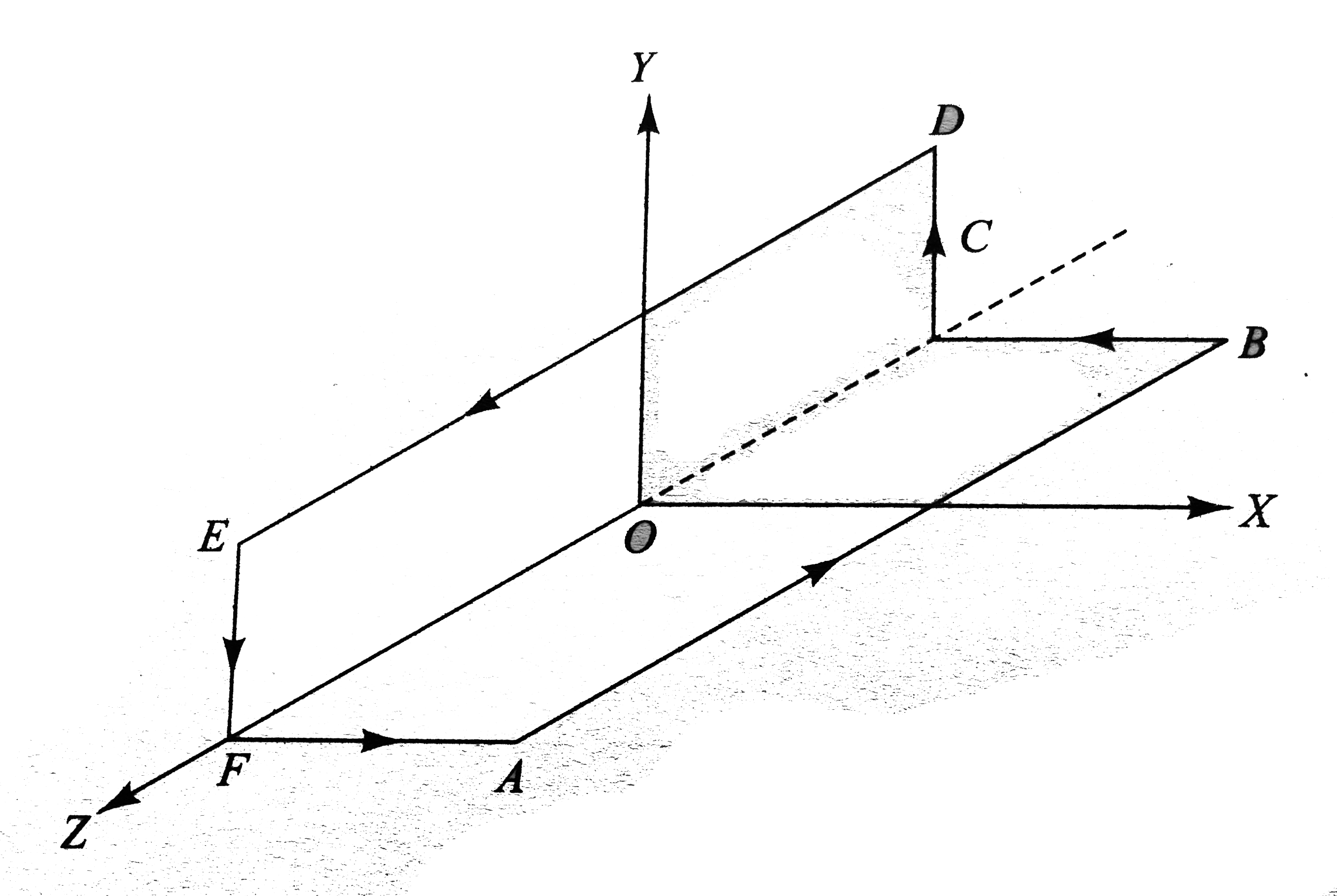
In Fig ABCDEFA was a square loop of side l, but is folded in two equal parts so that half of it lies in the x-z plane and the other half lies in the y-z plane. The origin O is centre of the frame also. The loop carries current 'i'. The magnetic field at the centre is

A

$\frac{μ_{0} i}{2 \sqrt{2} π l} (ˆ i - ˆ j)$

B

$\frac{μ_{0} i}{4 π l} (- ˆ i - ˆ j)$

C

$\frac{\sqrt{2} μ_{0} i}{π l} (ˆ i + ˆ j)$

D

$\frac{μ_{0} i}{\sqrt{2} π l} (ˆ i + ˆ j)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

(c) Due to FABC, the magnetic field at O is alpng y-axis and
due to CDEF, the magnetic field is along x-axis.

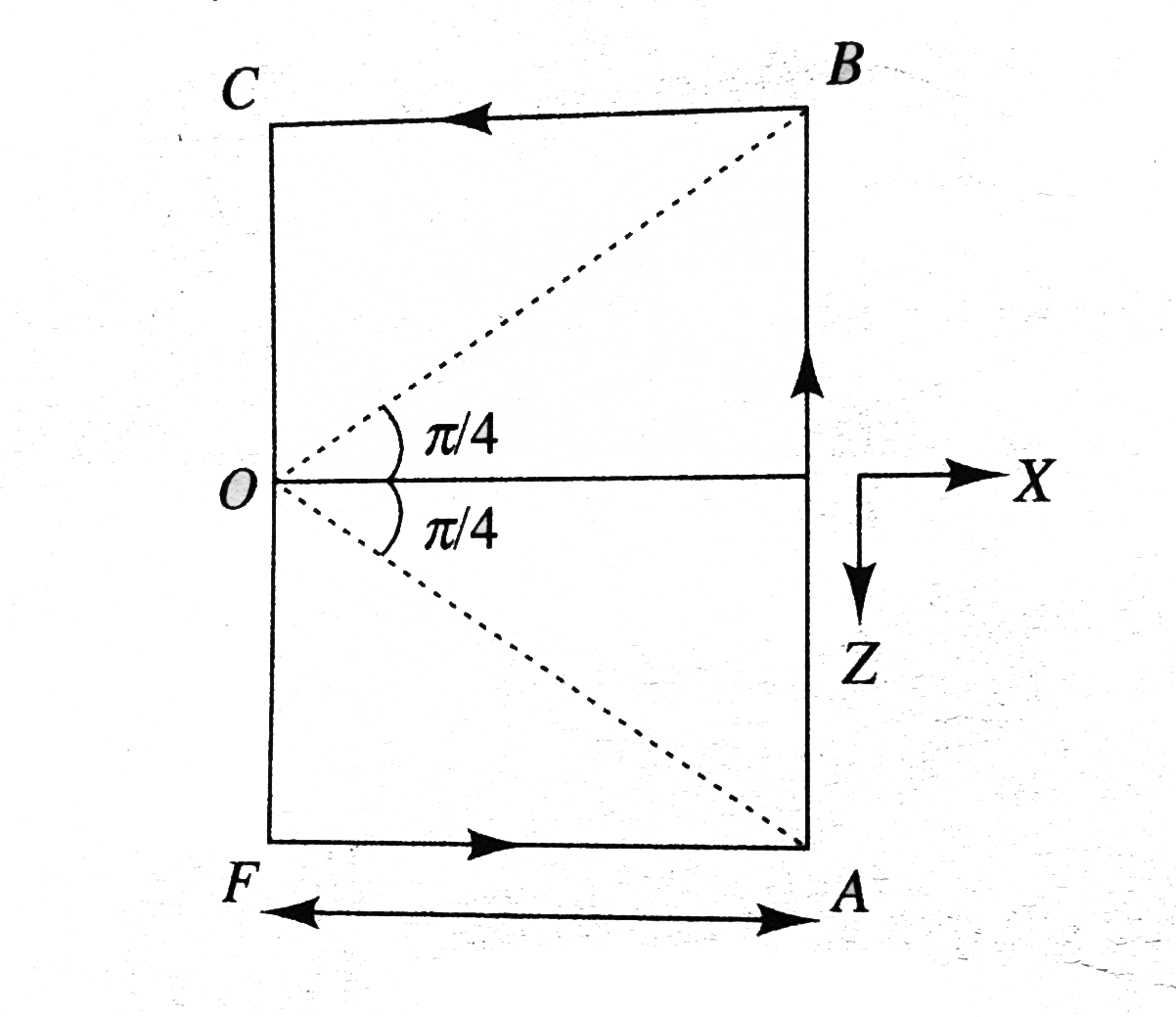
Hence, the field will be of the form $A [ˆ i + ˆ j]$
Calculating field due to FABC:
Due to AB:
${\to B}_{A B} = \frac{μ_{0} i}{4 π (\frac{l}{2})} ({sin 45}^{\circ} + {sin 45}^{\circ}) ˆ j = \sqrt{2} \frac{μ_{0} i}{2 π l} ˆ j$
Due to BC:
${\to B}_{A B} = \frac{μ_{0} i}{4 π (\frac{l}{2})} ({sin 0}^{\circ} + {sin 45}^{\circ}) ˆ j = \frac{μ_{0} i}{2 \sqrt{2} π l} ˆ j$
${\to B}_{F A} = \frac{μ_{0} i}{2 \sqrt{2} π l} ˆ j$
Hence, ${\to B}_{F A B C} = \frac{μ_{0} i}{π l} [\frac{1}{2 \sqrt{2}} + \frac{1}{2 \sqrt{2}} + \frac{\sqrt{2}}{2}] ˆ i$
$\Rightarrow {\to E}_{F A B C} = \frac{\sqrt{2} μ_{0} i}{π l} (ˆ j)$
Similarly due to CDEF: ${\to B}_{C D E F} = \frac{\sqrt{2} μ_{0} i}{π l} ˆ i$
$\Rightarrow {\to B}_{net} = \frac{\sqrt{2} μ_{0} i}{π l} (ˆ i + ˆ j)$

Updated on:21/07/2023

|

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-SOURCES OF MAGNETIC FIELD-Exercise (single Correct )

Home
Ask Doubt
Profile