Consider the RL circuit in Fig. When the switch is closed in position 1 and opens in position 2, electrical work must be performed on the inductor and on the resistor. The energy stored in the inductor is for the resistor energy appears as heat.
a. What is the ratio of PL/PR of the rate at which energy is stored in the inductor to the rate at which energy is dissipated in the resistor?
b. Express the ratio PL/PR as a function of time.
c. If the time constant of circuit is t, what is the time at which PL=PR?
To solve the given problem step by step, we will analyze the RL circuit and derive the required ratios and expressions.
Step 1: Power in the Resistor (P_R)
The power dissipated in the resistor can be expressed using Ohm's law:
PR=I2R
where I is the current flowing through the resistor and R is the resistance.
Step 2: Power in the Inductor (P_L)
The power associated with the inductor can be expressed in terms of the energy stored in the inductor. The energy U stored in the inductor is given by:
U=12LI2
To find the power PL, we differentiate the energy with respect to time:
PL=dUdt=ddt(12LI2)=LIdIdt
Step 3: Ratio of Powers (P_L / P_R)
Now, we can find the ratio of the power in the inductor to the power in the resistor:
PLPR=LIdIdtI2R
Simplifying this expression gives:
PLPR=LR⋅1I⋅dIdt
Step 4: Expressing the Ratio as a Function of Time
In an RL circuit, the current I as a function of time t can be expressed as:
I(t)=ER(1−e−tτ)
where τ=LR is the time constant of the circuit.
To find dIdt:
dIdt=ER⋅1τe−tτ
Substituting I(t) and dIdt into the ratio:
PLPR=LR⋅1ER(1−e−tτ)⋅(ER⋅1τe−tτ)
After simplification, we get:
PLPR=LτRe−tτ⋅RE
This simplifies to:
PLPR=LEτe−tτ
Step 5: Finding Time When P_L = P_R
To find the time t when PL=PR, we set the ratio equal to 1:
PLPR=1
From our previous expression:
LEτe−tτ=1
This implies:
e−tτ=EτL
Taking the natural logarithm of both sides:
−tτ=ln(EτL)
Thus:
t=−τln(EτL)
Final Answers
a. The ratio PLPR=LR⋅1I⋅dIdt.
b. The ratio PLPR=LEτe−tτ.
c. The time at which PL=PR is given by:
t=−τln(EτL).
The power dissipated in the resistor can be expressed using Ohm's law:
PR=I2R
where I is the current flowing through the resistor and R is the resistance.
The power associated with the inductor can be expressed in terms of the energy stored in the inductor. The energy U stored in the inductor is given by:
U=12LI2
To find the power PL, we differentiate the energy with respect to time:
PL=dUdt=ddt(12LI2)=LIdIdt
Now, we can find the ratio of the power in the inductor to the power in the resistor:
PLPR=LIdIdtI2R
Simplifying this expression gives:
PLPR=LR⋅1I⋅dIdt
In an RL circuit, the current I as a function of time t can be expressed as:
I(t)=ER(1−e−tτ)
where τ=LR is the time constant of the circuit.
dIdt=ER⋅1τe−tτ
PLPR=LR⋅1ER(1−e−tτ)⋅(ER⋅1τe−tτ)
PLPR=LτRe−tτ⋅RE
This simplifies to:
PLPR=LEτe−tτ
To find the time t when PL=PR, we set the ratio equal to 1:
PLPR=1
From our previous expression:
LEτe−tτ=1
This implies:
e−tτ=EτL
Taking the natural logarithm of both sides:
−tτ=ln(EτL)
Thus:
t=−τln(EτL)
a. The ratio PLPR=LR⋅1I⋅dIdt.
t=−τln(EτL).
Topper's Solved these Questions
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Solved Examples
3 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Exercise 4.1
24 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Multiple Correct Answer type
2 Videos
Similar Questions
Knowledge Check
The energy stored in an inductor of self-inductance L henry carrying a current of I ampere is
A12L2IB12LI2CLI2DL2I
In an inductor of inductance L=100mH, a current of I=10A is flowing. The energy stored in the inductor is
View SolutionIn an inductor of inductance L=100mH, a current of I=10A is flowing. The energy stored in the inductor is
View SolutionAn inductor may store energy in
View SolutionIn a decaying L−R circuit, the time after which energy stores in the inductor reduces to one fourth of its initial values is
View SolutionThe energy stored in an inductor of self inductance L carrying current l, is given by
View SolutionDefine self-inductance of a coil. Obtain the expression for the energy stored in an inductor L connected across a source of emf.
View SolutionIn the given at t=0, switch S is closed.
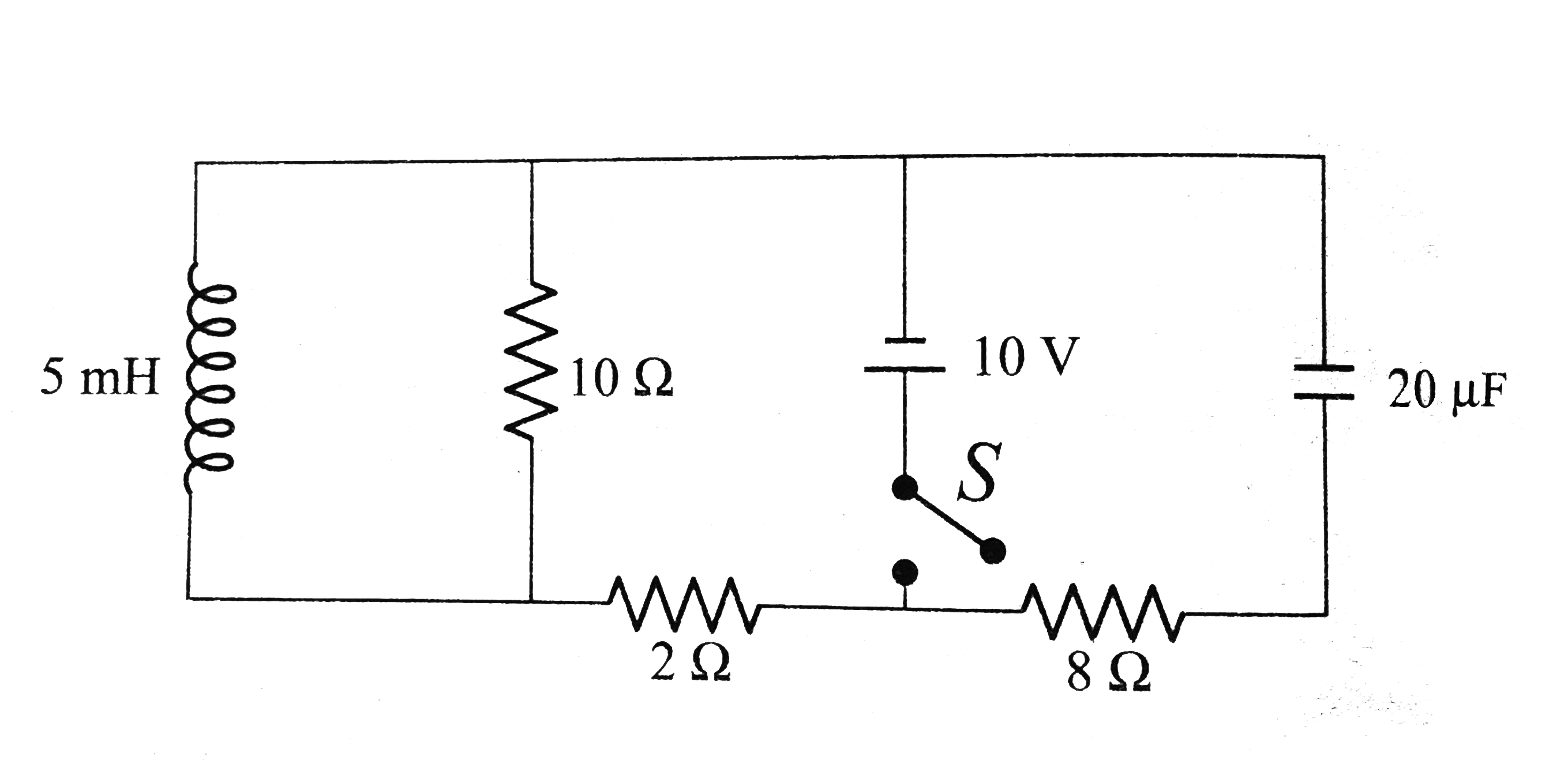
The energy stored in the inductor at any instant t(0<t∞) will be
View Solution