Two coils of self inductance 100 mH and 400 mH are placed very closed to each other. Find maximum mutual inductance between the two when 4 A current passes through them.
200mH
300mH
100√2mH
none of these
The correct Answer is:A
To solve the problem of finding the maximum mutual inductance between two coils with given self-inductances, we can follow these steps:
Step 1: Identify the Self-Inductances
We are given the self-inductances of the two coils:
- L1=100mH=100×10−3H
- L2=400mH=400×10−3H
Step 2: Use the Formula for Maximum Mutual Inductance
The maximum mutual inductance M between two coils can be calculated using the formula:
M=√L1⋅L2
Step 3: Substitute the Values into the Formula
Now, we substitute the values of L1 and L2 into the formula:
M=√100×10−3⋅400×10−3
Step 4: Calculate the Product
Calculating the product inside the square root:
100×400=40000
Thus,
M=√40000×10−6=√0.04=0.2H
Step 5: Convert to Millihenry
Since we need the answer in millihenries, we convert:
0.2H=200mH
Conclusion
The maximum mutual inductance between the two coils is:
M=200mH
Final Answer
Thus, the correct option is 200 mH.
---
We are given the self-inductances of the two coils:
- L1=100mH=100×10−3H
- L2=400mH=400×10−3H
The maximum mutual inductance M between two coils can be calculated using the formula:
M=√L1⋅L2
Now, we substitute the values of L1 and L2 into the formula:
M=√100×10−3⋅400×10−3
Calculating the product inside the square root:
100×400=40000
Thus,
M=√40000×10−6=√0.04=0.2H
Since we need the answer in millihenries, we convert:
0.2H=200mH
The maximum mutual inductance between the two coils is:
M=200mH
Thus, the correct option is 200 mH.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Multiple Correct
34 Videos
- MISCELLANEOUS VOLUME 5BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Linked Comprehension
84 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
Similar Questions
Knowledge Check
Two coil are placed close to each other. The mutual inductance of the pair of coils depends upon.
Amedium between the coilsBdistance between the two coilsCorientation of the two coilsDall of theseTwo coils of self-inductance 2mH and 8mH are placed so close together that the effective flux in one coil is completely linked with the other. The mutual inductance between these coil is
A16 mHB10 mHC6 mHD4 mH
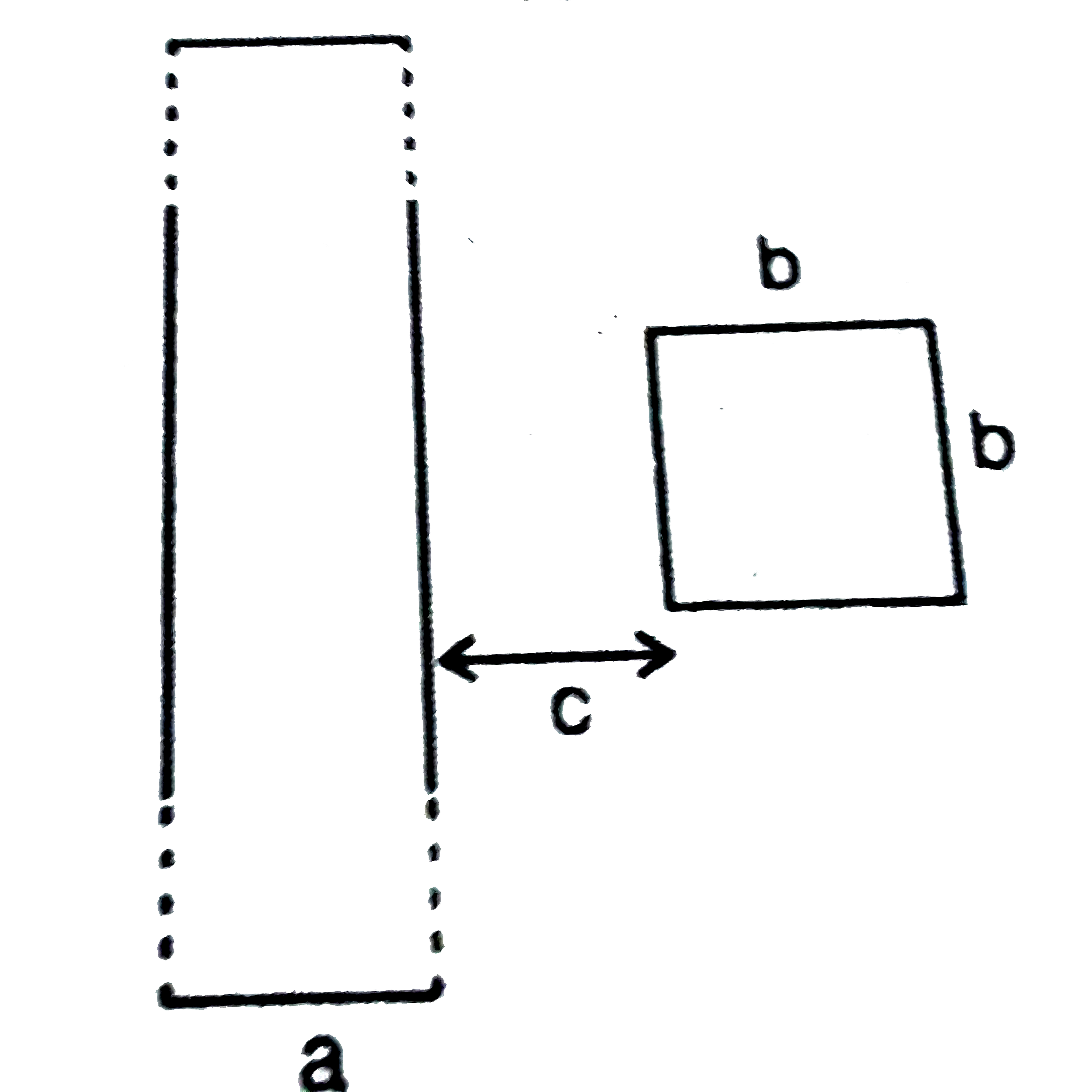
Find the mutual inductance between two rectangular loops, shown in the figure.
View SolutionTwo coils of self-inductance L1 and L2 are placed closed to each other so that total flux in one coil is completely linked with other. If M is mutual inductance between them, then
View SolutionTwo different coils of self inductance L 1 and L 2 are placed close to each other so that the effective flux in one coil is completely linked with other. If M is the mutual inductance between them, then

View SolutionName two factors on which mutual inductance between a pair of coils depends?
View SolutionThe self-inductances of two identical coils are 0.1H. They are wound over each other. Mutual inductance will be-
View SolutionTwo coils of self-inductance 2mH and 8mH are placed so close together that the effective flux in one coil is completely linked with the other. The mutual inductance between these coil is
View Solution
