A magnetic field →B=−B0ˆi exists within a sphere of radius R=v0T√3 where T is the time period of one revolution of a charged particle starting its motion form origin and moving with a velocity vce(v)0=v02√3ˆi−v02ˆj. Find the number of turns that the particle will take to come out of the magnetic field.
The correct Answer is:2
To solve the problem, we need to analyze the motion of a charged particle moving in a magnetic field. The magnetic field is given as →B=−B0^i, and the particle's velocity is given as:
→v=v02√3^i−v02^j
Step 1: Identify the components of velocity
The velocity can be broken down into two components:
- The component in the ^i direction: vx=v02√3
- The component in the ^j direction: vy=−v02
Step 2: Calculate the magnitude of the velocity
The magnitude of the velocity v can be calculated using the Pythagorean theorem:
v=√v2x+v2y=√(v02√3)2+(−v02)2
Calculating this gives:
v=√3v204+v204=√4v204=v0
Step 3: Determine the radius of the circular motion
The radius R of the circular motion in the magnetic field can be expressed as:
R=mvqB
However, we are given that R=v0T√3.
Step 4: Calculate the time period of one revolution
The time period T is the time taken for one complete revolution. The particle moves in a helical path due to the velocity components. The component of velocity perpendicular to the magnetic field contributes to circular motion, while the component parallel to the magnetic field contributes to linear motion.
The perpendicular component of the velocity v⊥ is:
v⊥=vy=−v02
Step 5: Calculate the pitch of the helical path
The pitch P of the helical path is given by the distance traveled in the direction of the magnetic field during one complete revolution. This is calculated as:
P=v∥⋅T
Where v∥ is the component of the velocity parallel to the magnetic field. Here, v∥=vx=v02√3.
Step 6: Calculate the number of turns
The number of turns n the particle makes while it is within the magnetic field can be calculated using the formula:
n=RP
Substituting the values we have:
n=v0T√3v∥⋅T=v0T√3v02√3⋅T
The T and v0 cancel out:
n=√312√3=2
Final Answer
The number of turns that the particle will take to come out of the magnetic field is n=2.
---
The velocity can be broken down into two components:
- The component in the ^i direction: vx=v02√3
- The component in the ^j direction: vy=−v02
The magnitude of the velocity v can be calculated using the Pythagorean theorem:
The radius R of the circular motion in the magnetic field can be expressed as:
The time period T is the time taken for one complete revolution. The particle moves in a helical path due to the velocity components. The component of velocity perpendicular to the magnetic field contributes to circular motion, while the component parallel to the magnetic field contributes to linear motion.
The pitch P of the helical path is given by the distance traveled in the direction of the magnetic field during one complete revolution. This is calculated as:
The number of turns n the particle makes while it is within the magnetic field can be calculated using the formula:
The number of turns that the particle will take to come out of the magnetic field is n=2.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 5Exercise:Linked Comprehension
Explore 84 Videos
- MISCELLANEOUS VOLUME 3Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 3Exercise:True and False
Explore 3 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:ddp.5.5
Explore 14 Videos
Similar Questions
Knowledge Check
A particle of mass m and charge q has and initial velocity →v=υ0ˆj. If an electric field →E=→–––(0)ˆi and magnetic field →B=B0ˆi act on the particle, its speed will double after a time :
At=2mv0qE0Bt=2B0qmv0Ct=√3B0qmv0Dt=√3mv0qE0
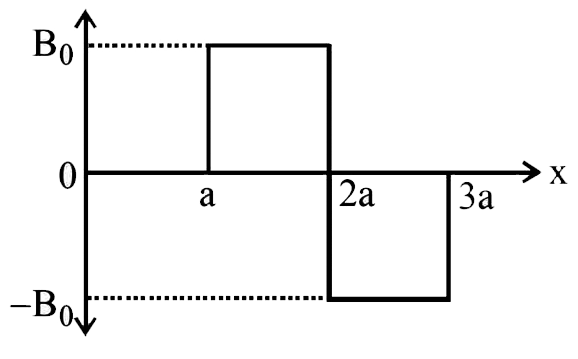
A magnetic field →B=B0ˆj , exists in the region a<x<2a , and →B=−B0ˆj , in the region 2a<x<3a, where B0 is a positive constant . A positive point charge moving with a velocity →v=v0ˆi , where v0 is a positive constant , enters the magnetic field at x=a . The trajectory of the charge in this region can be like
View SolutionA particle of charge q and mass m starts moving from the origin under the action of an electric field →E=E0ˆiand→B=B0ˆi with a velocity →v=v0ˆj. The speed of the particle will become 2v0 after a time.
View SolutionA particle of mass m and charge q has and initial velocity →v=υ0ˆj. If an electric field →E=→–––(0)ˆi and magnetic field →B=B0ˆi act on the particle, its speed will double after a time :
View SolutionThere exists a uniform magnetic field →B=+B0ˆk for x>0and→B=0 for all x<0. A charged particle placed at (0,−a,0) is given an initial velocity →v=v0ˆi. What is the magnitude and nature of charge on the particle such that it crosses through the origin ?
Strategy: When the charge is projected perpendicular to a uniform magnetic field, it follows a circular path. In this case, the force acting on it will be directed either towards +y-axis. or y-axis. It is given that it crosses point O. Thus, the force at (0,−a,0) must be towards y-axis
View SolutionA particle of specific charge α is projected from origin with velocity v=v0ˆi−v0ˆk in a uniform magnetic field B=−B0ˆk. Find time dependence of velocity and position of the particle.
View SolutionAn electric field →E=Bxˆi exists in space, where B=20V/m2. Taking the potential at (2m, 4m) to be zero, find the potential at the origin.
View SolutionIn region x>0 a uniform and constant magnetic field →B1=2B0ˆk exists. Another uniform and constant magnetic field →B2=B0ˆk exists in region x<0 A positively charged particle of mass m and charge q is crossing origin at time t =0 with a velocity ¯u=u0ˆi. The particle comes back to its initial position after a time : (B0,u0 are positive constants)
View Solution
