If velocity of a partical moving along a straight line changes sinusoidally with time as shown in given graph. Find the average speed over time interval t=0 to t=2(2n−1) second, n being any positive interget.
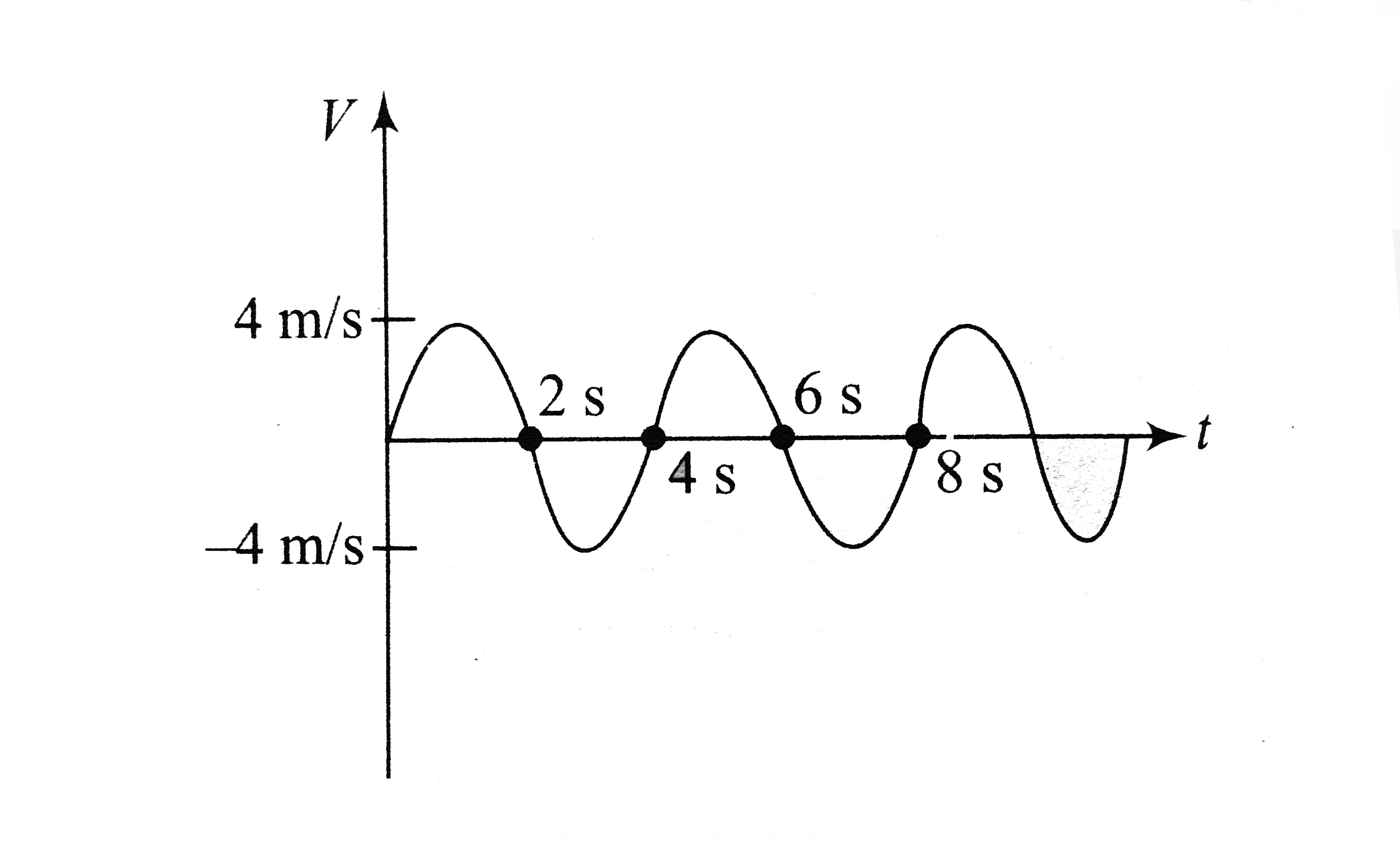
The correct Answer is:8πm/s
Method1 :
Average speed =Total distance travell edTotal time taken=g2(2n−1)
Here Δt=2(2n−1)=4n−=4(n−1)+2
From the graph it is clear that time period Tis4s
∴ΔT=(n−1)T+T2
Total distance travelled in one time period =4A where A is amplitude.
Therefore, total distance travelled in Δt is
S=(n−1)4A+2A=(2n−1)2A
∴>v<=(2n−1)2A2(2n−1)=A and ωA=vmax=4
⇒2π4A=4
⇒A=8π∴>v<8πm/s
Method 2
It can be observed from the graphthat average speed in time interval t=0 to t=2s is same as that in intervals t=0 to t=4s t=0, to t=8s, t=0 to t=12s
or t=0 to t=2(2n−1)s
The speed as function of time is
v=∣∣∣4sin(2π)Tt∣∣∣=∣∣∣4sin(2π)4t∣∣∣=∣4sin(πt)2
v=∣∣∣4sin(2πT)t∣∣∣=∣∣∣4sin(2π4)t∣∣∣=∣∣∣4sin(πt2)∣∣∣
The average speed on time interval t=0 to t=2s is
¯v=∫20vdt∫20dt=(∫204sin πt2dt)2=8πms
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct107videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct35videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.223videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger11videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type3videos
MISCELLANEOUS KINEMATICS
Similar Questions
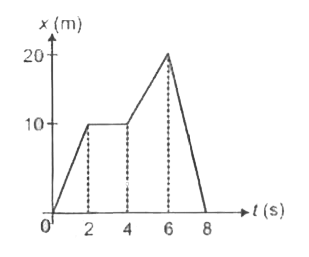
The position (x) - time (t) graph for a particle moving along a straight line is shown in figure. The average speed of particle in time interval t = 0 to t = 8 s is
 View Solution
View SolutionThe position (x) of a particle moving along x - axis veries with time (t) as shown in figure. The average acceleration of particle in time interval t = 0 to t = 8 s is
 View Solution
View SolutionPosition time graph is given find average speed in time t=0 and t=15sec is
 View Solution
View SolutionThe x-t graph of a particle moving along a straight line is shown in figure
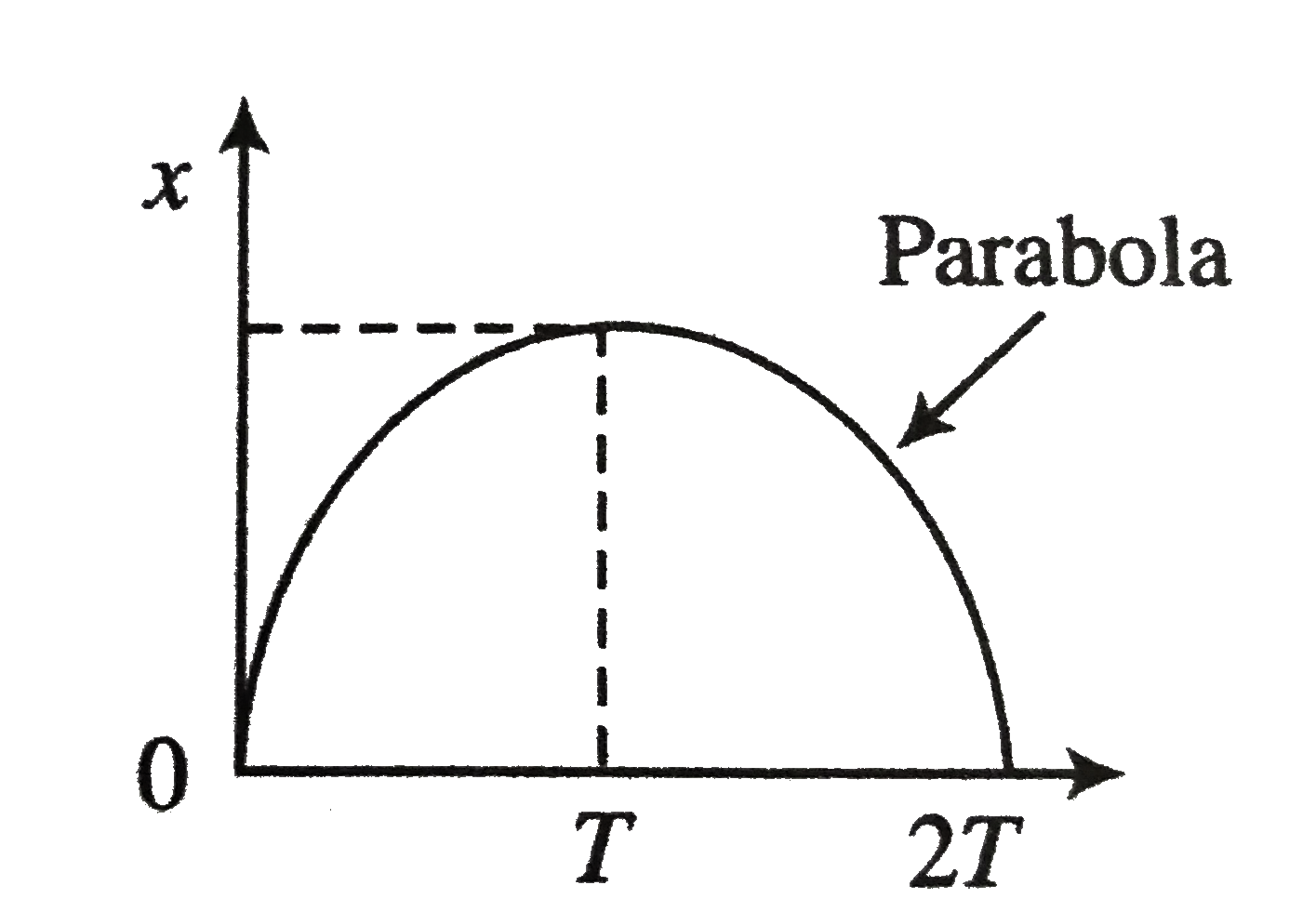
The speed-time graph of the particle is correctly shown byView SolutionThe velocity - time graph of a particle moving along a straight line is shown in the figure given below
The displacement of the particle in 5 second is
 View Solution
View SolutionA body moves in a straight line along Y-axis. Its distance y in metre) from the origin is given y=8t−3t2. The average speed in the time interval from t=0 second to t=1 second is
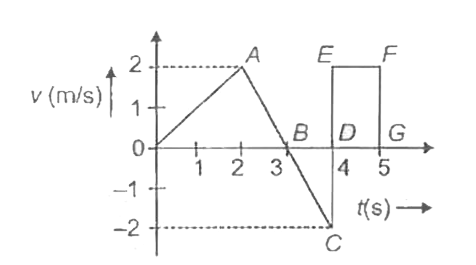
View SolutionThe velocity time graph of a particle moving along a straight line is shown in the figure. In the time interval from t=0 to t=8 second, answer the following three question.

The distance travelled by the particle is :View SolutionThe acceleration of a particle moving along a straight line at any time t is given by a = 4 - 2v, where v is the speed of particle at any time t The maximum velocity is
View SolutionA particle moves rectilinearly possessing a parabolic s-t graph. Find the average velocity of the particle over a time interval from t=12s→t=1.5s.
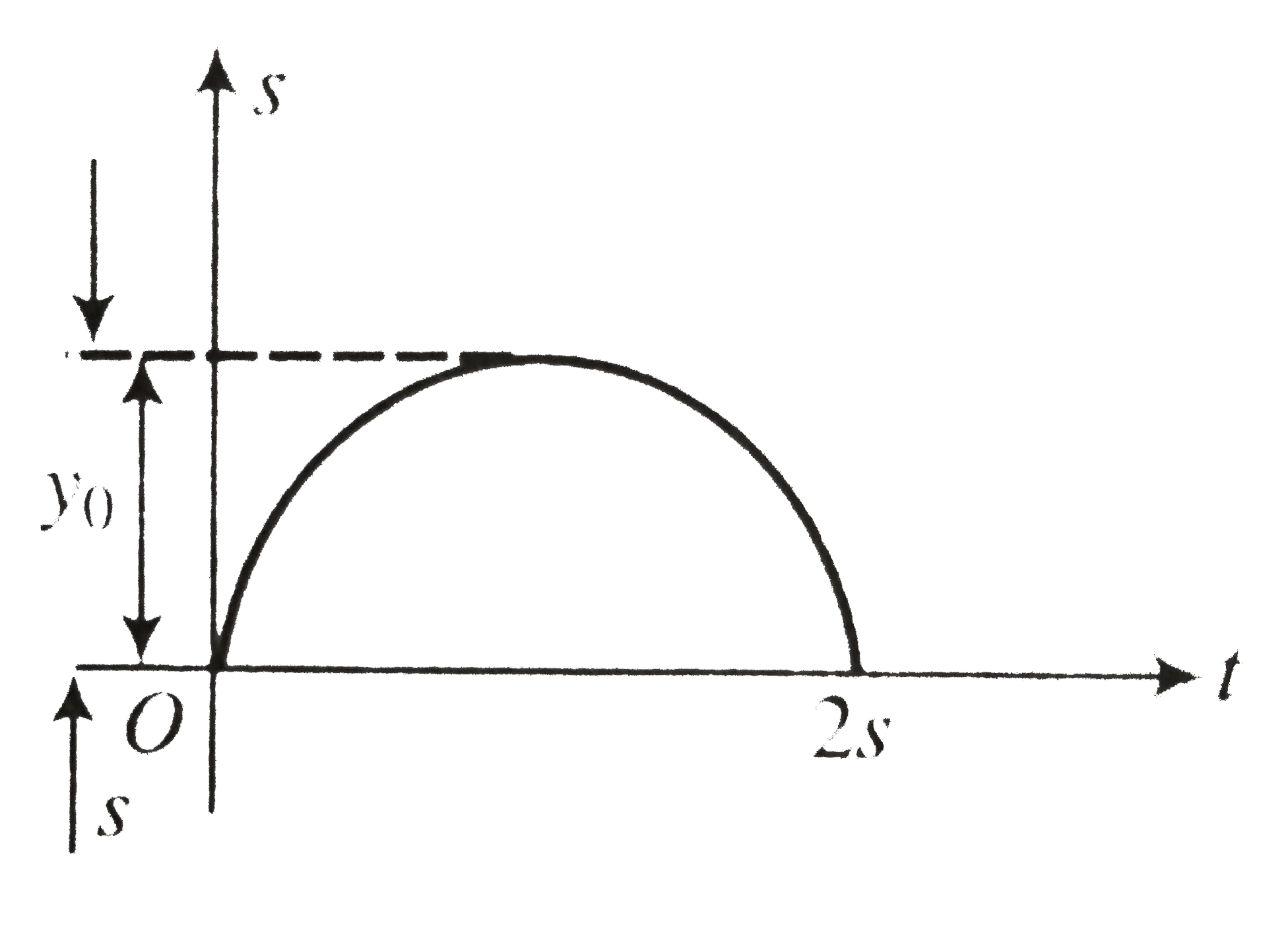 View Solution
View SolutionThe acceleration versus time graph of a particle moving along a straight line is shown in the figure. Draw the respective velocity-time graph Given v=0 at t=0.
 View Solution
View Solution

