Particle A makes a perfectly elastic collision with anther particle B at rest. They fly apart in opposite direction with equal speeds. If the masses are mA&mB respectively, then
2mA=mB
3mA=mB
4mA=mB
√3mA=mB
The correct Answer is:B
To solve the problem of a perfectly elastic collision between particle A and particle B, we will follow these steps:
Step 1: Understand the scenario
- Particle A is moving with an initial speed u and has a mass mA.
- Particle B is at rest and has a mass mB.
- After the collision, both particles move in opposite directions with equal speeds v.
Step 2: Apply conservation of momentum
The law of conservation of momentum states that the total momentum before the collision equals the total momentum after the collision.
Before collision:
- Momentum of A = mA⋅u
- Momentum of B = mB⋅0=0
Total initial momentum = mA⋅u
After collision:
- Momentum of A = −mA⋅v (negative because it moves in the opposite direction)
- Momentum of B = mB⋅v
Total final momentum = −mA⋅v+mB⋅v
Setting the initial momentum equal to the final momentum gives us:
mA⋅u=mB⋅v−mA⋅v
This simplifies to:
mA⋅u=(mB−mA)⋅v(Equation 1)
Step 3: Apply the coefficient of restitution
For a perfectly elastic collision, the coefficient of restitution E is defined as:
E=Velocity of separationVelocity of approach
Since both particles move apart with equal speeds after the collision, the velocity of separation is v+v=2v and the velocity of approach is u.
Setting the coefficient of restitution equal to 1 gives us:
1=2vu
From this, we can express u in terms of v:
u=2v(Equation 2)
Step 4: Substitute Equation 2 into Equation 1
Now we substitute u=2v into Equation 1:
mA⋅(2v)=(mB−mA)⋅v
This simplifies to:
2mA⋅v=mB⋅v−mA⋅v
Dividing through by v (assuming v≠0):
2mA=mB−mA
Rearranging gives:
2mA+mA=mB
Thus:
3mA=mB
Final Result
The relationship between the masses is:
mB=3mA
- Particle A is moving with an initial speed u and has a mass mA.
- Particle B is at rest and has a mass mB.
- After the collision, both particles move in opposite directions with equal speeds v.
The law of conservation of momentum states that the total momentum before the collision equals the total momentum after the collision.
- Momentum of A = mA⋅u
- Momentum of B = mB⋅0=0
- Momentum of A = −mA⋅v (negative because it moves in the opposite direction)
- Momentum of B = mB⋅v
mA⋅u=mB⋅v−mA⋅v
This simplifies to:
mA⋅u=(mB−mA)⋅v(Equation 1)
For a perfectly elastic collision, the coefficient of restitution E is defined as:
E=Velocity of separationVelocity of approach
Since both particles move apart with equal speeds after the collision, the velocity of separation is v+v=2v and the velocity of approach is u.
1=2vu
From this, we can express u in terms of v:
u=2v(Equation 2)
Now we substitute u=2v into Equation 1:
mA⋅(2v)=(mB−mA)⋅v
This simplifies to:
2mA⋅v=mB⋅v−mA⋅v
Dividing through by v (assuming v≠0):
2mA=mB−mA
Rearranging gives:
2mA+mA=mB
Thus:
3mA=mB
The relationship between the masses is:
mB=3mA
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
45 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
16 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - TRY YOURSELF
95 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Particle A makes a head on elastic collision with another stationary particle B. They fly apart in opposite directions with equal speeds. The mass ratio will be
View SolutionParticle 1 experiences a perfectly elastic collision with a stationary particle 2. Determine their mass ratio, if
(a) after a head-on collision the particles fly apart in the opposite directions with equal velocities,
(b) the particles fly apart symmetrically relative to the initial motion direction of particle I with the angle of divergence θ=60∘.
View SolutionTwo particles A and B have the same mass m. A is moving along X-axis with a speed of 5ms−1 and B is at rest. After undergoing a perfectly elastic collision with B, particle A gets scattered through an angle of 60∘. What is the direction of B, and the speeds of A and B, after the collision ?
View SolutionA moving particle of mass m makes a head-on perfectly inelastic collision with a particle of mas 2m which is initially at rest. Find the fractional loss in energy of the colliding partic le after collision.
View SolutionA particle moving with kinetic energy E makes a head on elastic collision with an identical particle at rest. During the collision
View SolutionStatement I: If a sphere of mass mmoving with speed u undergoes a perfectly elastic head-on collision with another sphere of heavier mass M at rest (M>m), then direction of velocity of sphere of mass m is reversed due to collision (no external force acts on system of two spheres).
Statement II: During a collision of spheres of unequal masses, the heavier mass exerts more force on the lighter mass in comparison to the force which lighter mass exerts on the heavier one,
View SolutionA particle A suffers an oblique elastic collision particle B that is at rest initially. If their masses with a are the same, then after the collision
View SolutionA particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
View SolutionA particle of mass m1, makes a head on elastic collision with another stationary body of mass m2 If m1, rebounds with half of its original speed then (m1m2) is
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
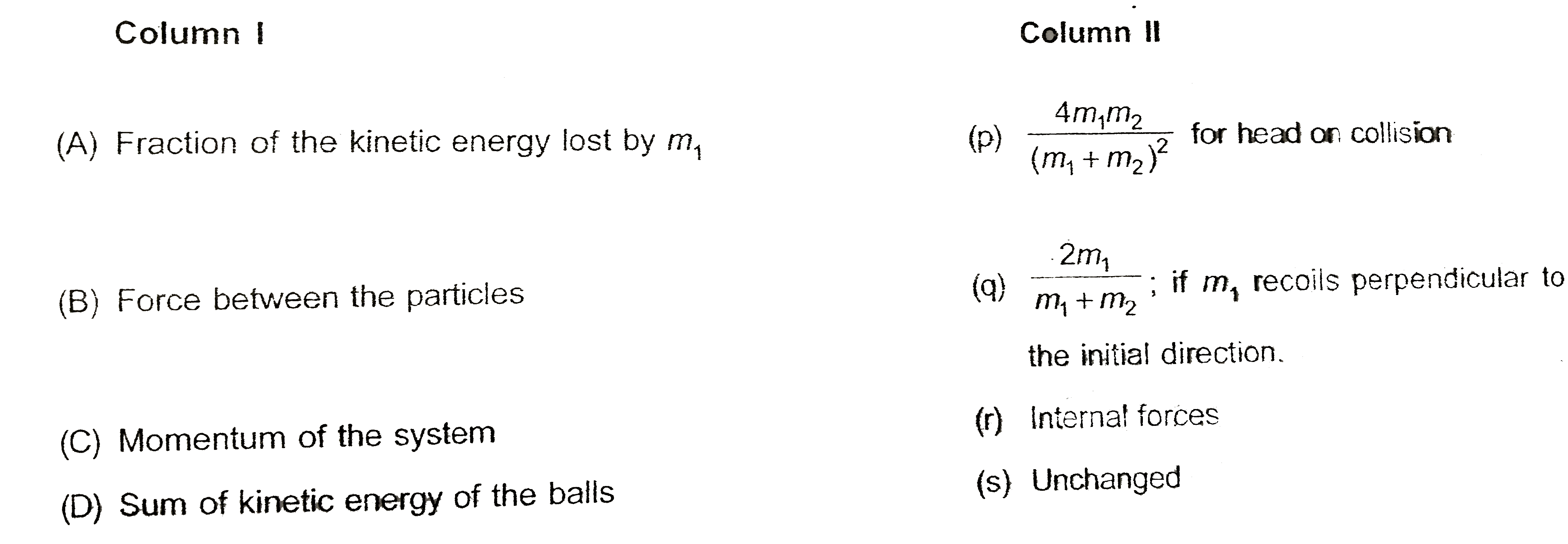
View Solution