A bullet of mass m hits a block of mass M. The transfer of energy is maximum when
m>>M
M>>m
M=2m
M=m
The correct Answer is:D
To solve the problem of determining when the transfer of energy is maximum when a bullet of mass m hits a block of mass M, we can follow these steps:
Step 1: Understand the System
We have a bullet of mass m moving with an initial velocity u that collides with a block of mass M which is initially at rest. After the collision, the bullet embeds itself into the block.
Step 2: Conservation of Momentum
Using the principle of conservation of momentum, we can express the momentum before and after the collision. The initial momentum pi is given by:
pi=mu+0=mu
The final momentum pf after the collision, when the bullet and block move together with a velocity v, is:
pf=(M+m)v
Setting these equal gives us:
mu=(M+m)v
From this, we can solve for v:
v=muM+m
Step 3: Calculate the Change in Kinetic Energy
The change in kinetic energy (ΔKE) can be calculated using the formula:
ΔKE=KEfinal−KEinitial
The initial kinetic energy KEinitial is:
KEinitial=12mu2
The final kinetic energy KEfinal after the collision is:
KEfinal=12(M+m)v2
Substituting v from Step 2 into the final kinetic energy:
KEfinal=12(M+m)(muM+m)2
Simplifying this gives:
KEfinal=12(M+m)m2u2(M+m)2=12m2u2M+m
Step 4: Find the Change in Kinetic Energy
Now, substituting back into the change in kinetic energy:
ΔKE=12m2u2M+m−12mu2
Factoring out 12u2:
ΔKE=12u2(m2M+m−m)
This simplifies to:
ΔKE=12u2(m2−m(M+m)M+m)=12u2(m2−mM−m2M+m)=12u2(−mMM+m)
Step 5: Determine Maximum Energy Transfer
The transfer of energy is maximum when the expression for ΔKE is maximized. This occurs when the ratio of the masses mM is equal to 1, meaning M=m.
Conclusion
Thus, the transfer of energy is maximum when the mass of the block M is equal to the mass of the bullet m.
Final Answer
The transfer of energy is maximum when M=m.
---
We have a bullet of mass m moving with an initial velocity u that collides with a block of mass M which is initially at rest. After the collision, the bullet embeds itself into the block.
Using the principle of conservation of momentum, we can express the momentum before and after the collision. The initial momentum pi is given by:
pi=mu+0=mu
The final momentum pf after the collision, when the bullet and block move together with a velocity v, is:
pf=(M+m)v
Setting these equal gives us:
mu=(M+m)v
From this, we can solve for v:
v=muM+m
The change in kinetic energy (ΔKE) can be calculated using the formula:
ΔKE=KEfinal−KEinitial
The initial kinetic energy KEinitial is:
KEinitial=12mu2
The final kinetic energy KEfinal after the collision is:
KEfinal=12(M+m)v2
Substituting v from Step 2 into the final kinetic energy:
KEfinal=12(M+m)(muM+m)2
Simplifying this gives:
KEfinal=12(M+m)m2u2(M+m)2=12m2u2M+m
Now, substituting back into the change in kinetic energy:
ΔKE=12m2u2M+m−12mu2
Factoring out 12u2:
ΔKE=12u2(m2M+m−m)
This simplifies to:
ΔKE=12u2(m2−m(M+m)M+m)=12u2(m2−mM−m2M+m)=12u2(−mMM+m)
The transfer of energy is maximum when the expression for ΔKE is maximized. This occurs when the ratio of the masses mM is equal to 1, meaning M=m.
Thus, the transfer of energy is maximum when the mass of the block M is equal to the mass of the bullet m.
The transfer of energy is maximum when M=m.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
45 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
16 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - TRY YOURSELF
95 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A bullet of mass m strikes a block of mass M. The bullet remains embedded in the block. Find the amplitude of the resulting SHM.

View SolutionA bullet of mass m is fired into a block of wood of mass M which hangs on the end of pendulum and gets embedded into it. When the bullet strikes with maximum rise R. Then, the velocity of the bullet is given by
View SolutionIn a free space a rifle on mass M shoots a bullet of mass m at a stationary block of mass M distance D away from it . When the bullet has moved through a distance d towards the block the centre of mass of the bullet − block system is at a distance of :
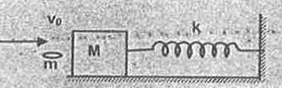
View SolutionA bullet of mass m embeds itself in a block of mass M resting on a smooth horizontal surface, attached to a spring of force constant k. If the initial speed of the bullet is v0 along horizontal, find (a) the maximum compression of the spring and (b) the time for the bullet - block system to come to rest.
View SolutionA bullet of mass m strikes a block of mass M connected to a light spring of stiffness k, with a speed v0 and gets into it. Find the loss of K.E. of the bullet.
View SolutionShow that in a head on elastic collision between two particles, the transference of energy is maximum when their mass ratio is unity.
View SolutionA bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
View SolutionA bullet of mass m hits a target of mass M hanging by a string and gets embedded in it. If the block rises to a height h as a result of this collision, the velocity of the bullet before collision is
View SolutionA block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?

View SolutionA bullet of mass m and velocity v is fired into a large block of mass M. The final velocity of the system is
View Solution
