A small metallic spherical ball is dropped from a height h. If it collides with the ground inelastically and rebounds, then
The momentum of the ball just after the collision is same as that just before the collision
Force on the ball is same (in magnitude) as the force on the ground during the collision
Total momentum of ball and Earth system is conserved
Total kinetic energy of the ball and the earth remains the same
To solve the problem, let's analyze the scenario step by step:
Step 1: Understanding the Situation
A small metallic spherical ball is dropped from a height h. When it hits the ground, it does so inelastically, meaning it does not retain all of its kinetic energy after the collision.
Step 2: Analyze Momentum Before and After Collision
Before the collision, the ball has momentum given by:
pbefore=mv
where m is the mass of the ball and v is its velocity just before impact. The velocity can be calculated using the equation of motion:
v=√2gh
Thus, the momentum before the collision is:
pbefore=m√2gh
After the inelastic collision, the ball rebounds with a lower velocity. The momentum after the collision is:
pafter=mv′
where v′ is the velocity after the collision. Since the collision is inelastic, v′<v.
Step 3: Evaluate the Options
1. Momentum of the ball just after the collision is the same as that just before the collision.
- This is false because the direction changes and the speed decreases after an inelastic collision.
2. Force on the ball is the same in magnitude as the force on the ground during the collision.
- This is true according to Newton's third law of motion (action-reaction pair).
3. Total momentum of the ball and earth system is conserved.
- This is true because the forces acting on the ball and the Earth are equal and opposite, leading to conservation of momentum for the system.
4. Total kinetic energy of the ball and the earth remains the same.
- This is false because in an inelastic collision, some kinetic energy is converted into other forms of energy (like heat or deformation), leading to a loss in total kinetic energy.
Conclusion
From the analysis:
- The second and third statements are true.
- The first and fourth statements are false.
Final Answer
- True statements: 2 and 3
- False statements: 1 and 4
A small metallic spherical ball is dropped from a height h. When it hits the ground, it does so inelastically, meaning it does not retain all of its kinetic energy after the collision.
Before the collision, the ball has momentum given by:
pbefore=mv
where m is the mass of the ball and v is its velocity just before impact. The velocity can be calculated using the equation of motion:
v=√2gh
Thus, the momentum before the collision is:
pbefore=m√2gh
pafter=mv′
where v′ is the velocity after the collision. Since the collision is inelastic, v′<v.
1. Momentum of the ball just after the collision is the same as that just before the collision.
- This is false because the direction changes and the speed decreases after an inelastic collision.
- This is true according to Newton's third law of motion (action-reaction pair).
- This is true because the forces acting on the ball and the Earth are equal and opposite, leading to conservation of momentum for the system.
- This is false because in an inelastic collision, some kinetic energy is converted into other forms of energy (like heat or deformation), leading to a loss in total kinetic energy.
From the analysis:
- The second and third statements are true.
- The first and fourth statements are false.
- True statements: 2 and 3
- False statements: 1 and 4
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - D) Linked Comprehension Type Questions
20 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - E) Assertion-Reason Type Questions
12 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - B) Objective Type Questions (One option is correct)
60 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
A small spherical solid ball is dropped from a great height in a viscous liquid. Its journey in the liquid is best described in the diagram given below by the
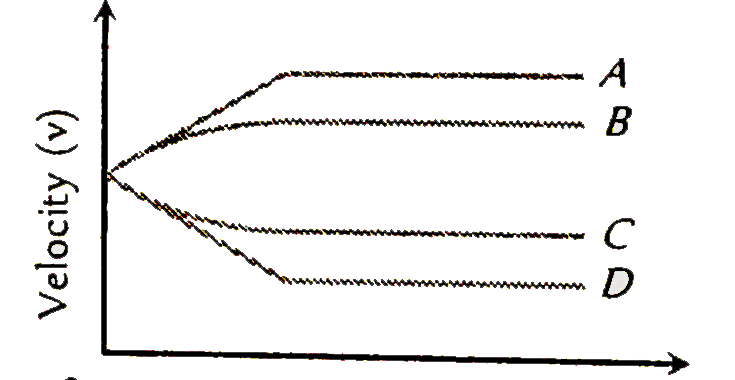
View SolutionA ball is dropped from height 'h' as shown.The collision is perfectly inelastic. Select the correct alternative (s)
View Solution
Knowledge Check
A wooden ball and an iron ball are dropped from the same height h in vaccum. If their radii are the same then the time taken by then to reach the ground are
Aapproximately equalBexactly equalCnot equalDzero
A ball of mass 1 kg is dropped from height 9.8 m, strikes with ground and rebounds at height 4.9 m, if the time of contact between ball and ground is 0.1 s then find impulse and average force acting on ball.
View SolutionA ball of mass m is dropped onto a floor from a certain height. The collision is perfectly elastic and the ball rebounds to the same height and again falls. Find the average force exerted by the ball on the floor during a long time interval.
View SolutionA golf ball is dropped from a height of 80 m. Each time the ball hits the ground, it rebounds to 1/3 of the height through which it has fallen. Then total distance travelled by the ball is 240m b. 160m c. 120m d. 320m
View SolutionA ball is drpped from rest at height 4h. After it has fallen a distance d, a second ball is dropped from rest at height h. What should d be (in terms of h) so that the balls hit the ground at the same time?
View SolutionA ball is thrown vertically downwards from a height of 20m with an intial velocity v0. It collides with the ground, loses 50% of its energy in collision and rebounds to the same height. The intial velocity v0 is (Take, g =10 ms−2)
View SolutionAn inelastic ball is dropped from a height 100 meter. If due to impact it loses 35% of its energy the ball will rise to a height of
View SolutionA particle of mass m is dropped from a height h above the ground. Simultaneously another particle of the same mass is thrown vertically upwards from the ground with a speed of√2gh. If they collide head-on completely inelastically, then the time taken for the combined mass to reach the ground is
View Solution
