Two projectiles collide in air, then for just after and before the collision
The momentum of the system is conserved in all directions
The momentum of the system is conserved only in horizontal direction as gravity is acting in vertical direction
The momentum of individual particles will not be conserved in all directions
The path of centre of mass after collision will depend on coefficient of restitution of collision
To analyze the situation of two projectiles colliding in the air, we will look at the conservation of momentum before and after the collision, as well as the effects of external forces. Here’s a step-by-step solution:
Step 1: Understand the System
We have two projectiles that collide in the air. We need to analyze the momentum of the system before and after the collision.
Hint: Remember that momentum is a vector quantity, which means it has both magnitude and direction.
Step 2: Identify Forces Acting on the System
Before and during the collision, the only external force acting on the system is gravity, which acts downward. This means that while the projectiles are in motion, they are subject to gravitational force.
Hint: Consider how external forces affect the conservation of momentum in different directions.
Step 3: Analyze Momentum Conservation
- Horizontal Direction: In the horizontal direction, there are no external forces acting on the system. Therefore, the momentum in the horizontal direction is conserved.
- Vertical Direction: In the vertical direction, the gravitational force acts on both projectiles. This means that the momentum in the vertical direction is not conserved during the collision due to the presence of this external force.
Hint: Think about how the presence of external forces can disrupt momentum conservation in certain directions.
Step 4: Evaluate Individual Particle Momentum
The momentum of each individual projectile will not be conserved in all directions due to the forces acting on them (specifically, gravity). Each projectile experiences a change in momentum because of the collision and the gravitational force.
Hint: Remember that the momentum of a system can be conserved even if the momentum of individual particles is not.
Step 5: Center of Mass Motion
The center of mass of the system will continue to move as it was moving before the collision, assuming no external horizontal forces are acting on it. The path of the center of mass will not change due to the collision.
Hint: The motion of the center of mass is determined by the net external forces acting on the system.
Conclusion
1. The momentum of the system is conserved in the horizontal direction but not in the vertical direction due to gravity.
2. The momentum of individual particles is not conserved in all directions.
3. The path of the center of mass remains unchanged in the absence of external forces acting on it.
Summary of Statements:
- Statement 1: False - Momentum is not conserved in all directions.
- Statement 2: True - Momentum is conserved only in the horizontal direction.
- Statement 3: True - Individual particle momentum is not conserved in all directions.
- Statement 4: False - The path of the center of mass does not depend on the collision.
We have two projectiles that collide in the air. We need to analyze the momentum of the system before and after the collision.
Before and during the collision, the only external force acting on the system is gravity, which acts downward. This means that while the projectiles are in motion, they are subject to gravitational force.
- Horizontal Direction: In the horizontal direction, there are no external forces acting on the system. Therefore, the momentum in the horizontal direction is conserved.
- Vertical Direction: In the vertical direction, the gravitational force acts on both projectiles. This means that the momentum in the vertical direction is not conserved during the collision due to the presence of this external force.
The momentum of each individual projectile will not be conserved in all directions due to the forces acting on them (specifically, gravity). Each projectile experiences a change in momentum because of the collision and the gravitational force.
The center of mass of the system will continue to move as it was moving before the collision, assuming no external horizontal forces are acting on it. The path of the center of mass will not change due to the collision.
1. The momentum of the system is conserved in the horizontal direction but not in the vertical direction due to gravity.
2. The momentum of individual particles is not conserved in all directions.
3. The path of the center of mass remains unchanged in the absence of external forces acting on it.
- Statement 1: False - Momentum is not conserved in all directions.
- Statement 2: True - Momentum is conserved only in the horizontal direction.
- Statement 3: True - Individual particle momentum is not conserved in all directions.
- Statement 4: False - The path of the center of mass does not depend on the collision.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - D) Linked Comprehension Type Questions
20 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - E) Assertion-Reason Type Questions
12 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - B) Objective Type Questions (One option is correct)
60 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
STATEMENT-l : In an elastic collision between two bodies, the relative speed of the bodies after collision is equal to the relative speed before the collision.
STATEMENT-2 : In an elastic collision, the linear momentum of the system is conserved.
View SolutionStatement I: In an elastic collision between two bodies, the relative speed of the bodies after collision is equal to the relative speed before the collision.
Statement II: In an elastic collision, the linear momentum of the system is conserved.
View SolutionTwo particles are seen to collide and move jointly together after the collision . During such a collision, for the total system,
View SolutionA body falling on the ground from a height of 10m, rebounds to a height 2.5 m Calculate
(i) The percentage loss in KE
(ii) Ratio of the velocities of the body just before and just after the collision.
View SolutionA particle of mass m collides with another stationary particle of mass M such that the second particle starts moving and the first particle stops just after the collision. Then which of the following conditions must always be valid ?
View SolutionTwo balls with masses m1=3 and m2=5kg have initial velocities v1=v2=5m/s in the directions shown in figure. They collide at the origin.
a. find the velocioty of the CM 3s before the collision.
b. Find the position of the CM 2s after the collision.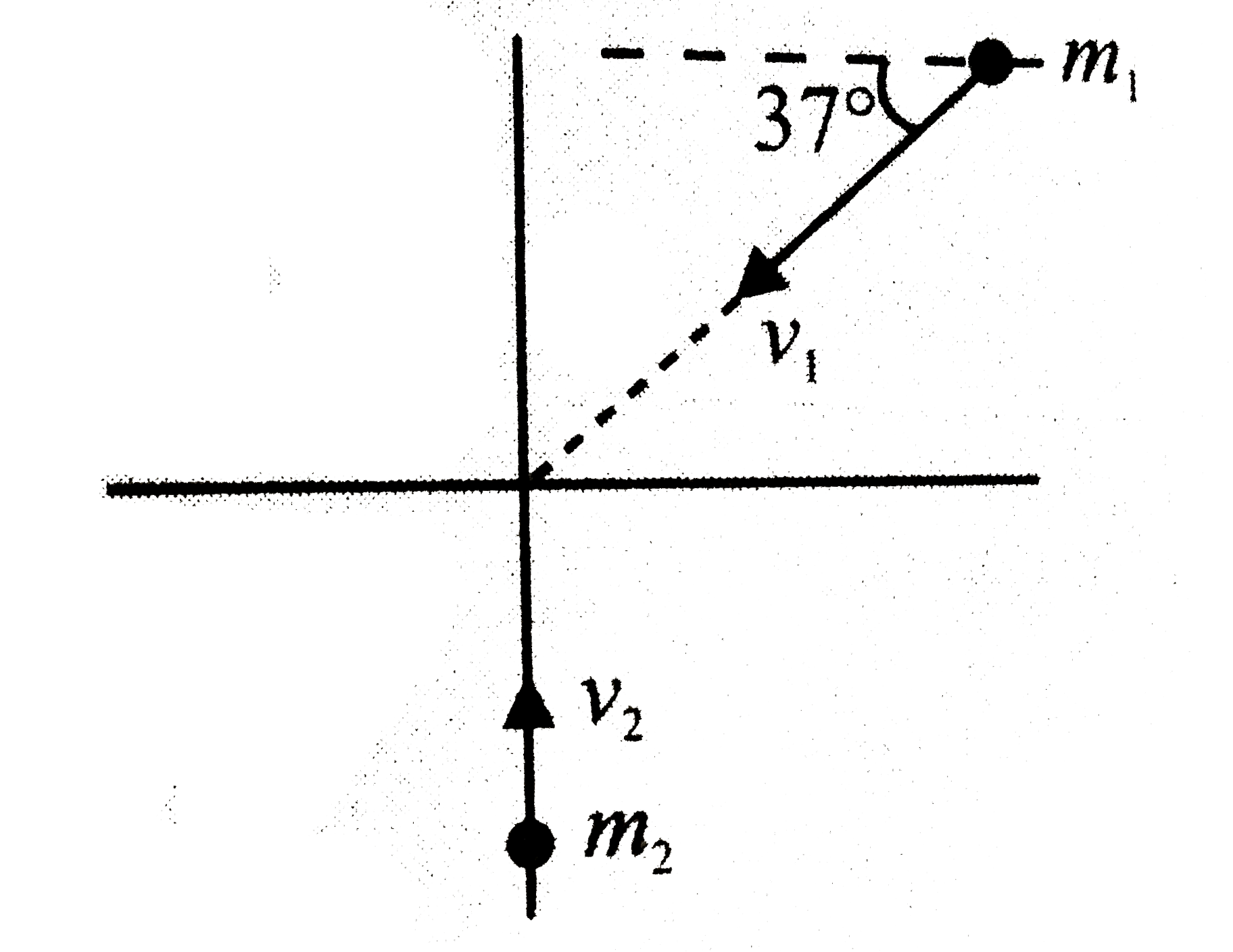
View SolutionA body falls on the ground from a height of 10 m and rebounds to a height of 2.5 m calculate (i) the percentage loss of kinetic energy of the body during the collision with the ground (ii). The ratio of the velocities of the body just before and just after hte collision.
View SolutionTwo identical buggies of each of mass 150kg move one after the other friction with same velocity 4ms. A man of mass m rides the rear buggy. At a certain moment, the man jumps into the front buggy with a velocity v relative to his buggy. As a result of this process, real boggy stops. If the sum of kinetic energies of the man and the front buggy just after collision with the from buggy differs from that just before collision by 2700J then
the mass m of the man is
View SolutionA glass ball collides with a smooth horizontal surface (xz plane) with a velocity V=ai−bj. If the coefficient of restitution of collision be e, the velocity of the ball just after the collision will be
View SolutionBody A of mass 4m moving with speed u collides with another body B of mass 2m at rest the collision is head on and elastic in nature. After the collision the fraction of energy lost by colliding body A is :
View Solution
