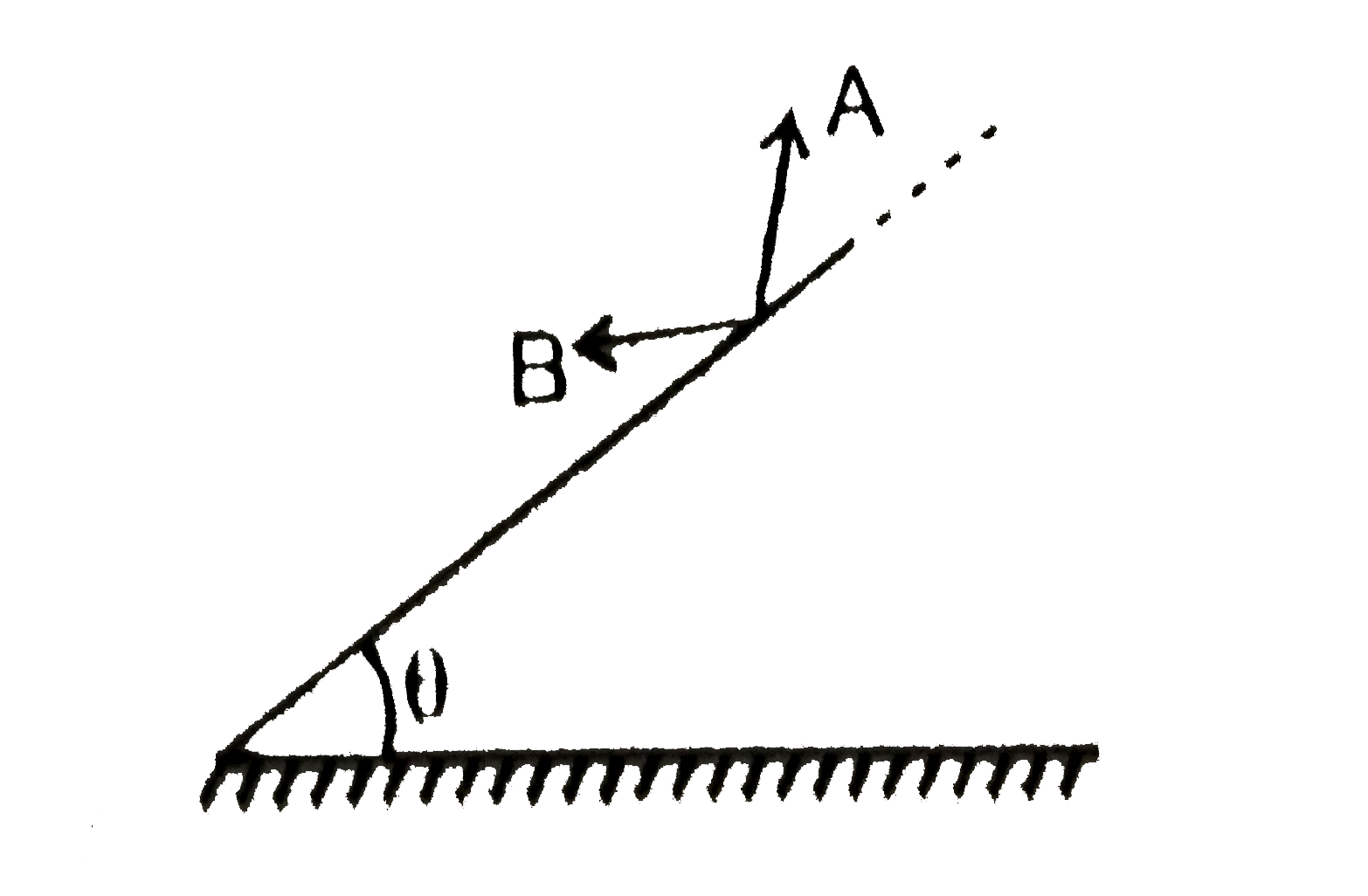
A disc of given some velocity up the incline so that it goes up, stops momentarily and comes down the incline. The disc never slips during its entire motion. The force of friction
Acts downward along the incline when the disc goes up the incline
Acts upward along the incline when the disc goes up the incline
Acts upward along the incline when the disc comes down in the incline
Acts downward along the incline when the disc comes down in the incline
To solve the problem regarding the direction of the force of friction acting on a disc rolling up and down an incline, we can break it down into a step-by-step analysis.
Step 1: Understand the Motion of the Disc
The disc is given an initial velocity up the incline. As it moves up, it will eventually stop and then roll back down. The key point is that the disc rolls without slipping, which means that both translational and rotational motions are involved.
Hint: Consider the forces acting on the disc as it moves up and down the incline.
Step 2: Analyze the Forces When the Disc Moves Up
When the disc moves up the incline, it is experiencing a force of friction. Since the disc is rolling up, it is slowing down. The frictional force must act in the direction opposite to the motion to reduce its speed.
Hint: Think about how friction affects the angular velocity of the disc.
Step 3: Determine the Direction of Friction When Going Up
As the disc moves upward, its angular speed (ω) decreases. The torque produced by the frictional force must act in a direction that opposes the motion. Therefore, the frictional force must act upward along the incline to create a counter-clockwise torque that reduces the angular speed.
Hint: Visualize the torque direction and how it relates to the angular speed.
Step 4: Analyze the Forces When the Disc Comes Down
When the disc reaches the top and starts to roll back down, its motion changes. Now, the disc is accelerating down the incline. The frictional force will again act to oppose the motion.
Hint: Consider how the direction of motion affects the frictional force.
Step 5: Determine the Direction of Friction When Coming Down
As the disc rolls down, it is accelerating and increasing its angular speed. The frictional force must still act in the direction opposite to the motion. Thus, the frictional force will again act upward along the incline to create a counter-clockwise torque that increases the angular speed.
Hint: Remember that friction always acts to oppose the relative motion between surfaces.
Conclusion
In both cases—when the disc is moving up and when it is coming down—the force of friction acts upward along the incline.
Final Answer
The correct statement is:
- Acts upward along the incline when the disc goes up the incline.
- Acts upward along the incline when the disc comes down the incline.
The disc is given an initial velocity up the incline. As it moves up, it will eventually stop and then roll back down. The key point is that the disc rolls without slipping, which means that both translational and rotational motions are involved.
When the disc moves up the incline, it is experiencing a force of friction. Since the disc is rolling up, it is slowing down. The frictional force must act in the direction opposite to the motion to reduce its speed.
As the disc moves upward, its angular speed (ω) decreases. The torque produced by the frictional force must act in a direction that opposes the motion. Therefore, the frictional force must act upward along the incline to create a counter-clockwise torque that reduces the angular speed.
When the disc reaches the top and starts to roll back down, its motion changes. Now, the disc is accelerating down the incline. The frictional force will again act to oppose the motion.
As the disc rolls down, it is accelerating and increasing its angular speed. The frictional force must still act in the direction opposite to the motion. Thus, the frictional force will again act upward along the incline to create a counter-clockwise torque that increases the angular speed.
In both cases—when the disc is moving up and when it is coming down—the force of friction acts upward along the incline.
The correct statement is:
- Acts upward along the incline when the disc goes up the incline.
- Acts upward along the incline when the disc comes down the incline.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:Assignment (Section - D) Linked Comprehension Type Questions
Explore 20 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:Assignment (Section - E) Assertion-Reason Type Questions
Explore 12 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTIONExercise:Assignment (Section - B) Objective Type Questions (One option is correct)
Explore 60 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBook:AAKASH INSTITUTE ENGLISHChapter:SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSExercise:Assignment (Section-D (Assertion and reason))
Explore 5 Videos
- TEST 1Book:AAKASH INSTITUTE ENGLISHChapter:TEST 1Exercise:EXERCISE
Explore 21 Videos
Similar Questions
For a given velocity of projection from a point on the inclined plane, the maximum range down the plane is three times the maximum range up the incline. Then find the angle of inclination of the inclined plane.
View SolutionA block is pushed with some velocity up a rough inclined plane. It stops after ascending few meters and then reverses its direction and returns back to point from where it started. If angle of inclination is 37∘ andthe time to climb up is half of the time to return back then coefficient of friction is:
View SolutionA pebble is thrown up. It goes to a height and then comes back on the ground. State the different changes in form of energy during its motion.
View SolutionThe force required to move a body up a rough inclined plane is double the force required to prevent the body from sliding down the plane. The coefficient of friction when the angle of inclination of the plane is 60∘ is .
View SolutionA small block of mass m is kept on a rough inclined surface of inclination θ fixed in an elevator. The elevator goes up with a uniform velocity v and the block does not slide on the wedge. The work done by the force of friction on the block in time t will be
View SolutionA particle is projected up the inclined such that its component of velocity along the incline is 10m/s. Time of flight is 2 sec and maximum height above the incline is 5m. Then velocity of projection will be
View SolutionThe force required to just move a body up the inclined plane is double the force required to just prevent the body from sliding down the plane. The coefficient of friction is μ. If θ is the angle of inclination of the plane than tanθ is equal to
View SolutionA block moves down a smooth inclined plane of inclination θ. Its velocity on reaching the bottom is v. If it slides down a rough inclined plane of some inclination, its velocity on reaching the bottom is v/n, where n is a number greater than 0. The coefficient of friction is given by -
View SolutionTwo stones A and B are projected from an inclined plane such that A has range up the incline and B has range down the incline. For range of both stones on the incline to be equal in magnitude, pick up the correct condition. ( Neglect air friction 0
View SolutionA small block of mass 1kg is kept on a rough inclined wedge of inclination 45∘ fixed in an elevator. The elevator goes up with a uniform velocity v=2m/s and the block does not slide on the wedge. Find the work done by the force of friction on the block in 1s. (g=10m/s2)
View Solution