A cubical tanker completely filled with water is moving with a constant velocity. A hole develops at the bottom in its horizontal surface through which water comes out. 4kg of water leaks out in 30 seconds. If the velocity of truck becomes x times of its initial velocity then find x.
To solve the problem, we will use the principle of conservation of momentum. Here are the steps to find the value of x:
Step 1: Understand the Given Information
- A cubical tanker filled with water is moving with an initial velocity V.
- Water leaks out of the tanker at a rate of 4kg in 30s.
- After the water leaks out, the velocity of the tanker becomes x times its initial velocity.
Step 2: Define the Initial and Final Momentum
- Let the initial mass of the tanker filled with water be M.
- The initial momentum Pinitial of the tanker is given by:
Pinitial=M⋅V
- After 30s, the mass of the water that has leaked out is 4kg. Therefore, the final mass of the tanker is:
M−4kg
- The final momentum Pfinal of the tanker, after the water has leaked out and its velocity is xV, is given by:
Pfinal=(M−4)⋅(xV)
Step 3: Apply Conservation of Momentum
According to the conservation of momentum:
Pinitial=Pfinal
This gives us the equation:
M⋅V=(M−4)⋅(xV)
Step 4: Simplify the Equation
We can divide both sides of the equation by V (assuming V≠0):
M=(M−4)⋅x
Step 5: Rearrange the Equation to Solve for x
Expanding the right side:
M=Mx−4x
Rearranging gives:
Mx−M=4x
Factoring out M on the left:
M(x−1)=4x
Now, we can solve for x:
x−1=4xM
Multiplying both sides by M:
M(x−1)=4x
Rearranging gives:
Mx−M=4x
Mx−4x=M
Factoring out x:
x(M−4)=M
Thus, we find:
x=MM−4
Step 6: Conclusion
The value of x is given by:
x=MM−4
- A cubical tanker filled with water is moving with an initial velocity V.
- Water leaks out of the tanker at a rate of 4kg in 30s.
- After the water leaks out, the velocity of the tanker becomes x times its initial velocity.
- Let the initial mass of the tanker filled with water be M.
- The initial momentum Pinitial of the tanker is given by:
Pinitial=M⋅V
M−4kg
Pfinal=(M−4)⋅(xV)
According to the conservation of momentum:
Pinitial=Pfinal
This gives us the equation:
M⋅V=(M−4)⋅(xV)
We can divide both sides of the equation by V (assuming V≠0):
M=(M−4)⋅x
Expanding the right side:
M=Mx−4x
Rearranging gives:
Mx−M=4x
Factoring out M on the left:
M(x−1)=4x
Now, we can solve for x:
x−1=4xM
Multiplying both sides by M:
M(x−1)=4x
Rearranging gives:
Mx−M=4x
Mx−4x=M
Factoring out x:
x(M−4)=M
Thus, we find:
x=MM−4
The value of x is given by:
x=MM−4
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - H) Multiple True-False Type Questions
4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - I) Subjective Type Questions
8 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - F) Matrix-Match Type Questions
8 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
What is the angular acceleration of a particle if the angular velocity of a particle becomes 4 times of its initial angular velocity 1 rad s in 2 seconds
View SolutionWhat is the angular acceleration of a particle if the angular velocity of a particle becomes 4 times of its initial angular velocity 1 rad s in 2 seconds
View Solution
Knowledge Check
A tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
Athe volume of water flowing out per second will decreaseBthe velocity of outflow of water remains unchangedCthe volume of water volume out per second remains zeroDBoth (b) and (c)A cylinder of height 20 m is completely filled with water. The velocity of efflux of water through a hole on the side wall of the cylinder near its bottom is (Take g=10ms−2)
A10ms−1B20ms−1C25.5 m s−1D5ms−1
A tube - well pump out 2400 kg of water per minute. If water is coming out with a velocity of 3m/s, the power of the pump is
View SolutionThe acceleration-time (a−t) graph of a particle moving along straight line is as shown in figure. The time at which velocity of particle becomes equal to its initial velocity is

View SolutionA container is filled with water , accelerating with acceleration 10m/s2 , along + ve X - axis on a smooth horizontal surface . The velocity of efflux of water at a point P t the bottom of the tank and near its left most corner is
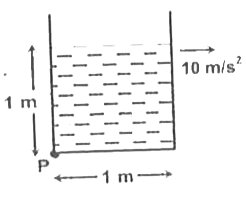
View SolutionA cylinder of height 20m is completely filled with water. The velocity of effux of water (∈ms−1) through a small hole on the side wall of the cylinder near its bottom is
View SolutionAn open cubical tank was initially fully filled with water. When the tank was accelerated on a horizontal plane along one of its side it was found that one thrid of volume of water was spilled out. The acceleration was
View SolutionA horizontal disk is rotating with angular velocity ω about a vertical axis passing through its centre. A ball is placed at the centre of groove and pushed slightly. The velocity of the ball when it comes out of the groove -

View Solution
