A magnetic needle possesses a dipole moment M . It is now bent at the middle to form L shape . What will be the effective dipole moment of the L shape ?
To solve the problem of finding the effective dipole moment of an L-shaped magnetic needle that was originally a straight needle with a dipole moment M, we can follow these steps:
Step 1: Understand the Original Dipole Moment
The original magnetic needle has a dipole moment M, which can be expressed as:
M=m⋅L
where m is the pole strength and L is the length of the needle.
Step 2: Analyze the L-Shaped Configuration
When the needle is bent at the middle to form an L-shape, the total length remains the same, but the effective length of the dipole changes. The L-shape consists of two segments, each of length L2.
Step 3: Calculate the Effective Length of the L-Shape
To find the effective length of the L-shaped configuration, we can use the Pythagorean theorem. The two segments of the L-shape can be treated as the two sides of a right triangle, where the effective length Leffective is the hypotenuse:
Leffective=√(L2)2+(L2)2
Calculating this gives:
Leffective=√L24+L24=√L22=L√2
Step 4: Determine the New Dipole Moment
The pole strength m remains the same since the cross-sectional area of the needle does not change. The new dipole moment M′ of the L-shaped needle can now be calculated as:
M′=m⋅Leffective=m⋅L√2
Substituting m from the original dipole moment equation:
M′=M√2
Final Result
Thus, the effective dipole moment of the L-shaped magnetic needle is:
M′=M√2
---
The original magnetic needle has a dipole moment M, which can be expressed as:
M=m⋅L
where m is the pole strength and L is the length of the needle.
When the needle is bent at the middle to form an L-shape, the total length remains the same, but the effective length of the dipole changes. The L-shape consists of two segments, each of length L2.
To find the effective length of the L-shaped configuration, we can use the Pythagorean theorem. The two segments of the L-shape can be treated as the two sides of a right triangle, where the effective length Leffective is the hypotenuse:
Leffective=√(L2)2+(L2)2
Calculating this gives:
Leffective=√L24+L24=√L22=L√2
The pole strength m remains the same since the cross-sectional area of the needle does not change. The new dipole moment M′ of the L-shaped needle can now be calculated as:
M′=m⋅Leffective=m⋅L√2
Substituting m from the original dipole moment equation:
M′=M√2
Thus, the effective dipole moment of the L-shaped magnetic needle is:
M′=M√2
Topper's Solved these Questions
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - Try Yourself
53 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - Assignment Section - A Objective Type Questions (One option is correct)
58 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - J
9 Videos
Similar Questions
A molecule possessing dipole moment is
View SolutionWith reference to magnetic dipole, match the tems of Column I with the tems of Column Ii and Choose the correct option from the codes given below.

View SolutionA thin bar magnet has dipole moment M . If this is bent in the form of semicircle , then find the new dipole moment .
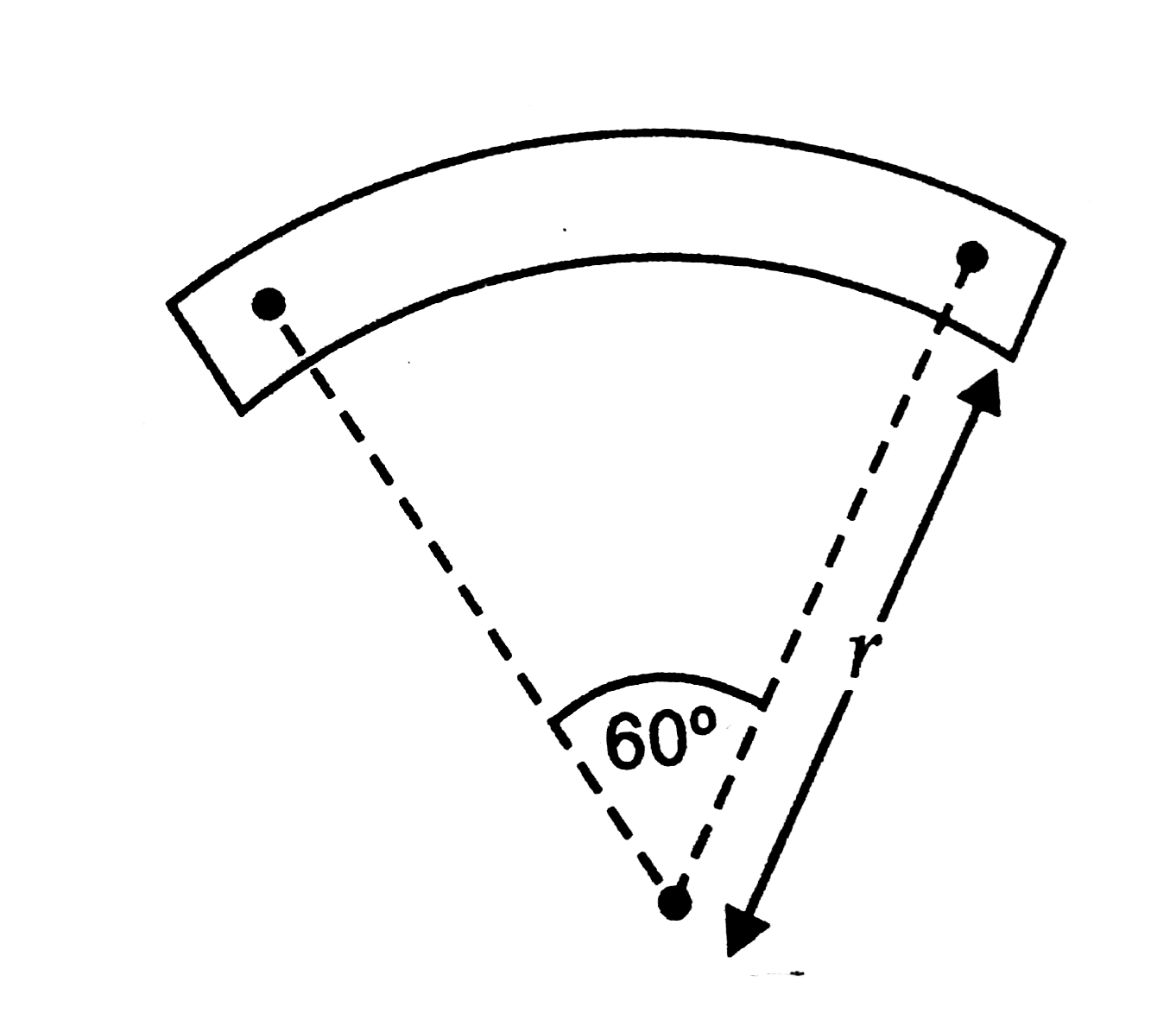
View SolutionA bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
View SolutionState the ȘI unit of magnetic dipole moment.
View SolutionCompute the magnetic dipole moment of the loop shown in Fig. 1.69
View SolutionA thin magnetic needle is bent to form a semi-circle . What will be the percentage change in equivalent dipole moment ?
View SolutionThe magnetic dipole moment of a bar magnet is directly proportional to volume of the bar magnetic . You are given a bar magnet of length I , magnetic pole strength m and magnetic dipole moment M . What will be the effect on dipole moment and pole strength if the bar magnet is cut into the two equal pieces ?
(i) Along it length ?
(ii) Transverse to its length ?
View SolutionWhat are SI units of dipole moment?
View SolutionA magnetic wire of dipole moment 4πAm2 is bent in the form of semicircle. The new magnetic moment is
View Solution
