The kinetic energy of a particle moving along x-axis varies with the distance x of the particle from origin as K=A+x3Bx1/4+C .Write the dimensional formula for A2B
The correct Answer is:[M−1L27/4T2]
To find the dimensional formula for A2B from the given expression for kinetic energy K=A+x3Bx1/4+C, we will follow these steps:
Step 1: Understand the given equation
The equation for kinetic energy is given as:
K=A+x3Bx1/4+C
Where:
- K is kinetic energy.
- A, B, and C are constants.
- x is the distance from the origin.
Step 2: Analyze the dimensions of kinetic energy
The dimensional formula for kinetic energy K is:
(K)=ML2T−2
Step 3: Apply the principle of homogeneity
According to the principle of homogeneity, all terms in the equation must have the same dimensions. Therefore, we can equate the dimensions of the numerator and the denominator.
Step 4: Analyze the numerator
The numerator is A+x3. Since x3 has dimensions:
(x3)=L3
This means that A must also have dimensions of L3 for the terms to be dimensionally consistent.
Step 5: Find the dimensions of A
Thus, we have:
(A)=L3
Now, calculating A2:
(A2)=(L3)2=L6
Step 6: Analyze the denominator
The denominator is Bx1/4+C. The term x1/4 has dimensions:
(x1/4)=L1/4
For dimensional consistency, the dimensions of Bx1/4 must equal the dimensions of x3 (which is L3). Thus:
(B)⋅(x1/4)=L3
This implies:
(B)⋅L1/4=L3⇒(B)=L3−1/4=L11/4
Step 7: Find the dimensional formula for A2B
Now we can find the dimensional formula for A2B:
(A2B)=(A2)⋅(B)=L6⋅L11/4=L6+11/4=L24/4+11/4=L35/4
Step 8: Consider the dimensions of kinetic energy in the denominator
The denominator also contains C, which must have the same dimensions as A for consistency. Thus, (C)=L3.
Step 9: Combine the dimensions to find A2B
Finally, we need to consider the dimensions of kinetic energy K in the denominator:
(Bx1/4) must have dimensions ML2T−2 as well.
Thus:
(B)=L3L1/4=L11/4
Step 10: Final dimensional formula for A2B
Now, we can combine everything:
(A2B)=L6⋅L11/4=L35/4
However, we need to consider the kinetic energy's mass dimension:
(A2B)=L35/4ML2T−2=M−1L27/4T2
Final Answer
Thus, the dimensional formula for A2B is:
(A2B)=M−1L27/4T2
The equation for kinetic energy is given as:
K=A+x3Bx1/4+C
Where:
- K is kinetic energy.
- A, B, and C are constants.
- x is the distance from the origin.
The dimensional formula for kinetic energy K is:
(K)=ML2T−2
According to the principle of homogeneity, all terms in the equation must have the same dimensions. Therefore, we can equate the dimensions of the numerator and the denominator.
The numerator is A+x3. Since x3 has dimensions:
(x3)=L3
This means that A must also have dimensions of L3 for the terms to be dimensionally consistent.
Thus, we have:
(A)=L3
Now, calculating A2:
(A2)=(L3)2=L6
The denominator is Bx1/4+C. The term x1/4 has dimensions:
(x1/4)=L1/4
For dimensional consistency, the dimensions of Bx1/4 must equal the dimensions of x3 (which is L3). Thus:
(B)⋅(x1/4)=L3
This implies:
(B)⋅L1/4=L3⇒(B)=L3−1/4=L11/4
Now we can find the dimensional formula for A2B:
(A2B)=(A2)⋅(B)=L6⋅L11/4=L6+11/4=L24/4+11/4=L35/4
The denominator also contains C, which must have the same dimensions as A for consistency. Thus, (C)=L3.
Finally, we need to consider the dimensions of kinetic energy K in the denominator:
(Bx1/4) must have dimensions ML2T−2 as well.
Thus:
(B)=L3L1/4=L11/4
Now, we can combine everything:
(A2B)=L6⋅L11/4=L35/4
However, we need to consider the kinetic energy's mass dimension:
(A2B)=L35/4ML2T−2=M−1L27/4T2
Thus, the dimensional formula for A2B is:
(A2B)=M−1L27/4T2
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )
8 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE
20 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - ASSIGNMENT SECTION H (Multiple True-False)
6 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (SECTION -D) (Assertion - Reason Type Questions)
10 Videos
- WAVE OPTICSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))
1 Videos
Similar Questions
For a particle moving along the x-axis, mark the correct statement(s).
View Solutionx-t equation of a particle moving along x-axis is given as
x=A+A(1−cosωt)
View SolutionThe kinetic energy K of a particle moving along x - axis varies with its position (x) as shown in figure
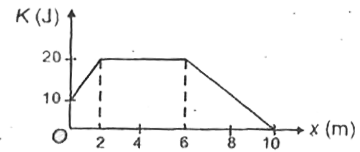
The magnitude of force acting on particle at x = 9 mis
View SolutionVelocity time graphs of particles A and B moving along x-axis are shown here. Both A and B start from origin:

View SolutionFor a particle moving along x−axis, speed must be increasing for the following graph :
View SolutionPosition of a particle moving along x-axis is given by x=2+8t−4t2. The distance travelled by the particle from t=0 to t=2 is:-
View SolutionThe potential energy of a particle varies with distance x from a fixed origin as U=A√xx2+B, where AandB are dimensional constants , then find the dimensional formula for AB.
View SolutionThe potential energy of a particle varies with distance x from a fixed origin as U=A√xx2+B, where AandB are dimensional constants , then find the dimensional formula for AB.
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionThe potential energy of a particle varies with distance x from a fixed origin as V=A√XX+B where A and B are constants . The dimension of AB are
View Solution
